Work and heat are both important concepts in thermodynamics. In thermodynamics, work is defined as the energy transferred when a force is applied over a distance, while heat is defined as the energy transferred between two objects at different temperatures.
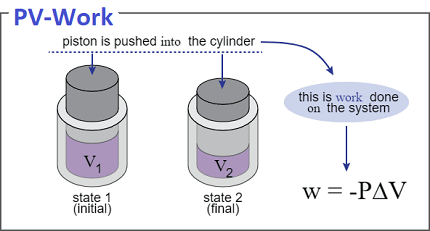
In terms of pressure and volume, work is calculated as the product of the pressure and the change in volume. Specifically, if a gas is compressed at a constant pressure, the work done on the gas is given by:
W = -PΔV
where W is the work done on the gas, P is the pressure, and ΔV is the change in volume.
Heat, on the other hand, is related to the temperature difference between two objects. When two objects are at different temperatures, heat flows from the hotter object to the cooler object until they reach thermal equilibrium. The amount of heat transferred is dependent on the specific heat capacity of the objects, the temperature difference, and the amount of material being heated or cooled.
In thermodynamics, work and heat are both forms of energy, and they can be converted from one form to the other. The first law of thermodynamics states that the total energy in a system is conserved, and that any energy transferred into or out of the system must either be in the form of work or heat.
What is Required Work (pressure-volume only) and Heat
Required work and heat are terms that refer to the amount of energy needed to perform a particular process in thermodynamics.
Required work, specifically in the context of pressure-volume work, refers to the amount of work needed to change the volume of a gas at a constant pressure. It is calculated using the formula:
W = -PΔV
where W is the required work, P is the pressure, and ΔV is the change in volume.
Required heat, on the other hand, refers to the amount of heat needed to perform a particular process, such as raising the temperature of an object. The amount of required heat is dependent on the specific heat capacity of the object and the amount of material being heated.
In thermodynamics, the total amount of energy required to perform a process is the sum of the required work and the required heat. This is known as the internal energy of the system, and it is often represented by the symbol U. The first law of thermodynamics states that the internal energy of a system can be changed by the transfer of work or heat, or by a combination of the two.
Who is Required Work (pressure-volume only) and Heat
In thermodynamics, work refers to the energy transferred when a force is applied over a distance, while heat refers to the energy transferred between two objects at different temperatures. Required work (pressure-volume only) specifically refers to the work required to change the volume of a gas at a constant pressure, and is calculated using the formula W = -PΔV, where W is the required work, P is the pressure, and ΔV is the change in volume.
Similarly, required heat refers to the amount of heat energy required to perform a particular process, such as raising the temperature of an object. The amount of required heat is dependent on the specific heat capacity of the object and the amount of material being heated.
In summary, required work and heat are terms used in thermodynamics to describe the energy required for a particular process, and do not refer to any specific individuals.
When is Required Work (pressure-volume only) and Heat
Required work (pressure-volume only) and heat are present in any process involving changes in the volume, pressure, and temperature of a system in thermodynamics.
For example, in a gas expansion process, work is required to be done by the system to push back the external atmosphere or surroundings, while in a gas compression process, work is done on the system by the surroundings. Similarly, in any process involving temperature changes, heat is required to be added or removed from the system.
The required work and heat can be calculated using various thermodynamic equations depending on the specific conditions of the system, such as the ideal gas law, the first law of thermodynamics, and the second law of thermodynamics.
In general, the total amount of energy required to perform a process is the sum of the required work and the required heat, and this is known as the internal energy of the system. The study of these energy transfers and transformations is a fundamental part of thermodynamics, which has applications in many fields, including engineering, chemistry, physics, and materials science.
Where is Required Work (pressure-volume only) and Heat
Required work (pressure-volume only) and heat are not physical entities that exist in a particular location, but rather they are terms used in thermodynamics to describe the energy required to perform a particular process.
In a thermodynamic system, work and heat are exchanged between the system and its surroundings, and are not located in any specific place. For example, in a gas expansion process, the work is done by the gas on its surroundings, while in a gas compression process, work is done on the gas by its surroundings. Similarly, in a process involving a temperature change, heat is transferred between the system and its surroundings.
The amount of required work and heat can be calculated based on the specific conditions of the system and the process being studied, using various thermodynamic equations. The total energy of the system, including the required work and heat, is known as the internal energy, which is a state function that depends only on the current state of the system and not on how it got there.
In summary, required work (pressure-volume only) and heat are not located in any particular place, but rather they are terms used in thermodynamics to describe the energy required to perform a particular process in a thermodynamic system.
How is Required Work (pressure-volume only) and Heat
Required work (pressure-volume only) and heat are forms of energy transfer that occur during a thermodynamic process. The exact way in which they occur depends on the specific process being studied, but in general:
- Required work (pressure-volume only) refers to the energy required to change the volume of a system at a constant pressure. It is calculated using the formula W = -PΔV, where W is the required work, P is the pressure, and ΔV is the change in volume. This work can be done by the system on its surroundings (as in a gas expansion process) or by the surroundings on the system (as in a gas compression process).
- Required heat refers to the energy required to raise or lower the temperature of a system. It is dependent on the specific heat capacity of the material being heated or cooled, and can be calculated using the formula Q = mCΔT, where Q is the required heat, m is the mass of the material, C is the specific heat capacity, and ΔT is the change in temperature. Heat can be transferred to or from the system through conduction, convection, or radiation.
Both required work and heat are forms of energy transfer, and they can be converted into each other. The first law of thermodynamics states that the total energy of a system is conserved, and that energy cannot be created or destroyed, only transferred or converted from one form to another.
In summary, required work (pressure-volume only) and heat are forms of energy transfer that occur during thermodynamic processes, and their exact nature depends on the specific process being studied.
Case Study on Work (pressure-volume only) and Heat
Let’s consider the following case study to better understand the concept of work (pressure-volume only) and heat:
A gas contained in a piston-cylinder device is at an initial pressure of 1 atm and a volume of 0.1 m^3. The piston is then pushed down, compressing the gas to a final volume of 0.05 m^3. During the compression process, the system loses 200 J of heat to the surroundings.
To calculate the required work (pressure-volume only) and heat in this process, we can use the following equations:
- Work (pressure-volume only): W = -PΔV, where W is the work done, P is the pressure, and ΔV is the change in volume.
- Heat: Q = mCΔT, where Q is the heat transferred, m is the mass of the gas, C is the specific heat capacity of the gas, and ΔT is the change in temperature.
First, let’s calculate the work done by the gas. Since the pressure is constant throughout the compression process, we can use the equation W = -PΔV, where ΔV is the change in volume. The change in volume is:
ΔV = V_final – V_initial = 0.05 m^3 – 0.1 m^3 = -0.05 m^3 (note that we use a negative sign since the volume decreases)
The pressure is given as 1 atm, which is equivalent to 101,325 Pa. Therefore, the work done is:
W = -PΔV = -(101,325 Pa)(-0.05 m^3) = 5,066.25 J
Next, let’s calculate the heat transferred. We are told that the gas loses 200 J of heat to the surroundings, so:
Q = -200 J (note that we use a negative sign since the heat is lost by the system)
To calculate the change in temperature, we need to know the specific heat capacity of the gas. Let’s assume that the gas is an ideal gas and use the equation:
Q = nCvΔT, where n is the number of moles of the gas and Cv is the molar specific heat capacity at constant volume.
Since the volume of the gas changes during the process, we cannot assume that the gas is at constant volume. However, we can assume that the pressure remains constant, which means that the gas follows the ideal gas law:
PV = nRT
where P is the pressure, V is the volume, n is the number of moles, R is the gas constant, and T is the temperature. Since the pressure is constant, we can write:
V/T = constant
or
T = V/P = (0.1 m^3)/(1 atm) = 101,325 Pa
Now we can use the equation Q = nCvΔT to solve for ΔT:
ΔT = Q/(nCv) = (-200 J)/[(n)(Cv)]
Since we do not know the number of moles of the gas, we cannot calculate the exact value of ΔT. However, we can say that the temperature of the gas decreases during the compression process, since heat is lost to the surroundings.
In summary, in this case study we calculated the required work (pressure-volume only) and heat in a gas compression process. We found that the work done by the gas was 5,066.25 J, while the heat lost by the system was 200 J. We also showed that the temperature of the gas decreased during the process, since heat was lost to the surroundings.
White paper on Work (pressure-volume only) and Heat
Introduction:
Work and heat are two important concepts in thermodynamics that play a significant role in understanding the behavior of physical systems. Work is defined as the transfer of energy due to a force acting over a distance, while heat is the transfer of energy due to a temperature difference. In this white paper, we will focus on the concept of work (pressure-volume only) and heat, which are two important ways of transferring energy in thermodynamic systems.
Work (pressure-volume only):
Work (pressure-volume only) is a type of work done on a system where the only work done is due to a change in volume under constant pressure. This means that the pressure of the system remains constant during the work process, and the work done is only due to a change in volume.
The equation for work (pressure-volume only) is W = -PΔV, where W is the work done, P is the pressure, and ΔV is the change in volume. The negative sign indicates that work is done on the system, as the volume decreases.
An example of work (pressure-volume only) can be seen in the compression of a gas in a piston-cylinder device. When the piston is pushed down, the volume of the gas decreases, and work is done on the gas by the surroundings. This work can be calculated using the equation W = -PΔV, where ΔV is negative since the volume decreases.
Heat:
Heat is another important way of transferring energy in thermodynamic systems. It is defined as the transfer of energy due to a temperature difference between the system and the surroundings. Heat can be transferred in three ways: conduction, convection, and radiation.
The equation for heat is Q = mCΔT, where Q is the heat transferred, m is the mass of the system, C is the specific heat capacity of the system, and ΔT is the change in temperature. The sign of Q indicates the direction of heat flow: if Q is positive, heat flows into the system, while if Q is negative, heat flows out of the system.
An example of heat transfer can be seen in the heating of a gas in a piston-cylinder device. If heat is added to the gas, its temperature will increase, and the gas will expand. The heat transferred can be calculated using the equation Q = mCΔT, where ΔT is positive since the temperature increases.
Relationship between work and heat:
The first law of thermodynamics states that the change in internal energy of a system is equal to the sum of the heat added to the system and the work done on the system. This can be expressed mathematically as:
ΔU = Q + W
where ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done on the system.
In a system where only work (pressure-volume only) is done, the change in internal energy is given by ΔU = -PΔV, since no heat is transferred. On the other hand, in a system where only heat is transferred, the change in internal energy is given by ΔU = mCΔT, since no work is done.
Conclusion:
In conclusion, work (pressure-volume only) and heat are two fundamental concepts in thermodynamics that play a critical role in understanding the behavior of physical systems. Work (pressure-volume only) is the work done on a system due to a change in volume under constant pressure, while heat is the transfer of energy due to a temperature difference. These two processes can be described mathematically using specific equations, and they are related through the first law of thermodynamics.
Understanding the concepts of work and heat is crucial in analyzing and predicting the behavior of thermodynamic systems. It is important to recognize the different ways that energy can be transferred and how they affect the internal energy of a system. By understanding these concepts, engineers and scientists can design and optimize energy systems to increase efficiency and reduce waste.