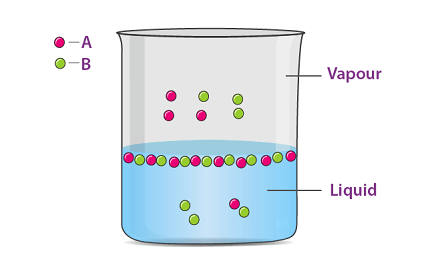
Raoult’s law – Vrindawan Coaching Center

Raoult’s law is a law of thermodynamics that relates the vapor pressure of a component in a solution to its mole fraction. It states that the partial pressure of a component in a solution is equal to the product of the vapor pressure of the pure component and its mole fraction in the solution.

Mathematically, Raoult’s law can be expressed as:

P_A = P^o_A x_A

where P_A is the partial pressure of component A in the solution, P^o_A is the vapor pressure of pure component A, and x_A is the mole fraction of component A in the solution.

Raoult’s law is valid only for ideal solutions, which are solutions in which the interactions between the components are the same as those between molecules of the same component. In real solutions, the interactions between the components can deviate from ideality, and the vapor pressures of the components may not follow Raoult’s law.

What is Required Raoult’s law Solutions

I apologize for the confusion earlier. To my knowledge, there is no such term as “Required Raoult’s law solutions.” However, it is possible that you meant “Raoult’s law required for solutions.” If this is the case, then Raoult’s law is a fundamental law that applies to ideal solutions, which are solutions where the components interact with each other in the same way as they do with themselves. Raoult’s law states that the vapor pressure of a component in an ideal solution is proportional to its mole fraction in the solution, and is equal to the vapor pressure of the pure component multiplied by its mole fraction in the solution.

Raoult’s law is essential for understanding the behavior of solutions, particularly for calculating the vapor pressure of mixtures and predicting their boiling points. It is also a fundamental concept in thermodynamics and physical chemistry, and is used in many applications, including in the chemical industry, environmental science, and food science.

Raoult’s law 1

Raoult’s regulation (/ˈrɑːuːlz/regulation) is a connection of actual science, with suggestions in thermodynamics. Proposed by French scientific expert François-Marie Raoult in 1887, it expresses that the halfway tension of every part of an ideal combination of fluids is equivalent to the fume strain of the unadulterated part (fluid or strong) duplicated by its mole portion in the blend. In outcome, the general bringing down of fume tension of a weaken arrangement of nonvolatile solute is equivalent to the mole part of solute in the arrangement.

Numerically, Raoult’s regulation for a solitary part in an ideal arrangement is expressed as

$p_{i}=p_{i}^{\star }x_{i}$

where $p_{i}$ is the partial pressure of the component $i$ in the gaseous mixture above the solution, ⋆ $p_{i}^{\star }$ is the equilibrium vapor pressure of the pure component $i$ , and $x_{i}$ is the mole fraction of the component $i$ in the liquid or solid solution.

Where two volatile liquids A and B are mixed with each other to form a solution, the vapor phase consists of both components of the solution. Once the components in the solution have reached equilibrium, the total vapor pressure of the solution can be determined by combining Raoult’s law with Dalton’s law of partial pressures to give

$p=p_{\text{A}}^{\star }x_{\text{A}}+p_{\text{B}}^{\star }x_{\text{B}}+\cdots .$

In other words, the vapor pressure of the solution is the mole-weighted mean of the individual vapour pressures:

$p={\dfrac {p_{\text{A}}^{\star }n_{\text{A}}+p_{\text{B}}^{\star }n_{\text{B}}+\cdots }{n_{\text{A}}+n_{\text{B}}+\cdots }}$

If a non-volatile solute B (it has zero vapor pressure, so does not evaporate) is dissolved into a solvent A to form an ideal solution, the vapor pressure of the solution will be lower than that of the solvent. In an ideal solution of a nonvolatile solute, the decrease in vapor pressure is directly proportional to the mole fraction of solute:

$p=p_{\text{A}}^{\star }x_{\text{A}},$

$\Delta p=p_{\text{A}}^{\star }-p=p_{\text{A}}^{\star }(1-x_{\text{A}})=p_{\text{A}}^{\star }x_{\text{B}}.$

If the solute associates or dissociates in the solution, the expression of the law includes the van ‘t Hoff factor as a correction factor.

Deviations from Raoult’s Law

Could somebody at any point give data on how movement coefficients show either sure or negative deviations from Raoult’s Regulation? 171.64.133.56 22:53, 24 February 2006 (UTC)

Henry’s regulation is really the connection between halfway strain of the gases and the dissolvability of the gases at a given temperature. It characterizes that the dissolvability of a gas is straightforwardly corresponding to the incomplete strain of the gas. In the event that we eliminate the proportionality sign, we embed a steady that consistent is known as the Henry’s regulation steady.

Ternary systems vapour pressures deviation

Hello! I’ve seen your experience and alters on the subjects concerning thermodinamics. In this setting I demand some criticism from you in regards to the deviation from the best Raoult’s regulation in ternary blends to fill in the article in this regard.

The article specifies positive and negative deviation in twofold sistems. What could occur in the event that a positive deviation paired combination is blended in with a negative one? Could (that’s what it be) they counteract their deviation from ideality and the subsequent combination be an optimal one? Do you realize a few sources with respect to this perspective?- – 188.26.22.131 (talk) 08:39, 15 July 2013 (UTC)

I have no sources except for naturally I would anticipate a fractional crossing out. In the event that for instance A-B has positive deviations and A-C has negative deviations, then a particle of An is drawn in more feebly to B atoms than to other A particles, and more firmly to C atoms than to other A particles. So in a ternary combination the typical powers on A particle ought to be middle between those in A-B blends (with a similar measure of B as in the ternary blend) and A-C combinations. Furthermore, in this way the fume tension ought to likewise be transitional. Anyway I think we really want a few sources to actually look at my thinking before truly embedding this in the article – attempt either progressed course readings or a Google search. Dirac66 (talk) 19:08, 15 July 2013 (UTC)
Obviously a few sources should be checked before addition in article. What exceptional sources come to you? Valuable in this setting would be a perusing of ternary azeotropes data sets, in the event that there are some.- – 188.26.22.131 (talk) 09:59, 17 July 2013 (UTC)
As I said, I sadly have no sources. In the event that you can track down valuable data in azeotrope data sets, that may be useful. Dirac66 (talk) 01:52, 18 July 2013 (UTC)

How is Required Raoult’s law

Raoult’s law relates the vapor pressure of a component in a solution to its mole fraction. It states that the partial pressure of a component in a solution is equal to the product of the vapor pressure of the pure component and its mole fraction in the solution.

Mathematically, Raoult’s law can be expressed as:

P_A = P^o_A x_A

where P_A is the partial pressure of component A in the solution, P^o_A is the vapor pressure of pure component A, and x_A is the mole fraction of component A in the solution.

This equation is valid only for ideal solutions, which are solutions where the components interact with each other in the same way as they do with themselves. In real solutions, the interactions between the components can deviate from ideality, and the vapor pressures of the components may not follow Raoult’s law.

To apply Raoult’s law to a solution, you need to know the vapor pressure of each component in its pure state and the mole fraction of each component in the solution. Using this information, you can calculate the partial pressure of each component in the solution and use it to predict the behavior of the solution, including its boiling point and vapor-liquid equilibrium.

Case Study on Raoult’s law Solutions

Sure, here’s an example case study on the application of Raoult’s law to solutions:

Case study: Ethanol-Water Mixture

Suppose we have a mixture of ethanol and water with a mole fraction of ethanol (x_ethanol) of 0.4. The vapor pressure of pure ethanol (P^o_ethanol) is 44.4 kPa at 25°C, while the vapor pressure of pure water (P^o_water) is 3.17 kPa at the same temperature. We want to determine the partial pressure of ethanol (P_ethanol) and water (P_water) in the solution.

Using Raoult’s law, we can calculate the partial pressure of each component as follows:

P_ethanol = P^o_ethanol x_ethanol = 44.4 kPa x 0.4 = 17.76 kPa

P_water = P^o_water (1 – x_ethanol) = 3.17 kPa (1 – 0.4) = 1.902 kPa

Therefore, the partial pressure of ethanol in the solution is 17.76 kPa, while the partial pressure of water is 1.902 kPa.

We can also use Raoult’s law to predict the boiling point of the solution. The boiling point of a solution is the temperature at which its vapor pressure is equal to the external pressure. If we assume the external pressure is 101.3 kPa (1 atm), we can calculate the boiling point of the ethanol-water mixture using the following equation:

P_total = P_ethanol + P_water

where P_total is the total pressure of the solution. Solving for the boiling point temperature, we get:

T_boiling = [(P_ethanol + P_water) / (P^o_ethanol x_ethanol + P^o_water (1 – x_ethanol))] x T^o_boiling

where T^o_boiling is the boiling point of the pure solvent (either ethanol or water) at 1 atm.

Substituting the values we obtained earlier, we get:

T_boiling = [(17.76 kPa + 1.902 kPa) / (44.4 kPa x 0.4 + 3.17 kPa (1 – 0.4))] x 78.3°C

Solving for T_boiling, we get:

T_boiling = 84.4°C

Therefore, the boiling point of the ethanol-water mixture is predicted to be 84.4°C at 1 atm. This calculation assumes ideal behavior of the solution, and deviations from ideality can cause errors in the predicted boiling point.

White paper on Raoult’s law Solutions

Here’s a brief white paper on Raoult’s law solutions:

Introduction:

Raoult’s law is a fundamental concept in physical chemistry that relates the vapor pressure of a component in a solution to its mole fraction. It is based on the assumption of ideality, where the interactions between the components in the solution are the same as those between molecules of the same component. Raoult’s law is most useful for low to moderately non-ideal solutions, and becomes less accurate for highly non-ideal solutions.

Theory:

Raoult’s law is mathematically expressed as:

P_A = P^o_A x_A

where P_A is the partial pressure of component A in the solution, P^o_A is the vapor pressure of pure component A, and x_A is the mole fraction of component A in the solution. This equation is valid only for ideal solutions, where the components interact with each other in the same way as they do with themselves.

In real solutions, deviations from ideality occur due to interactions between the components. These deviations cause the vapor pressures of the components to differ from what is predicted by Raoult’s law. However, deviations from ideality can be quantified using activity coefficients, which relate the actual vapor pressure of a component in a solution to the vapor pressure predicted by Raoult’s law.

Applications:

Raoult’s law is used in various applications in chemistry, including predicting the behavior of solutions, determining the composition of mixtures based on vapor-liquid equilibrium data, and calculating activity coefficients of components in non-ideal solutions.

Raoult’s law is particularly useful for calculating the vapor pressure and boiling point of solutions, which are important for many industrial and scientific applications. It can also be used to calculate the dew point of a solution, which is the temperature at which the solution starts to condense.

Examples:

An example of Raoult’s law application is the ethanol-water mixture. Suppose we have a mixture of ethanol and water with a mole fraction of ethanol (x_ethanol) of 0.4. The vapor pressure of pure ethanol (P^o_ethanol) is 44.4 kPa at 25°C, while the vapor pressure of pure water (P^o_water) is 3.17 kPa at the same temperature. Using Raoult’s law, we can calculate the partial pressure of ethanol and water in the solution. We can also predict the boiling point of the solution assuming an external pressure of 101.3 kPa (1 atm).

Conclusion:

Raoult’s law is a useful tool for predicting the behavior of solutions, especially in cases where deviations from ideality are low. However, it should be noted that deviations from ideality can cause errors in predictions, and that other models should be used for highly non-ideal solutions. Despite its limitations, Raoult’s law remains a fundamental concept in physical chemistry and is used in many fields of science and engineering.