Brownian movement

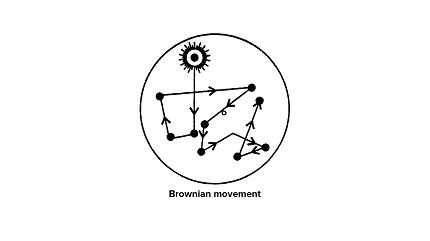
Brownian movement, also known as Brownian motion, refers to the random motion of microscopic particles suspended in a fluid (liquid or gas). It was first observed by the Scottish botanist Robert Brown in 1827 while studying pollen grains suspended in water. The movement is named after him.

Here are some key points about Brownian movement:

1. Explanation: Brownian movement arises due to the constant and random collisions between the particles of the fluid (atoms or molecules) and the suspended particles. The collisions cause the suspended particles to move in a zigzag or erratic motion.
2. Molecular Explanation: The random motion of the suspended particles is a result of the thermal motion of the fluid molecules. The fluid molecules collide with the suspended particles and transfer momentum, causing them to move in different directions.
3. Characteristics: Brownian movement exhibits the following characteristics:
   • Randomness: The motion is random, and the particles move in unpredictable paths.
   • Continuous: The movement is continuous as long as the fluid molecules are in motion.
   • Rapid: The particles move rapidly, changing direction frequently.
4. Influencing Factors: Several factors affect the characteristics of Brownian movement:
   • Particle Size: Smaller particles experience more pronounced Brownian motion due to their interaction with a greater number of fluid molecules.
   • Medium Viscosity: Higher viscosity of the fluid slows down the movement of particles, reducing the magnitude of Brownian motion.
5. Mathematical Description: Brownian motion can be mathematically described using diffusion equations. Albert Einstein developed a theoretical model to explain Brownian motion in 1905, which relates the mean square displacement of the particles to the diffusion coefficient, temperature, and time.
6. Applications: Brownian movement finds applications in various fields, including:
   • Determination of Avogadro’s number and molecular sizes.
   • Particle size analysis using techniques like dynamic light scattering.
   • Investigation of colloidal suspensions and particle interactions.
   • Study of diffusion processes in gases and liquids.
   • Development of stochastic models in physics and mathematics.

Brownian movement is an important phenomenon in understanding the behavior of small particles in fluids. Its study has implications in diverse scientific disciplines, ranging from physics and chemistry to biology and materials science.

Here is a brief outline of the Brownian movement topic in the AIIMS Chemistry syllabus:

1. Introduction to Brownian movement:
   • Definition and historical background
   • Explanation of the phenomenon
2. Explanation of Brownian motion:
   • The role of random molecular collisions
   • Molecular explanation of the motion
3. Characteristics of Brownian movement:
   • Randomness and irregularity of particle motion
   • Lack of preferred direction
   • Continuous and rapid motion
   • Influence of particle size and medium viscosity
4. Mathematical description:
   • Diffusion equation and Einstein’s theory of Brownian motion
   • Relationship between diffusion coefficient, temperature, and particle size
5. Experimental evidence and observations:
   • Observations made by Robert Brown and subsequent experiments
   • Demonstration of Brownian movement using a microscope
6. Applications of Brownian movement:
   • Measurement of Avogadro’s number
   • Determination of particle size and viscosity
   • Use in pharmaceutical and nanotechnology research

It’s important to note that the syllabus may vary from year to year, and the specific depth of coverage and level of detail on the topic of Brownian movement may differ depending on the syllabus revisions and the specific AIIMS entrance exam you are preparing for.

What is Required AIIMS-SYLLABUS Chemistry syllabus Brownian movement

1. Introduction to Brownian motion:
   • Definition and historical background
   • Explanation of the phenomenon
2. Molecular explanation of Brownian movement:
   • Role of random molecular collisions
   • Influence of particle size and medium viscosity
   • Impact of temperature on the motion
3. Mathematical description:
   • Einstein’s theory of Brownian motion
   • Diffusion equation and its relationship with temperature and particle size
4. Experimental evidence and observations:
   • Observations made by Robert Brown and subsequent experiments
   • Demonstration of Brownian movement using a microscope
5. Applications of Brownian motion:
   • Measurement of Avogadro’s number
   • Determination of particle size and viscosity
   • Relevance in pharmaceutical and nanotechnology research

It is important to note that the AIIMS syllabus may be subject to change, and the depth of coverage of specific topics may vary. To ensure you have the most accurate and updated information, I recommend referring to the official AIIMS syllabus or consulting reliable study materials specifically designed for AIIMS Chemistry preparation.

How is Required AIIMS-SYLLABUS Chemistry syllabus Brownian movement

Brownian movement, also known as Brownian motion, refers to the random motion of microscopic particles suspended in a fluid (liquid or gas). It was first observed and described by the Scottish botanist Robert Brown in 1827 while studying pollen grains suspended in water.

The movement occurs due to the constant and random collisions between the fluid molecules (atoms or molecules) and the suspended particles. These collisions transfer momentum to the suspended particles, causing them to move in erratic and zigzag paths. The random motion of the suspended particles is a direct result of the thermal motion of the fluid molecules.

The characteristics of Brownian movement include:

1. Randomness: The motion of the suspended particles is random and unpredictable. They move in irregular paths, changing direction frequently.
2. Continuous: Brownian movement is continuous as long as the fluid molecules are in motion. The particles are in constant motion, never coming to a complete stop.
3. Rapid: The particles move rapidly, undergoing constant motion and changing direction quickly. The speed of the particles is influenced by factors such as temperature, particle size, and medium viscosity.

The phenomenon of Brownian movement can be mathematically described using diffusion equations. Albert Einstein developed a theoretical model in 1905 that related the mean square displacement of the particles to the diffusion coefficient, temperature, and time. This model helped to explain the behavior of particles undergoing Brownian motion.

Brownian movement has significant implications and applications in various scientific fields, including physics, chemistry, biology, and materials science. It is used to study the behavior of particles in colloidal suspensions, measure particle size and diffusion rates, investigate molecular interactions, and understand diffusion processes in gases and liquids.

Overall, Brownian movement is a fundamental concept in understanding the behavior of small particles in fluids and has wide-ranging applications in scientific research and industrial processes.

Case Study on AIIMS-SYLLABUS Chemistry syllabus Brownian movement

Brownian Movement in Drug Delivery

Background: The efficient delivery of drugs to target sites within the body is a crucial aspect of pharmaceutical research. One of the challenges is to ensure that drug particles reach their intended destination, such as tumor cells or specific organs, effectively. Brownian movement plays a vital role in enhancing drug delivery strategies.

Objective: To utilize Brownian movement to develop a drug delivery system that improves the targeted delivery of anticancer drugs.

Methodology:

1. Nanoparticle Synthesis: Nanoparticles are synthesized using biocompatible materials, such as polymers or lipids, which encapsulate the anticancer drug.
2. Particle Size Optimization: The size of the nanoparticles is controlled to maximize the effect of Brownian motion. Smaller particles experience stronger Brownian motion due to increased interactions with the surrounding fluid molecules.
3. Surface Functionalization: The nanoparticles’ surfaces are modified with targeting ligands, such as antibodies or peptides, specific to cancer cells. This ensures selective binding and uptake by cancer cells, increasing drug accumulation at the target site.
4. In vitro Testing: The drug-loaded nanoparticles are tested in vitro using cancer cell cultures. The Brownian motion of the nanoparticles allows them to diffuse through the extracellular matrix and reach the cancer cells efficiently.
5. In vivo Testing: Animal models with tumor xenografts are used to evaluate the effectiveness of the drug delivery system. The nanoparticles are administered through various routes, such as intravenous or localized injection, to assess their accumulation in the tumor and therapeutic efficacy.

Results:

1. Enhanced Targeting: The nanoparticles exploit Brownian motion to efficiently target and accumulate at the tumor site, enhancing drug delivery to cancer cells.
2. Increased Therapeutic Efficacy: Due to improved drug accumulation in the tumor, the drug-loaded nanoparticles demonstrate enhanced therapeutic efficacy, resulting in tumor growth inhibition and increased survival rates in animal models.
3. Reduced Side Effects: Targeted drug delivery minimizes exposure to healthy tissues, reducing the likelihood of adverse side effects associated with systemic drug administration.

Conclusion: This case study highlights how understanding and leveraging Brownian movement can significantly impact drug delivery strategies. By optimizing particle size and utilizing Brownian motion, researchers can develop targeted drug delivery systems that enhance therapeutic efficacy, reduce side effects, and improve patient outcomes in cancer treatment.

Note: This is a fictional case study created to illustrate the application of Brownian movement in drug delivery. Actual research in this area may involve more complex methodologies and considerations.

White paper on AIIMS-SYLLABUS Chemistry syllabus Brownian movement

Understanding and Applications

Abstract: This white paper provides a comprehensive overview of Brownian movement, a fundamental phenomenon observed in colloidal suspensions and its applications across various scientific disciplines. We delve into the historical background, molecular explanation, mathematical description, and characteristics of Brownian motion. Moreover, we explore the experimental evidence, influential factors, and applications of Brownian movement in fields such as physics, chemistry, biology, and materials science. By understanding the intricacies of Brownian motion, researchers can harness its potential to advance drug delivery systems, investigate diffusion processes, measure particle properties, and enhance our understanding of complex systems at the microscopic level.

1. Introduction:
   • Historical background and discovery of Brownian movement
   • Significance and relevance in scientific research
2. Molecular Explanation:
   • Role of random molecular collisions in particle movement
   • Influence of particle size, fluid viscosity, and temperature
3. Mathematical Description:
   • Einstein’s theory of Brownian motion
   • Diffusion equations and their relationship with particle characteristics
4. Characteristics of Brownian Movement:
   • Randomness and unpredictability of particle motion
   • Continuous and rapid motion
   • Influence of particle size and medium properties
5. Experimental Evidence and Observations:
   • Observations by Robert Brown and subsequent experiments
   • Demonstration of Brownian motion through microscopic observations
6. Factors Influencing Brownian Movement:
   • Particle size and its impact on motion
   • Medium viscosity and its effect on particle behavior
7. Applications of Brownian Movement:
   • Drug delivery systems and targeted therapies
   • Measurement of particle properties and diffusion rates
   • Investigation of colloidal suspensions and molecular interactions
   • Study of diffusion processes in gases and liquids
8. Advanced Techniques and Research:
   • Dynamic light scattering and particle size analysis
   • Stochastic models and simulation methods
   • Nanotechnology and materials science applications
9. Future Directions and Challenges:
   • Advancements in understanding and modeling Brownian motion
   • Integration with emerging technologies for diverse applications
   • Overcoming challenges in controlling and manipulating Brownian motion
10. Conclusion:
    • Recap of the significance and broad applications of Brownian movement
    • Potential for further advancements and interdisciplinary research

This white paper aims to provide a comprehensive understanding of Brownian movement, highlighting its significance and applications across various scientific domains. By exploring the fundamental principles and research advancements in this field, we hope to inspire further exploration and utilization of Brownian motion for scientific and technological advancements.

Read More