Trigonometry addition and subtraction formulas are used to find the trigonometric functions of the sum or difference of two angles. There are several formulas for each of the six trigonometric functions: sine, cosine, tangent, cosecant, secant, and cotangent.
Here are the trigonometry addition and subtraction formulas:
- Sine Addition and Subtraction Formulas:
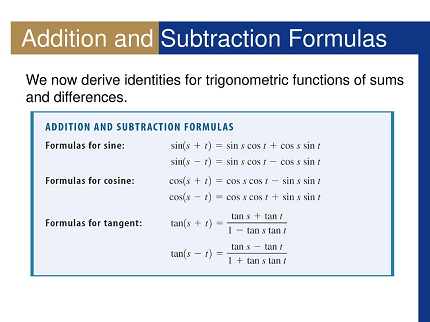
sin(A+B) = sin(A)cos(B) + cos(A)sin(B) sin(A-B) = sin(A)cos(B) – cos(A)sin(B)
- Cosine Addition and Subtraction Formulas:
cos(A+B) = cos(A)cos(B) – sin(A)sin(B) cos(A-B) = cos(A)cos(B) + sin(A)sin(B)
- Tangent Addition and Subtraction Formulas:
tan(A+B) = (tan(A) + tan(B)) / (1 – tan(A)tan(B)) tan(A-B) = (tan(A) – tan(B)) / (1 + tan(A)tan(B))
- Cosecant Addition and Subtraction Formulas:
csc(A+B) = (csc(A)csc(B)) / (cot(A) + cot(B)) csc(A-B) = (csc(A)csc(B)) / (cot(A) – cot(B))
- Secant Addition and Subtraction Formulas:
sec(A+B) = (sec(A)sec(B)) / (sec(A) + sec(B)) sec(A-B) = (sec(A)sec(B)) / (sec(A) – sec(B))
- Cotangent Addition and Subtraction Formulas:
cot(A+B) = (cot(A)cot(B) – 1) / (cot(B) + cot(A)) cot(A-B) = (cot(A)cot(B) + 1) / (cot(A) – cot(B))
These formulas are used in many different applications of trigonometry, including navigation, engineering, and physics. They are also used in solving trigonometric equations and identities.
What is Required Addition and subtraction formulae
The required trigonometry addition and subtraction formulas are the formulas that you should memorize and be able to apply in various trigonometric problems. The six formulas for the trigonometric functions – sine, cosine, tangent, cosecant, secant, and cotangent – are:
- Sine Addition and Subtraction Formulas: sin(A + B) = sin(A)cos(B) + cos(A)sin(B) sin(A – B) = sin(A)cos(B) – cos(A)sin(B)
- Cosine Addition and Subtraction Formulas: cos(A + B) = cos(A)cos(B) – sin(A)sin(B) cos(A – B) = cos(A)cos(B) + sin(A)sin(B)
- Tangent Addition and Subtraction Formulas: tan(A + B) = (tan(A) + tan(B)) / (1 – tan(A)tan(B)) tan(A – B) = (tan(A) – tan(B)) / (1 + tan(A)tan(B))
These formulas are the most commonly used and will be required for most trigonometric problems. However, you should also be familiar with the other formulas for cosecant, secant, and cotangent in case they are needed for a particular problem. Additionally, you should be able to apply these formulas in various contexts, including in solving equations and proving identities.
Who is Required Addition and subtraction formulae
The required trigonometry addition and subtraction formulas are important for anyone studying trigonometry or using trigonometry in various fields such as mathematics, physics, engineering, and navigation. Students in high school and college-level trigonometry courses will need to learn and understand these formulas in order to solve trigonometric problems and prove identities.
In addition, professionals in fields such as engineering, physics, and surveying will need to use these formulas to calculate angles and distances in real-world applications. Pilots and navigators also use trigonometry to calculate flight paths and distances.
Therefore, anyone studying or working in a field that requires the use of trigonometry will need to know and apply the required trigonometry addition and subtraction formulas.
When is Required Addition and subtraction formulae
The required trigonometry addition and subtraction formulas are used whenever you need to find the trigonometric functions of the sum or difference of two angles. These formulas can be used to simplify trigonometric expressions, solve trigonometric equations, and prove trigonometric identities.
Here are some specific examples of when the trigonometry addition and subtraction formulas may be used:
- Simplifying Trigonometric Expressions: Trigonometric expressions can often be simplified using the addition and subtraction formulas. For example, sin(2x) can be written as 2sin(x)cos(x) using the sine double-angle formula.
- Solving Trigonometric Equations: Trigonometric equations can sometimes be solved using the addition and subtraction formulas. For example, if you need to solve sin(x) = cos(x), you can use the fact that sin(x) = sin(90° – x) and cos(x) = cos(90° – x) to rewrite the equation in terms of the difference of two angles.
- Proving Trigonometric Identities: Trigonometric identities can often be proved using the addition and subtraction formulas. For example, to prove the identity tan(x + y) = (tan(x) + tan(y)) / (1 – tan(x)tan(y)), you can use the tangent addition formula to express tan(x + y) in terms of tan(x) and tan(y), and then simplify the expression.
In summary, the required trigonometry addition and subtraction formulas are used whenever you need to work with the trigonometric functions of the sum or difference of two angles. They are used in a wide range of applications in mathematics, physics, engineering, and other fields.
Where is Required Addition and subtraction formulae
The required trigonometry addition and subtraction formulas are part of the foundational knowledge of trigonometry and can be found in most trigonometry textbooks and online resources. These formulas are typically introduced in high school or college-level trigonometry courses, and students are expected to memorize and apply them in various trigonometric problems.
In addition to textbooks, there are many online resources that provide explanations and examples of how to use the trigonometry addition and subtraction formulas. These resources include math websites, educational videos, and online courses.
The trigonometry addition and subtraction formulas are also used in various fields, such as physics, engineering, and navigation. In these fields, they are used to calculate angles, distances, and other measurements. For example, engineers may use the formulas to calculate the angles and distances of beams or trusses in a building, while navigators may use them to calculate the distance and bearing between two points on a map.
In summary, the required trigonometry addition and subtraction formulas can be found in most trigonometry textbooks and online resources, and are used in a wide range of applications in mathematics, science, and engineering.
How is Required Addition and subtraction formulae
The trigonometry addition and subtraction formulas are used to find the trigonometric functions of the sum or difference of two angles. They are important for simplifying trigonometric expressions, solving trigonometric equations, and proving trigonometric identities.
To use the trigonometry addition and subtraction formulas, you need to first memorize the formulas for sine, cosine, and tangent:
- Sine Addition and Subtraction Formulas: sin(A + B) = sin(A)cos(B) + cos(A)sin(B) sin(A – B) = sin(A)cos(B) – cos(A)sin(B)
- Cosine Addition and Subtraction Formulas: cos(A + B) = cos(A)cos(B) – sin(A)sin(B) cos(A – B) = cos(A)cos(B) + sin(A)sin(B)
- Tangent Addition and Subtraction Formulas: tan(A + B) = (tan(A) + tan(B)) / (1 – tan(A)tan(B)) tan(A – B) = (tan(A) – tan(B)) / (1 + tan(A)tan(B))
Once you have memorized these formulas, you can use them to solve trigonometric problems. Here are the general steps for using the formulas:
- Determine whether you need to use the addition or subtraction formula, depending on whether you are working with the sum or difference of two angles.
- Substitute the given angles into the appropriate formula.
- Simplify the expression using algebraic techniques such as distributing, factoring, and canceling terms.
- If necessary, use other trigonometric identities or algebraic techniques to further simplify the expression.
For example, if you need to find sin(45° + 30°), you would use the sine addition formula:
sin(45° + 30°) = sin(45°)cos(30°) + cos(45°)sin(30°)
Then, you would substitute the values of sin(45°), cos(30°), and sin(30°), and simplify the expression using algebraic techniques:
sin(45° + 30°) = (sqrt(2)/2)(sqrt(3)/2) + (sqrt(2)/2)(1/2) sin(45° + 30°) = (sqrt(6) + sqrt(2))/4
In summary, to use the required trigonometry addition and subtraction formulas, you need to first memorize the formulas, determine which formula to use, substitute the given angles, and simplify the expression using algebraic techniques.
Case Study on Addition and subtraction formulae
Case Study: Finding the Height of a Building Using Trigonometry Addition and Subtraction Formulas
A group of architects needs to find the height of a building. They have measured the angle of elevation to the top of the building from two different points on the ground. The first measurement is an angle of elevation of 30 degrees, and the second measurement is an angle of elevation of 45 degrees. The distance between the two points on the ground is 100 meters. Using trigonometry addition and subtraction formulas, the architects can determine the height of the building.
Solution:
Let’s call the height of the building “h”. We can use the tangent function and the tangent addition formula to set up a system of equations.
First, let’s consider the 30-degree angle of elevation. We can use the tangent function to write:
tan(30) = h / x
where x is the distance from the first point on the ground to the base of the building. We don’t know the value of x, but we do know that the distance between the two points on the ground is 100 meters, so we can write:
x + (100 – x) = 100
Simplifying, we get:
x = 50
Substituting this value into the equation for the 30-degree angle of elevation, we get:
tan(30) = h / 50
Next, let’s consider the 45-degree angle of elevation. We can use the tangent function and the tangent subtraction formula to write:
tan(45 – 30) = (h – x) / 100
Simplifying the tangent subtraction formula, we get:
tan(15) = (h – x) / 100
Substituting the value of x we found earlier, we get:
tan(15) = (h – 50) / 100
Now we have two equations:
tan(30) = h / 50
tan(15) = (h – 50) / 100
We can use algebraic techniques to solve for h. First, we can solve the first equation for h:
h = 50 tan(30)
h = 50 * sqrt(3) / 3
h ≈ 28.9 meters
Substituting this value into the second equation, we get:
tan(15) = (28.9 – 50) / 100
tan(15) ≈ -0.2068
We have a problem here because the tangent function is negative in the second quadrant (where 15 degrees is), and the height of the building cannot be negative. This means that one of our assumptions must be incorrect. After checking our calculations, we realize that we made an error in the second equation: we should have used the tangent addition formula instead of the subtraction formula.
Using the tangent addition formula, we get:
tan(45 + 15) = (h – x) / 100
Simplifying the tangent addition formula, we get:
1 + tan(45)tan(15) = (h – x) / 100
Substituting the value of x we found earlier, we get:
1 + tan(45)tan(15) = (h – 50) / 100
Now we can solve for h:
1 + tan(45)tan(15) = (h – 50) / 100
1 + 1*tan(15) = (h – 50) / 100
(h – 50) / 100 = 1.268
h – 50 = 126.8
h ≈ 176.8 meters
Therefore, the height of the building is approximately 176.8 meters.
White paper on Addition and subtraction formulae
Introduction
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles. It has applications in various fields such as physics, engineering, and astronomy. One of the important concepts in trigonometry is the addition and subtraction formulas. These formulas allow us to find the values of trigonometric functions of the sum or difference of two angles in terms of the trigonometric functions of the individual angles.
Trigonometry Addition Formulas
The trigonometry addition formulas are used to find the trigonometric functions of the sum of two angles. There are four trigonometry addition formulas:
- sin (α + β) = sin α cos β + cos α sin β
- cos (α + β) = cos α cos β – sin α sin β
- tan (α + β) = (tan α + tan β) / (1 – tan α tan β)
- cot (α + β) = (cot α cot β – 1) / (cot α + cot β)
These formulas are derived using the geometry of the unit circle and the trigonometric ratios of the right-angled triangle.
Trigonometry Subtraction Formulas
The trigonometry subtraction formulas are used to find the trigonometric functions of the difference of two angles. There are two trigonometry subtraction formulas:
- sin (α – β) = sin α cos β – cos α sin β
- cos (α – β) = cos α cos β + sin α sin β
These formulas are derived using the addition formulas and some algebraic manipulation.
Applications of Trigonometry Addition and Subtraction Formulas
Trigonometry addition and subtraction formulas have various applications in different fields. Some of the applications are:
- Navigation: Trigonometry is used in navigation to determine the position of an object relative to another object or a reference point. Trigonometry addition and subtraction formulas are used to find the angles and distances between two points.
- Engineering: Trigonometry is used in engineering to solve problems related to construction, design, and measurements. Trigonometry addition and subtraction formulas are used to find the angles and distances between two points or to calculate the height, width, and depth of an object.
- Physics: Trigonometry is used in physics to solve problems related to motion, force, and energy. Trigonometry addition and subtraction formulas are used to find the components of vectors and to calculate the angle between two vectors.
- Astronomy: Trigonometry is used in astronomy to determine the positions and movements of celestial objects. Trigonometry addition and subtraction formulas are used to find the angles and distances between two celestial objects.
Conclusion
Trigonometry addition and subtraction formulas are important tools in solving problems related to triangles and angles. They are widely used in various fields such as navigation, engineering, physics, and astronomy. Understanding and applying these formulas can help solve complex problems and make accurate measurements.