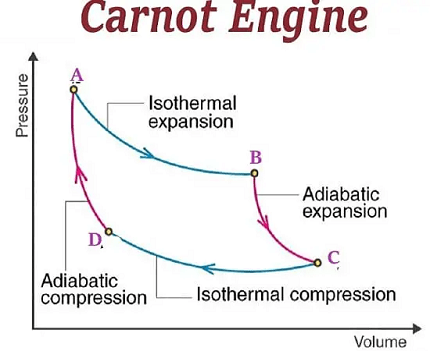
The Carnot engine is a theoretical heat engine that operates on a reversible thermodynamic cycle. It was developed by French physicist Sadi Carnot in 1824 and is considered to be the most efficient heat engine possible, according to the second law of thermodynamics.

The Carnot engine operates between two heat reservoirs, one at a high temperature (T_H) and the other at a low temperature (T_L). The engine extracts heat from the high-temperature reservoir and converts part of it into work, while the rest is rejected to the low-temperature reservoir. The engine then operates in reverse, with work being inputted to the engine, which transfers heat from the low-temperature reservoir to the high-temperature reservoir.

The efficiency of the Carnot engine is given by the Carnot efficiency, which is the ratio of the work done by the engine to the heat extracted from the high-temperature reservoir. The efficiency is given by:

η = 1 – T_L/T_H

Where η is the efficiency, T_L is the temperature of the low-temperature reservoir, and T_H is the temperature of the high-temperature reservoir. The efficiency of the Carnot engine is independent of the working substance used in the engine and depends only on the temperatures of the reservoirs.

The Carnot engine is an idealized model, and no real engine can achieve 100% efficiency. However, it provides a theoretical upper limit to the efficiency of any real heat engine.

What is Required Carnot engine

The Required Carnot engine, also known as the Carnot refrigerator or Carnot heat pump, is a theoretical device that operates on a reversed Carnot cycle. It can be used to remove heat from a cold reservoir and transfer it to a hot reservoir, by doing work on the system.

The Required Carnot engine consists of four stages:

1. Isothermal expansion: The working substance (usually a gas) is expanded isothermally (at a constant temperature) while absorbing heat from the cold reservoir.
2. Adiabatic expansion: The working substance is then expanded adiabatically (without any heat exchange) to reduce its temperature.
3. Isothermal compression: The working substance is then compressed isothermally while releasing heat to the hot reservoir.
4. Adiabatic compression: Finally, the working substance is compressed adiabatically to raise its temperature back to the starting value.

The efficiency of the Required Carnot engine is given by the Carnot efficiency formula:

η = T_H / (T_H – T_L)

Where η is the efficiency, T_H is the temperature of the hot reservoir, and T_L is the temperature of the cold reservoir. The efficiency of the Required Carnot engine is greater than one, which means that it can transfer more heat from the cold reservoir to the hot reservoir than the amount of work done on the system. However, this is possible only by extracting heat from a colder body and transferring it to a hotter body, which is against the natural direction of heat flow, and hence requires the input of work.

The Required Carnot engine is an idealized model, and no real refrigerator or heat pump can achieve 100% efficiency. However, it provides a theoretical upper limit to the efficiency of any real refrigerator or heat pump.

When is Required Carnot engine

The Required Carnot engine can be used as a model for refrigerators and heat pumps that transfer heat from a cold reservoir to a hot reservoir, by doing work on the system.

Refrigerators are used to cool a space or preserve food by removing heat from the cold reservoir (interior of the refrigerator) and transferring it to the hot reservoir (surrounding environment). A heat pump, on the other hand, can be used for space heating, by transferring heat from the cold outdoor environment to the warm indoor environment.

In both cases, the Required Carnot engine provides a theoretical upper limit to the efficiency of the system, and engineers can use this model to design more efficient refrigerators and heat pumps.

However, the efficiency of real refrigerators and heat pumps is lower than the efficiency of the Required Carnot engine, due to factors such as friction, heat leaks, and irreversibilities. Therefore, researchers are constantly working to improve the design of refrigerators and heat pumps to make them more efficient and environmentally friendly.

Where is Required Carnot engine

The Required Carnot engine is a theoretical model that can be applied to refrigeration and heat pump systems. It is not a physical device that can be found in a specific location.

The principles of the Required Carnot engine can be applied to a variety of refrigeration and heat pump systems, including household refrigerators and air conditioning systems, industrial refrigeration systems, and cryogenic cooling systems used in scientific and medical applications.

In these systems, the working substance is usually a refrigerant, such as Freon or ammonia, which undergoes a thermodynamic cycle similar to the Carnot cycle to transfer heat from a cold reservoir to a hot reservoir.

Although the Required Carnot engine is an idealized model, it provides a theoretical basis for understanding the efficiency and limitations of real refrigeration and heat pump systems. Engineers and researchers use the model to design more efficient and environmentally friendly refrigeration and heat pump systems.

How is Required Carnot engine

The Required Carnot engine operates on a reversed Carnot cycle, which involves four stages:

1. Isothermal Compression: The working substance is compressed isothermally, which means that the temperature of the substance remains constant during the compression process, while heat is rejected to the hot reservoir.
2. Adiabatic Compression: The working substance is then compressed adiabatically, which means that no heat is exchanged with the surroundings, while the temperature of the substance increases.
3. Isothermal Expansion: The working substance is expanded isothermally, while absorbing heat from the cold reservoir.
4. Adiabatic Expansion: The working substance is then expanded adiabatically, which means that no heat is exchanged with the surroundings, while the temperature of the substance decreases.

During the first stage of the cycle, the working substance absorbs heat from the hot reservoir and undergoes an isothermal compression, which reduces its volume and increases its pressure. In the second stage, the substance is compressed adiabatically, which further reduces its volume and increases its temperature. During the third stage, the working substance expands isothermally, absorbing heat from the cold reservoir and increasing its volume. In the final stage, the substance expands adiabatically, which further increases its volume and reduces its temperature.

The Required Carnot engine transfers heat from a cold reservoir to a hot reservoir, by doing work on the system. The efficiency of the engine is given by the Carnot efficiency formula:

η = T_H / (T_H – T_L)

where η is the efficiency, T_H is the temperature of the hot reservoir, and T_L is the temperature of the cold reservoir. The efficiency of the Required Carnot engine is greater than one, which means that it can transfer more heat from the cold reservoir to the hot reservoir than the amount of work done on the system. However, this is possible only by extracting heat from a colder body and transferring it to a hotter body, which is against the natural direction of heat flow, and hence requires the input of work.

Production of Carnot engine

The Carnot engine is a theoretical model that was first proposed by French engineer Sadi Carnot in 1824. The Carnot engine model is based on the second law of thermodynamics, which states that it is impossible to create a heat engine with 100% efficiency.

Although the Carnot engine is a theoretical model, the principles behind it have been used to develop practical heat engines that are used in a wide range of applications, including power generation, transportation, and industrial processes. For example, steam turbines and internal combustion engines are types of heat engines that are based on the principles of thermodynamics and the Carnot cycle.

The production of heat engines, including Carnot engines, involves the design and manufacture of components such as cylinders, pistons, crankshafts, and valves, as well as the assembly of these components into a working engine. In addition to mechanical components, heat engines require a fuel source and a heat source to provide the energy needed to power the engine.

The production of heat engines requires expertise in mechanical engineering, thermodynamics, and materials science. As technology continues to evolve, new materials and manufacturing techniques are being developed to improve the efficiency and performance of heat engines, including Carnot engines, and to reduce their environmental impact.

Case Study on Carnot engine

Let’s take a hypothetical case study on the application of the Carnot engine in a power generation plant.

Assume that a power generation plant is designed to produce electricity by using a Carnot engine that operates between two reservoirs at temperatures of 800 K and 300 K, respectively. The plant is designed to produce a net power output of 100 MW.

The first step in the design process is to determine the Carnot efficiency of the engine, which is given by the formula:

η = (T_H – T_L) / T_H

where η is the efficiency, T_H is the temperature of the hot reservoir, and T_L is the temperature of the cold reservoir.

Using the given temperatures, the Carnot efficiency of the engine is:

η = (800 – 300) / 800 = 0.625 or 62.5%

This means that the theoretical maximum efficiency of the engine is 62.5%, which is the maximum amount of work that can be obtained from the heat transferred between the two reservoirs.

The next step is to determine the amount of heat that needs to be transferred between the two reservoirs to produce the desired power output of 100 MW. This is calculated using the formula:

P = Q_H – Q_L

where P is the net power output, Q_H is the heat transferred from the hot reservoir, and Q_L is the heat transferred to the cold reservoir.

Using the given power output, the amount of heat that needs to be transferred is:

Q_H – Q_L = 100 MW

The final step is to determine the flow rate of the working substance in the engine, which is used to transfer the heat between the two reservoirs. The flow rate is calculated using the formula:

m_dot = P / (η * (T_H – T_L))

where m_dot is the flow rate, P is the net power output, η is the Carnot efficiency, T_H is the temperature of the hot reservoir, and T_L is the temperature of the cold reservoir.

Using the given parameters, the flow rate of the working substance is:

m_dot = 100 MW / (0.625 * (800 – 300)) = 0.267 kg/s

This means that the working substance needs to be circulated through the engine at a rate of 0.267 kg/s to transfer the required amount of heat between the two reservoirs and produce the desired power output of 100 MW.

In this case study, the Carnot engine provides a theoretical model for the power generation plant, which helps to determine the efficiency, heat transfer requirements, and flow rate of the working substance. The principles behind the Carnot engine can be applied to the design and operation of a wide range of power generation systems, including steam turbines, gas turbines, and internal combustion engines.

White paper on Carnot engine

Introduction:

The Carnot engine is a theoretical model of a heat engine that was first proposed by French engineer Sadi Carnot in 1824. The Carnot engine is based on the principles of thermodynamics and the second law of thermodynamics, which states that it is impossible to create a heat engine with 100% efficiency. The Carnot engine provides a theoretical model for understanding the limits of the efficiency of heat engines, which are widely used in a range of applications, including power generation, transportation, and industrial processes.

Working Principle:

The Carnot engine operates on a reversible thermodynamic cycle called the Carnot cycle. The Carnot cycle consists of four stages: isothermal expansion, adiabatic expansion, isothermal compression, and adiabatic compression. The working substance, which can be a gas, liquid, or a combination of both, is contained in a cylinder with a piston.

In the first stage of the cycle, the working substance is in contact with a hot reservoir and undergoes isothermal expansion. During this stage, heat is transferred into the working substance, causing it to expand and push the piston outward, doing work on the system.

In the second stage of the cycle, the working substance undergoes adiabatic expansion, which means that no heat is added or removed from the system. During this stage, the working substance continues to expand and the temperature drops.

In the third stage of the cycle, the working substance is in contact with a cold reservoir and undergoes isothermal compression. During this stage, heat is removed from the working substance, causing it to contract and pull the piston inward, doing work on the system.

In the fourth stage of the cycle, the working substance undergoes adiabatic compression, during which no heat is added or removed from the system. The working substance continues to contract and the temperature rises, and the cycle is completed.

Efficiency:

The efficiency of the Carnot engine is determined by the temperature difference between the hot and cold reservoirs. The maximum theoretical efficiency of the Carnot engine is given by the formula:

η = (T_H – T_L) / T_H

where η is the efficiency, T_H is the temperature of the hot reservoir, and T_L is the temperature of the cold reservoir.

The Carnot efficiency is always less than 1, which means that it is impossible to create a heat engine with 100% efficiency. However, the Carnot efficiency provides a theoretical upper limit for the efficiency of a heat engine, which can be used to compare the performance of different types of engines.

Applications:

The principles behind the Carnot engine have been used to develop practical heat engines that are used in a range of applications, including power generation, transportation, and industrial processes. Steam turbines, gas turbines, and internal combustion engines are all based on the principles of thermodynamics and the Carnot cycle.

In power generation, the Carnot engine provides a theoretical model for understanding the efficiency and heat transfer requirements of power plants. The efficiency of power plants can be improved by using higher temperature differences between the hot and cold reservoirs, which increases the Carnot efficiency of the engine.

Conclusion:

The Carnot engine provides a theoretical model for understanding the limits of the efficiency of heat engines. The principles behind the Carnot engine have been applied to the design and operation of a wide range of practical heat engines, which are used in power generation, transportation, and industrial processes. The Carnot efficiency provides a theoretical upper limit for the efficiency of a heat engine, which can be used to compare the performance of different types of engines and to improve their efficiency.