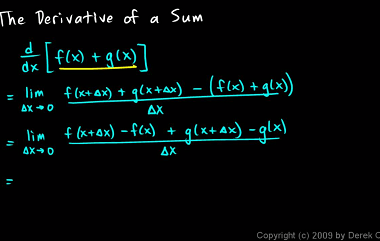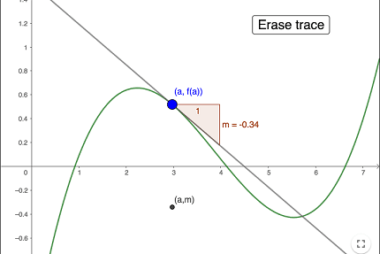
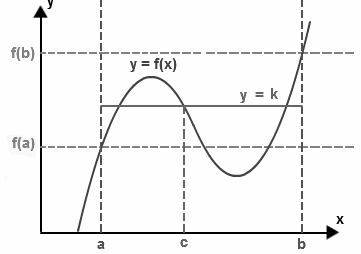
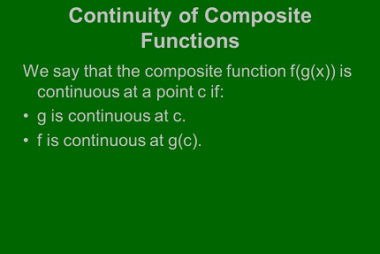
Differential calculus is a branch of calculus that deals with the study of rates of change and slopes of curves. Trigonometry is a branch of mathematics that deals with the study of triangles and the relationships between their sides and angles. The two subjects are related in that trigonometric functions, such as sine, cosine, and…
Rationality refers to the ability to think logically, make sound judgments, and make decisions based on reason rather than emotions or impulses. It involves using critical thinking skills to analyze information, evaluate evidence, and draw conclusions based on facts and evidence. In general, a rational person is someone who can separate their emotions from their…
The derivative of a polynomial is obtained by differentiating each term of the polynomial with respect to the variable. For example, let’s consider the polynomial: f(x) = 5x^3 + 2x^2 – 7x + 4 To find its derivative, we differentiate each term with respect to x: f'(x) = (d/dx)(5x^3) + (d/dx)(2x^2) – (d/dx)(7x) + (d/dx)(4)…
The chain rule is a fundamental rule in calculus that allows you to differentiate a function that is composed of two or more functions. More specifically, if you have a function f(x) that is composed of two functions g(x) and h(x), such that f(x) = g(h(x)), then the chain rule states that: f'(x) = g'(h(x))…
Let f(x) and g(x) be two functions that are differentiable at x. The derivative of their sum, h(x) = f(x) + g(x), is given by: h'(x) = f'(x) + g'(x) In other words, to find the derivative of the sum of two functions, you simply take the derivatives of each function individually and add them…
The derivative of a function represents the rate at which the function changes at each point. It is defined as the limit of the difference quotient as the distance between two points on the function approaches zero. The derivative of a function f(x) with respect to x is denoted as f'(x) or df/dx. The formula…
The intermediate value property is a property of continuous functions that states that if a continuous function f(x) takes on two values, say a and b, at two different points a and b in its domain, then it must take on every value between a and b at some point c in its domain. Formally,…
The continuity of composite functions is governed by the following theorem: Let f be a function defined on an interval I containing a point a, and let g be a function defined on an interval J containing f(a). If f is continuous at a and g is continuous at f(a), then the composite function g…
L’Hôpital’s rule is a technique used to evaluate limits of functions of the form “f(x)/g(x)” where both f(x) and g(x) approach zero (or infinity) as x approaches a particular value. The rule states that if the limit of the quotient of the derivatives of f(x) and g(x) exists, then this limit is equal to the…
The product of two functions is obtained by multiplying the two functions together at each point in their domain. If f(x) and g(x) are two functions, their product is denoted as f(x) * g(x) and is defined as: (f * g)(x) = f(x) * g(x) For example, if f(x) = x^2 and g(x) = 3x,…
