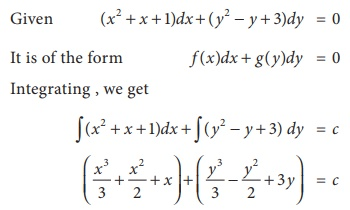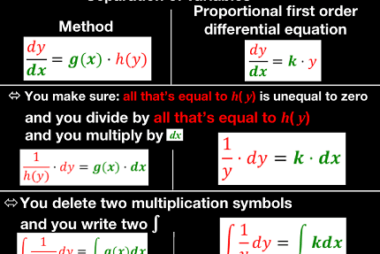
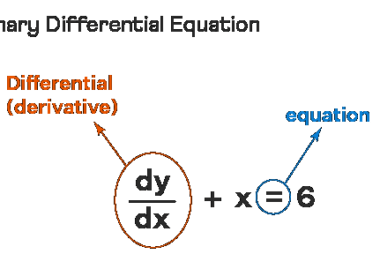
A linear first-order differential equation has the form: y’ + p(x)y = q(x) where y’ denotes the derivative of y with respect to x, p(x) and q(x) are functions of x. To solve this equation, we use an integrating factor, which is a function u(x) that we multiply both sides of the equation by. We…
Separation of variables is a method used to solve certain types of differential equations. The method involves assuming that the solution to the differential equation can be expressed as a product of two functions, each of which depends on only one of the variables in the equation. For example, consider the partial differential equation: ∂u/∂t…
A homogeneous differential equation of the first order and first degree is an equation of the form: dy/dx = f(x,y) where f(x,y) is a homogeneous function of degree 1 in x and y. In other words, if we replace y with ky and x with kx for any constant k, the function f(x,y) remains the…
Ordinary differential equations (ODEs) describe the relationships between a function and its derivatives with respect to a single independent variable. There are several ways to form ODEs, but some of the most common are: In general, the formation of ODEs requires an understanding of the underlying physical or mathematical principles that govern the system being…
Definite integrals can be used to determine the area bounded by a simple curve and the x-axis, or by a simple curve and the y-axis. The area can be found by dividing it into small rectangles, finding the area of each rectangle, and then adding up the areas of all the rectangles. This process is…
Integration by substitution is a technique used to simplify an integral by replacing the variable of integration with a new variable. This new variable is chosen so that the resulting integral becomes easier to evaluate. The basic steps for integration by substitution are as follows: Partial fraction decomposition is a technique used to simplify a…
Integration by parts is a technique used in calculus to find the integral of a product of two functions. The general formula for integration by parts is: ∫ u dv = u v – ∫ v du where u and v are functions of x, and dv and du are their differentials. To use this…
The fundamental theorem of calculus is a theorem that links the concept of differentiation with that of integration, and it has two parts. Part 1: Let f be a continuous function on the interval [a,b], and let F be a function defined by F(x) = ∫a^x f(t) dt where a ≤ x ≤ b. Then…
A definite integral is a mathematical concept that represents the area under a curve between two specified points on the x-axis. It is denoted by the symbol ∫, and is defined as: ∫[a,b] f(x) dx = F(b) – F(a) where f(x) is a function, F(x) is its antiderivative or indefinite integral, and a and b…
Definite integrals are a way to find the area under a curve between two points. One way to think about definite integrals is as the limit of a sum of rectangles. Suppose we want to find the area under the curve of a function f(x) between x=a and x=b. We can start by dividing the…
