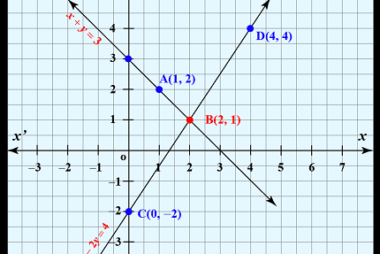
Matrices are often used to solve systems of simultaneous linear equations in two or three variables. The matrix method involves representing the coefficients of the equations and the constants as matrices, and then performing matrix operations to obtain the solution. Let’s take an example of two linear equations in two variables: 3x + 2y =…
A matrix is said to be symmetric if it is equal to its transpose. That is, for an n x n matrix A, A is symmetric if A^T = A. A matrix is said to be skew-symmetric if it is equal to the negative of its transpose. That is, for an n x n matrix…
A matrix is said to be diagonal if all its non-diagonal elements are zero. In other words, a diagonal matrix is a square matrix where all the elements outside the main diagonal are zero. The main diagonal of a matrix is the set of elements that runs from the top-left corner to the bottom-right corner…
There are several matrix operations with unique properties. Here are some of the properties of common matrix operations: These are just a few properties of some common matrix operations. There are many more properties of matrices that are important to know when working with linear algebra. What is Required Properties of these matrix operations It…
The inverse of a square matrix A of order n is denoted by A^-1 and is defined as a matrix such that the product of A and A^-1 is the identity matrix of the same order, i.e., AA^-1 = I_n. For a square matrix A of order 2, its inverse is given by the formula:…
The adjoint of a matrix is also known as the adjugate matrix or the classical adjoint of a matrix. Given an n-by-n matrix A, its adjoint is defined as the transpose of its matrix of cofactors. To understand this definition, we need to first define what the matrix of cofactors is. Let’s assume that we…
The determinant of a square matrix of order up to three can be calculated as follows: For a 1×1 matrix: The determinant of a 1×1 matrix is simply the value of the only element in the matrix. For a 2×2 matrix: The determinant of a 2×2 matrix is calculated as follows: |a b| |c d|…
Matrices are mathematical objects consisting of rows and columns of numbers. They are commonly used in many fields of mathematics, physics, engineering, and computer science. One of the most important concepts in matrix theory is the idea of elementary row and column transformations. These are operations that can be performed on a matrix to transform…
In linear algebra, the transpose of a matrix is an operation that flips the matrix over its diagonal, reflecting its rows and columns. The transpose of a matrix A is denoted by A^T. To compute the transpose of a matrix, you simply write the rows of the matrix as columns, and the columns as rows.…
Multiplication by a scalar: Multiplying a matrix by a scalar means multiplying every entry of the matrix by that scalar. For example, if A is a matrix and k is a scalar, then the product kA is obtained by multiplying every entry of A by k. Formally, if A = [a_ij] is an m x…
