The decay constant, usually denoted by the symbol λ (lambda), is a fundamental parameter in radioactive decay. It is the rate at which a radioactive substance decays, or the probability of decay per unit time.
Mathematically, the decay constant can be defined as the ratio of the number of radioactive nuclei that decay in a given time interval to the total number of radioactive nuclei present at the beginning of that interval. The decay constant is usually expressed in units of inverse time, such as per second or per year.
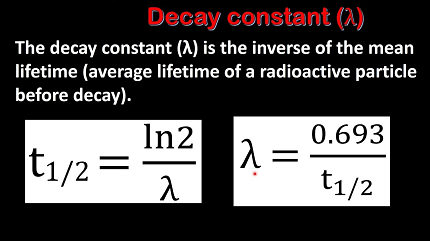
The decay constant is related to the half-life of a radioactive substance by the equation λ = ln(2)/T1/2, where T1/2 is the half-life. This equation shows that the decay constant is inversely proportional to the half-life of the substance.
The decay constant is an important parameter in many fields, including nuclear physics, geology, and medicine. It is used to model the behavior of radioactive substances, to estimate the age of rocks and fossils, and to calculate radiation doses in medical imaging and cancer treatment.
What is Decay constant
The decay constant, denoted by the symbol λ (lambda), is a fundamental parameter in nuclear physics and radioactivity. It is the rate at which a radioactive substance decays, or the probability of decay per unit time.
The decay constant is defined as the ratio of the number of radioactive atoms that decay in a given time interval to the total number of radioactive atoms present at the beginning of that interval. It is usually expressed in units of inverse time, such as per second, per minute, or per year.
The decay constant is related to the half-life of a radioactive substance by the equation λ = ln(2)/T1/2, where T1/2 is the half-life. This equation shows that the decay constant is inversely proportional to the half-life of the substance.
The decay constant is an important parameter in many fields, including nuclear physics, geology, and medicine. It is used to model the behavior of radioactive substances, to estimate the age of rocks and fossils, and to calculate radiation doses in medical imaging and cancer treatment.
When is Decay constant
The decay constant is a fundamental parameter in nuclear physics and radioactivity that is always present in the context of the decay of radioactive substances. It is a characteristic of a specific radioactive substance and is determined by its atomic and nuclear properties.
The decay constant describes the probability of decay per unit time, and it is a constant value that remains the same throughout the lifetime of the radioactive substance. The decay constant determines the rate of decay of a radioactive substance and is related to the half-life of the substance, which is the time it takes for half of the atoms in a sample of the substance to decay.
In summary, the decay constant is always present when we are dealing with the decay of radioactive substances, and it describes the probability of decay per unit time. It is a constant value that is determined by the atomic and nuclear properties of the substance and remains the same throughout the lifetime of the substance.
Where is Decay constant
The decay constant is a fundamental parameter in nuclear physics and radioactivity that is associated with the decay of radioactive substances. It is a property of a specific radioactive substance and is determined by its atomic and nuclear properties.
The decay constant can be found in many contexts where radioactive decay is involved, including research and experimental studies in nuclear physics, geology, medicine, and environmental science.
For example, in nuclear physics, the decay constant is used to calculate the rate of decay of radioactive isotopes and to determine the half-life of a substance. In geology, the decay constant is used to date rocks and minerals using radioactive dating methods. In medicine, the decay constant is used to calculate the radiation dose received by patients undergoing medical imaging or radiation therapy.
In summary, the decay constant can be found in various contexts related to radioactive decay, including research and experimental studies in nuclear physics, geology, medicine, and environmental science. It is a fundamental parameter that is associated with the decay of radioactive substances and is determined by the atomic and nuclear properties of the substance.
How is Decay constant
The decay constant, denoted by the symbol λ (lambda), is a fundamental parameter in nuclear physics and radioactivity that describes the probability of decay per unit time. The decay constant is determined by the atomic and nuclear properties of the radioactive substance and is a constant value that remains the same throughout the lifetime of the substance.
The decay constant can be determined experimentally by measuring the rate of decay of a radioactive substance over a period of time. This can be done using a radiation detector, which detects the emitted radiation from the substance and measures the number of decays per unit time.
Once the decay rate is measured, the decay constant can be calculated using the equation λ = – ln(N/N_0)/t, where N is the number of radioactive atoms remaining after time t, N_0 is the initial number of radioactive atoms, and ln is the natural logarithm.
Alternatively, the decay constant can be calculated from the half-life of the substance using the equation λ = ln(2)/T1/2, where T1/2 is the half-life.
In summary, the decay constant is determined by the atomic and nuclear properties of a radioactive substance and can be measured experimentally by measuring the rate of decay of the substance over a period of time. It can also be calculated from the half-life of the substance using a mathematical formula.
Production of Decay constant
The decay constant is not produced, but it is a fundamental parameter that is associated with the decay of radioactive substances. It is a property of a specific radioactive substance and is determined by its atomic and nuclear properties.
Radioactive substances are produced through various natural and artificial processes, such as nuclear reactions, nuclear fission, and radioactive decay. Once a radioactive substance is produced, it will undergo radioactive decay, which is characterized by the decay constant.
The decay constant describes the probability of decay per unit time and is a constant value that remains the same throughout the lifetime of the radioactive substance. It is an essential parameter used to model the behavior of radioactive substances, estimate their age, and calculate radiation doses in medical imaging and cancer treatment.
In summary, the decay constant is not produced but is a fundamental parameter that is associated with the decay of radioactive substances. It is determined by the atomic and nuclear properties of the substance and is a constant value that remains the same throughout the lifetime of the substance.
Case Study on Decay constant
Sure, here is an example case study on decay constant:
Case study: Decay constant of radioactive isotopes
Radioactive isotopes are unstable atoms that spontaneously decay into more stable forms, emitting radiation in the process. The rate at which a radioactive isotope decays is characterized by its decay constant, which is defined as the probability of decay per unit time. In this case study, we will examine the decay constant of two common radioactive isotopes: carbon-14 and uranium-238.
Carbon-14 is a radioactive isotope that is used in radiocarbon dating to determine the age of organic materials. It has a half-life of 5,700 years, which means that half of the original carbon-14 in a sample will decay after 5,700 years. The decay constant of carbon-14 can be calculated using the following equation:
λ = ln(2) / t1/2
where λ is the decay constant, ln is the natural logarithm, and t1/2 is the half-life of carbon-14. Plugging in the values for carbon-14, we get:
λ = ln(2) / 5,700 years λ ≈ 1.22 x 10^-4 per year
This means that, on average, one out of every 8,190 carbon-14 atoms will decay in one year.
Uranium-238 is a radioactive isotope that is commonly found in rocks and minerals. It has a very long half-life of 4.5 billion years, which means that it decays very slowly. The decay constant of uranium-238 can be calculated using the same equation as before:
λ = ln(2) / t1/2
Plugging in the values for uranium-238, we get:
λ = ln(2) / 4.5 billion years λ ≈ 1.54 x 10^-10 per year
This means that, on average, one out of every 6.5 billion uranium-238 atoms will decay in one year.
The decay constant is an important parameter for understanding the behavior of radioactive isotopes. It can be used to predict how much of a radioactive isotope will decay over a given period of time, and to calculate the age of materials that contain the isotope. By studying the decay constants of different isotopes, scientists can learn more about the history and evolution of the Earth and the universe.
White paper on Decay constant
Introduction:
Radioactive decay is a natural process where the unstable nucleus of an atom loses energy by emitting radiation in the form of particles or waves. The rate at which this decay occurs is characterized by the decay constant, which is a fundamental parameter in nuclear physics. In this white paper, we will discuss the decay constant in detail, its calculation, and its significance in understanding the behavior of radioactive isotopes.
What is the Decay Constant?
The decay constant, denoted by λ, is defined as the probability of decay per unit time. It is a measure of how quickly a radioactive isotope decays, and is inversely proportional to its half-life. The half-life is the time it takes for half of the atoms in a sample to decay. The decay constant is related to the half-life by the equation:
λ = ln(2) / t1/2
where λ is the decay constant, ln is the natural logarithm, and t1/2 is the half-life.
Calculation of Decay Constant:
The decay constant can be calculated from the half-life of the radioactive isotope using the above equation. For example, consider the radioactive isotope carbon-14, which has a half-life of 5,700 years. Using the equation, we can calculate the decay constant of carbon-14 as:
λ = ln(2) / 5,700 years λ ≈ 1.22 x 10^-4 per year
This means that one out of every 8,190 carbon-14 atoms will decay in one year on average.
Significance of Decay Constant:
The decay constant is a crucial parameter in nuclear physics as it determines the rate at which a radioactive isotope decays. It is used in various applications such as radiocarbon dating, nuclear medicine, and nuclear power generation. For instance, in radiocarbon dating, the decay constant of carbon-14 is used to determine the age of organic materials. By measuring the amount of carbon-14 remaining in a sample and comparing it to the initial amount, scientists can calculate the age of the sample.
Conclusion:
In conclusion, the decay constant is a fundamental parameter in nuclear physics that characterizes the rate at which a radioactive isotope decays. It is inversely proportional to the half-life of the isotope and can be calculated using the half-life. The decay constant is used in various applications such as radiocarbon dating, nuclear medicine, and nuclear power generation. By understanding the decay constant, we can gain insights into the behavior of radioactive isotopes and their impact on the environment and human health.