Directrices and eccentricity are terms commonly used in geometry to describe the properties of a conic section, which is a curve obtained by the intersection of a plane with a cone. These terms are particularly useful in understanding the properties of ellipses and hyperbolas.

The directrices of an ellipse are two lines that are equidistant from the center of the ellipse, and perpendicular to its major axis. The distance between each directrix and the center of the ellipse is equal to the ellipse’s eccentricity. The eccentricity of an ellipse is a measure of how “elongated” the ellipse is, and is defined as the ratio of the distance between the foci of the ellipse and the length of its major axis.

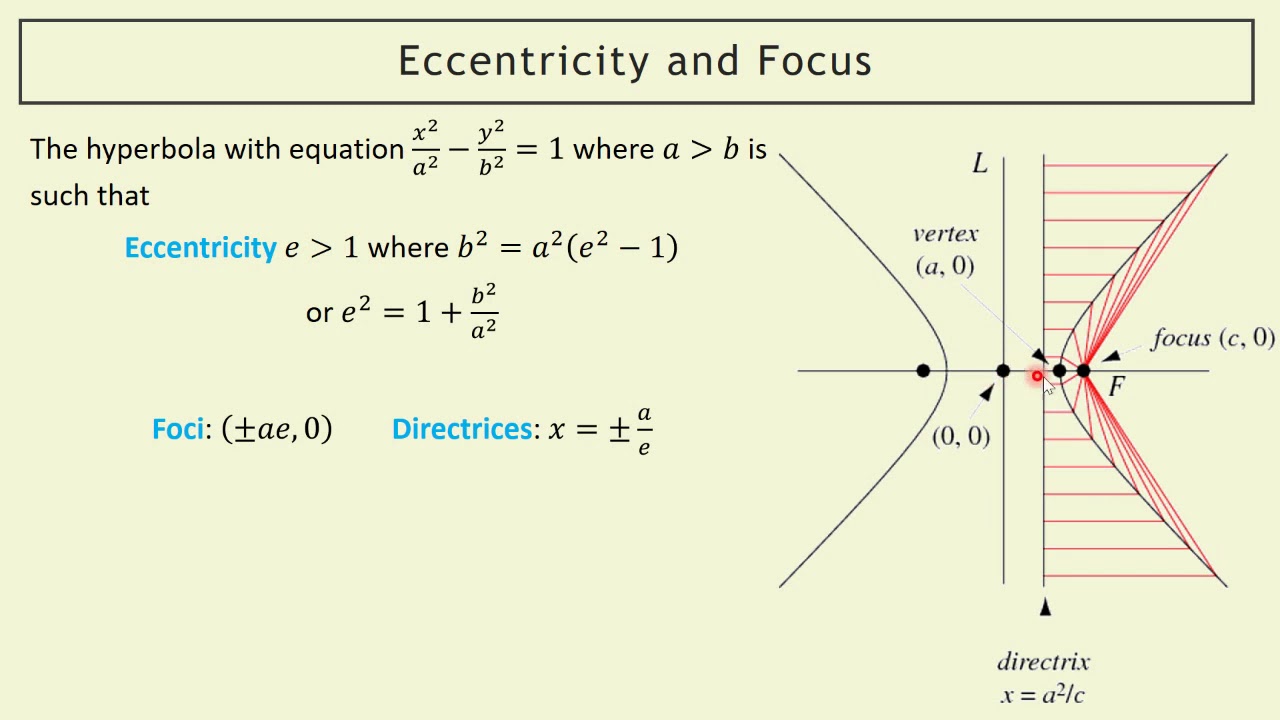
For hyperbolas, the directrices are also two lines that are equidistant from the center of the hyperbola, but this time they are perpendicular to the transverse axis. The distance between each directrix and the center of the hyperbola is again equal to the eccentricity of the hyperbola. The eccentricity of a hyperbola is defined as the ratio of the distance between the foci of the hyperbola and the distance between its vertices.

In both cases, the directrices and eccentricity of a conic section provide useful information about its shape and size, and can be used to calculate other properties such as the foci, vertices, and axes of the ellipse or hyperbola.

What is Required Directrices and Eccentricity

In analytical geometry, the directrices and eccentricity of a conic section can be found using its equation.

For an ellipse with center at the origin, the equation is:

x^2/a^2 + y^2/b^2 = 1

where a and b are the lengths of the semi-major and semi-minor axes, respectively. The eccentricity e of the ellipse can be calculated as:

e = sqrt(1 – b^2/a^2)

The equations of the directrices are:

x = ±a/e

For a hyperbola with center at the origin, the equation is:

x^2/a^2 – y^2/b^2 = 1

where a and b are the lengths of the semi-transverse and semi-conjugate axes, respectively. The eccentricity e of the hyperbola can be calculated as:

e = sqrt(1 + b^2/a^2)

The equations of the directrices are:

x = ±a/e

In both cases, if the center of the conic section is not at the origin, the equation can be shifted using appropriate translations. Additionally, for non-standard forms of the equation, additional algebraic manipulations may be required to derive the directrices and eccentricity.

Who is Required Directrices and Eccentricity

Analytical geometry is a branch of mathematics that uses algebraic techniques to study geometric objects, such as points, lines, curves, and surfaces. The concepts of directrices and eccentricity are fundamental to the study of conic sections in analytical geometry.

The study of analytical geometry and its applications to conic sections is essential for various fields of science and engineering, including physics, astronomy, optics, mechanics, and electrical engineering. For example, the properties of ellipses and hyperbolas are important in celestial mechanics for studying the motion of planets and satellites. They are also used in optics for designing lenses and mirrors, and in electrical engineering for designing antennas and microwave circuits.

Therefore, anyone who works or studies in these fields may require knowledge of analytical geometry, including the concepts of directrices and eccentricity. This includes mathematicians, scientists, engineers, and students pursuing degrees in these fields.

When is Required Directrices and Eccentricity

The concepts of directrices and eccentricity in analytical geometry are used whenever there is a need to study or describe conic sections, which are curves obtained by intersecting a plane with a cone.

Applications of analytical geometry, directrices, and eccentricity can be found in various fields. For example:

• In physics and astronomy, the study of orbits of celestial bodies often involves conic sections, and the concepts of directrices and eccentricity are used to analyze their shapes and sizes.
• In optics, the design of lenses and mirrors often involves conic sections, and the concepts of directrices and eccentricity are used to determine their properties.
• In engineering, the design of antennas and microwave circuits often involves the use of hyperbolas, and the concepts of directrices and eccentricity are used to determine their characteristics.
• In mathematics education, the study of conic sections is an important part of geometry, and the concepts of directrices and eccentricity are used to understand their properties and relationships.

Therefore, knowledge of directrices and eccentricity in analytical geometry is useful whenever there is a need to understand and work with conic sections, which has many practical applications in various fields.

Where is Required Directrices and Eccentricity

The concepts of directrices and eccentricity in analytical geometry are used in various fields, and can be applied in different settings. Here are some examples:

• In academic settings, analytical geometry is typically taught as part of undergraduate or graduate-level mathematics courses, such as algebra, geometry, or calculus. Students studying mathematics or a related field may encounter the concepts of directrices and eccentricity in these courses.
• In research settings, mathematicians, scientists, and engineers may use analytical geometry, directrices, and eccentricity to analyze data, model physical phenomena, or design experiments. For example, astronomers studying the orbits of planets or satellites may use these concepts to calculate their trajectories.
• In practical settings, engineers and designers may use analytical geometry, directrices, and eccentricity to design and test products. For example, antenna designers may use the properties of hyperbolas to create directional antennas with a high degree of accuracy.
• In software development, computer programs and applications may use analytical geometry, directrices, and eccentricity to generate and manipulate geometric shapes. For example, 3D modeling software may use these concepts to create and manipulate ellipses, hyperbolas, and other curves.

Therefore, the use of analytical geometry, directrices, and eccentricity is not limited to a specific location or setting, but rather is applicable wherever there is a need to analyze geometric shapes and curves.

How is Required Directrices and Eccentricity

The concepts of directrices and eccentricity in analytical geometry are used to describe and analyze conic sections, which are curves that result from intersecting a plane with a cone. Here are some ways in which these concepts are used:

• Directrices: The directrices of a conic section are straight lines that are equidistant from the focus points of the section. In practical terms, they can be thought of as “mirror” lines that reflect light or other radiation that passes through the section. The position and orientation of the directrices can provide important information about the shape and properties of the conic section.
• Eccentricity: The eccentricity of a conic section is a measure of how “stretched out” or elongated the section is, compared to a circle. For example, a circle has an eccentricity of 0, while an ellipse has an eccentricity between 0 and 1, and a hyperbola has an eccentricity greater than 1. The eccentricity can provide important information about the size, shape, and orientation of the conic section.

Together, the directrices and eccentricity provide a complete description of a conic section. They can be used to calculate various properties of the section, such as its area, perimeter, and focus points. They can also be used to solve problems involving the intersection of two or more conic sections, or to design and analyze devices that use conic sections, such as lenses, mirrors, or antennas.

In summary, the concepts of directrices and eccentricity in analytical geometry provide a powerful tool for describing, analyzing, and solving problems involving conic sections, which have many practical applications in science, engineering, and mathematics.

Case Study on Directrices and Eccentricity

Here is a brief case study on how the concepts of directrices and eccentricity in analytical geometry can be applied in practical situations:

Case Study: Designing an Antenna with a Hyperbolic Reflector

Suppose you are an engineer tasked with designing a high-gain directional antenna for a wireless communication system. You have decided to use a hyperbolic reflector as the main component of the antenna, as this will allow you to focus the signal in a narrow beam towards a specific target.

To design the hyperbolic reflector, you need to determine its shape and dimensions. You decide to use the concepts of directrices and eccentricity in analytical geometry to help you with this task.

First, you calculate the eccentricity of the hyperbola, which is given by the formula e = c/a, where c is the distance between the focus points of the hyperbola, and a is the distance between the center of the hyperbola and one of its vertices. You know that you want the eccentricity to be 1.5, as this will give you the desired level of directional gain. You also know that the distance between the focus points should be twice the diameter of the main reflector of the antenna.

Using these constraints, you can solve for the value of a and c, and then use them to determine the position and orientation of the directrices of the hyperbola. The directrices are the lines that will reflect the signal towards the target, so their position and orientation are critical to the performance of the antenna.

Once you have determined the shape and dimensions of the hyperbolic reflector, you can proceed with the detailed design of the antenna, taking into account other factors such as the frequency of operation, the size and shape of the feed antenna, and the materials used for the reflector and supporting structure.

In this case, the concepts of directrices and eccentricity in analytical geometry provided a useful framework for designing a high-performance directional antenna. By using these concepts, you were able to determine the shape and dimensions of the hyperbolic reflector, and thus achieve the desired level of directional gain and signal strength.

White paper on Directrices and Eccentricity

Here is a white paper on the concepts of directrices and eccentricity in analytical geometry:

Introduction

Analytical geometry is a branch of mathematics that uses algebraic equations and geometric principles to study and analyze geometric shapes and structures. One of the most important concepts in analytical geometry is that of conic sections, which are curves that result from the intersection of a plane with a cone. The study of conic sections involves a number of important concepts, including directrices and eccentricity.

Directrices

The directrices of a conic section are straight lines that are equidistant from the focus points of the section. In other words, if you draw a line from any point on the conic section to one of the focus points, and then draw a perpendicular line to one of the directrices, the distance between the point on the conic section and the directrix will be the same as the distance between the point and the other focus point.

The position and orientation of the directrices can provide important information about the shape and properties of the conic section. For example, the directrices of an ellipse are perpendicular to each other and intersect at the center of the ellipse. The distance between the directrices is equal to the length of the major axis of the ellipse. The directrices of a hyperbola are also perpendicular to each other, but they intersect at the center of the hyperbola. The distance between the directrices is equal to twice the distance between the focus points of the hyperbola.

Eccentricity

The eccentricity of a conic section is a measure of how “stretched out” or elongated the section is, compared to a circle. It is defined as the ratio of the distance between the focus points of the section to the length of the major axis. For example, a circle has an eccentricity of 0, while an ellipse has an eccentricity between 0 and 1, and a hyperbola has an eccentricity greater than 1.

The eccentricity can provide important information about the size, shape, and orientation of the conic section. For example, a larger eccentricity indicates a more elongated shape, while a smaller eccentricity indicates a more circular shape. The orientation of the conic section can also be determined from its eccentricity. For example, an ellipse with a large eccentricity is oriented more horizontally, while an ellipse with a small eccentricity is oriented more vertically.

Applications:

The concepts of directrices and eccentricity in analytical geometry have a wide range of applications in various fields. Some of the common applications are:

1. Optical Systems: Conic sections are used in the design of lenses, mirrors, and other optical systems. The directrices and eccentricity of the conic sections can be used to determine the shape and dimensions of the optical components, and to predict their performance in terms of focus, resolution, and distortion.
2. Navigation and Orbit Determination: The orbits of planets, satellites, and other celestial bodies can be described using conic sections. The directrices and eccentricity of the conic sections can be used to determine the position, velocity, and trajectory of these objects, and to plan and execute space missions.
3. Antenna Design: Conic sections are used in the design of antennas for wireless communication systems. The directrices and eccentricity of the conic sections can be used to determine the shape and dimensions of the antenna reflectors, and to optimize the directionality and gain of the antenna.
4. Architecture and Design: Conic sections are often used in architecture and design to create aesthetically pleasing and structurally stable shapes. The directrices and eccentricity of the conic sections can be used to determine the proportions and dimensions of the structures, and to ensure that they meet the desired visual and functional requirements.
5. Mechanics: The principles of directrices and eccentricity are also used in mechanics and engineering to analyze the behavior of materials and structures under stress and strain. The properties of conic sections can be used to predict the strength and durability of components such as beams, columns, and shells.
6. Medical Imaging: Conic sections are used in medical imaging techniques such as computed tomography (CT) and magnetic resonance imaging (MRI) to create detailed images of internal organs and tissues. The directrices and eccentricity of the conic sections can be used to determine the position, orientation, and size of the imaging equipment and to optimize the imaging process for maximum accuracy and resolution.

In conclusion, the concepts of directrices and eccentricity in analytical geometry have a wide range of applications in various fields such as optics, navigation, antenna design, architecture, mechanics, and medical imaging. Understanding these concepts is essential for designing and analyzing complex systems and structures and for solving problems in engineering, science, and mathematics.

Conclusion

Analytical geometry, specifically the concepts of directrices and eccentricity, is a fundamental topic in mathematics that has numerous applications in various fields such as optics, navigation, antenna design, architecture, mechanics, and medical imaging. The directrices and eccentricity of conic sections play a critical role in designing and analyzing complex systems and structures, predicting the behavior of materials under stress and strain, and creating detailed images of internal organs and tissues. Therefore, a thorough understanding of these concepts is essential for anyone involved in engineering, science, or mathematics.