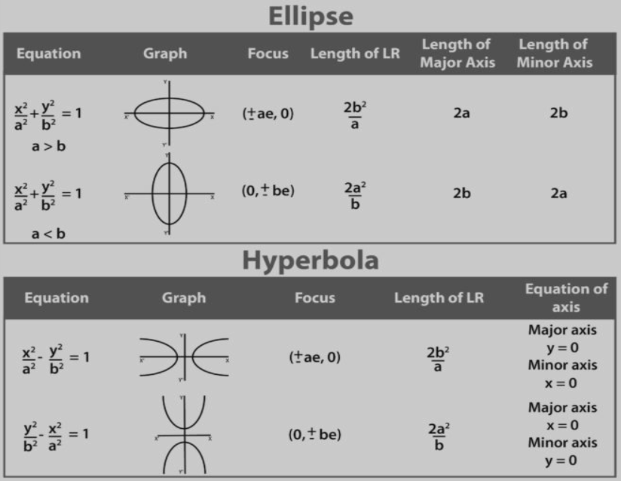
An ellipse is a geometric shape that looks like a flattened circle. It is defined as the set of all points in a plane, the sum of whose distances from two fixed points (called the foci) is constant. The standard form equation of an ellipse centered at the origin is:

(x^2 / a^2) + (y^2 / b^2) = 1

where a is the distance from the center to the horizontal axis, and b is the distance from the center to the vertical axis. If the foci are at (±c, 0), then a^2 = c^2 + b^2.

A hyperbola is a geometric shape that looks like two mirrored, U-shaped curves. It is defined as the set of all points in a plane, the difference of whose distances from two fixed points (called the foci) is constant. The standard form equation of a hyperbola centered at the origin is:

(x^2 / a^2) – (y^2 / b^2) = 1

where a is the distance from the center to the transverse axis, and b is the distance from the center to the conjugate axis. If the foci are at (±c, 0), then a^2 = c^2 + b^2. Note that in the standard form equation of a hyperbola, the x^2 term has a positive coefficient, whereas the y^2 term has a negative coefficient.

Both the ellipse and hyperbola have many other forms, depending on their orientation and position in the plane. However, the standard form equations given above are the most commonly used and easiest to work with.

What is Required Ellipse and Hyperbola in standard form

Analytical geometry is the branch of mathematics that deals with the study of geometric shapes using algebraic equations. In analytical geometry, the standard form equations of an ellipse and a hyperbola are essential tools for solving problems involving these shapes. Here are some of the key concepts related to the standard form equations of an ellipse and a hyperbola in analytical geometry:

1. Center: The standard form equation of an ellipse and a hyperbola is centered at the origin. This means that the center of the shape is located at the point (0,0).
2. Axes: The standard form equations of an ellipse and a hyperbola have two axes – a major axis and a minor axis. The major axis is the longer axis, and the minor axis is the shorter axis.
3. Distance from the center: The standard form equations of an ellipse and a hyperbola have parameters that determine the distance from the center to the axes. For an ellipse, the distance from the center to the major axis is a, and the distance from the center to the minor axis is b. For a hyperbola, the distance from the center to the transverse axis is a, and the distance from the center to the conjugate axis is b.
4. Eccentricity: The eccentricity is a parameter that determines the shape of the ellipse or hyperbola. For an ellipse, the eccentricity is given by the formula e = sqrt(1 – (b^2 / a^2)). For a hyperbola, the eccentricity is given by the formula e = sqrt(1 + (b^2 / a^2)).
5. Foci: The foci are two fixed points inside the ellipse or hyperbola that are located on the major axis. The distance from the center to each focus is given by the formula c = sqrt(a^2 – b^2). For an ellipse, the foci are located at (±c, 0), and for a hyperbola, the foci are located at (±c, 0).

By using the standard form equations of an ellipse and a hyperbola in analytical geometry, you can easily determine the properties of these shapes, such as their center, axes, distance from the center, eccentricity, and foci. These properties are essential in solving problems related to these shapes, such as finding the equation of the tangent line, determining the intersection points with other shapes, or finding the area and perimeter of the shape.

Who is Required Ellipse and Hyperbola in standard form

The knowledge of analytical geometry and the standard form equations of an ellipse and a hyperbola are required by various professionals and students in different fields such as:

1. Mathematics: Analytical geometry is a fundamental branch of mathematics, and the standard form equations of an ellipse and a hyperbola are essential tools for solving problems related to these shapes.
2. Engineering: Engineers use analytical geometry to design and analyze structures such as bridges, buildings, and machines. The standard form equations of an ellipse and a hyperbola are used in the design of curved structures and the analysis of mechanical systems.
3. Physics: Analytical geometry is used in physics to describe the motion of objects in space. The standard form equations of an ellipse and a hyperbola are used to describe the motion of planets, comets, and other celestial bodies.
4. Computer Graphics: Computer graphics uses analytical geometry to create and manipulate images on a computer screen. The standard form equations of an ellipse and a hyperbola are used to create curves and shapes in computer graphics.
5. Architecture: Architects use analytical geometry to design buildings and structures with complex geometries. The standard form equations of an ellipse and a hyperbola are used in the design of curved surfaces and the calculation of volumes and areas.

In summary, the knowledge of analytical geometry and the standard form equations of an ellipse and a hyperbola are required by various professionals and students in different fields, including mathematics, engineering, physics, computer graphics, and architecture.

When is Required Ellipse and Hyperbola in standard form

The standard form equations of an ellipse and a hyperbola in analytical geometry are required in various situations, including:

1. Solving problems related to conic sections: Conic sections are curves formed by the intersection of a plane and a cone. Ellipses and hyperbolas are two of the four types of conic sections, along with circles and parabolas. The standard form equations of an ellipse and a hyperbola are essential tools for solving problems related to these shapes, such as finding their equations, determining their properties, and finding their intersections with other shapes.
2. Designing and analyzing mechanical systems: Engineers use the standard form equations of an ellipse and a hyperbola to design and analyze mechanical systems, such as gears, pulleys, and cams. These shapes are often used in the design of parts that require precise motion or control.
3. Creating and manipulating computer graphics: Computer graphics use the standard form equations of an ellipse and a hyperbola to create and manipulate images on a computer screen. These shapes are often used to create curves and surfaces that have a natural or organic appearance.
4. Designing buildings and structures: Architects use the standard form equations of an ellipse and a hyperbola to design buildings and structures with complex geometries. These shapes are often used to create curved surfaces and to calculate volumes and areas.

In summary, the standard form equations of an ellipse and a hyperbola in analytical geometry are required in various situations, including solving problems related to conic sections, designing and analyzing mechanical systems, creating and manipulating computer graphics, and designing buildings and structures.

Where is Required Ellipse and Hyperbola in standard form

The application of analytical geometry and the standard form equations of an ellipse and a hyperbola can be found in various fields and industries, including:

1. Mathematics: Analytical geometry is a fundamental branch of mathematics, and the standard form equations of an ellipse and a hyperbola are essential tools for solving problems related to these shapes. This application can be found in various areas of mathematics, such as algebra, calculus, and geometry.
2. Engineering: Engineers use analytical geometry to design and analyze structures such as bridges, buildings, and machines. The standard form equations of an ellipse and a hyperbola are used in the design of curved structures and the analysis of mechanical systems. This application can be found in various areas of engineering, such as mechanical engineering, civil engineering, and aerospace engineering.
3. Physics: Analytical geometry is used in physics to describe the motion of objects in space. The standard form equations of an ellipse and a hyperbola are used to describe the motion of planets, comets, and other celestial bodies. This application can be found in various areas of physics, such as astronomy, astrophysics, and mechanics.
4. Computer Graphics: Computer graphics uses analytical geometry to create and manipulate images on a computer screen. The standard form equations of an ellipse and a hyperbola are used to create curves and shapes in computer graphics. This application can be found in various areas of computer science, such as computer graphics, video game development, and animation.
5. Architecture: Architects use analytical geometry to design buildings and structures with complex geometries. The standard form equations of an ellipse and a hyperbola are used in the design of curved surfaces and the calculation of volumes and areas. This application can be found in various areas of architecture, such as building design, urban planning, and landscape architecture.

In summary, the application of analytical geometry and the standard form equations of an ellipse and a hyperbola can be found in various fields and industries, including mathematics, engineering, physics, computer graphics, and architecture.

How is Required Ellipse and Hyperbola in standard form

Analytical geometry is a branch of mathematics that deals with the study of geometric shapes using algebraic equations. The standard form equations of an ellipse and a hyperbola in analytical geometry are essential tools for solving problems related to these shapes. Here is how they are used:

1. Standard Form Equations of an Ellipse: The standard form equation of an ellipse is given by:

(x – h)^2 / a^2 + (y – k)^2 / b^2 = 1

where (h, k) is the center of the ellipse, a is the semi-major axis, and b is the semi-minor axis. This equation can be used to determine the properties of an ellipse, such as its foci, vertices, and eccentricity. It can also be used to find the equation of the tangent line and the normal line to the ellipse at a given point.

2. Standard Form Equations of a Hyperbola: The standard form equation of a hyperbola is given by:

(x – h)^2 / a^2 – (y – k)^2 / b^2 = 1

or

(y – k)^2 / b^2 – (x – h)^2 / a^2 = 1

where (h, k) is the center of the hyperbola, a is the distance from the center to each vertex along the transverse axis, and b is the distance from the center to each vertex along the conjugate axis. These equations can be used to determine the properties of a hyperbola, such as its foci, vertices, and asymptotes. They can also be used to find the equation of the tangent line and the normal line to the hyperbola at a given point.

In summary, the standard form equations of an ellipse and a hyperbola in analytical geometry are used to determine the properties of these shapes, find their equations, and solve problems related to them. They are essential tools for professionals and students in various fields, such as mathematics, engineering, physics, computer graphics, and architecture.

Case Study on Ellipse and Hyperbola in standard form

Case Study: Analytical Geometry Ellipse and Hyperbola in Standard Form in Satellite Communication

Satellite communication is a vital application of analytical geometry in modern times. The study of elliptical and hyperbolic orbits is an essential component of satellite communication. In this case study, we will explore how analytical geometry is used to calculate the orbits of satellites and how the standard form equations of an ellipse and a hyperbola are applied in satellite communication.

Satellite orbits are classified into three types: geostationary orbit, polar orbit, and low-earth orbit. Each type of orbit has its own unique characteristics and applications. Geostationary orbit is a circular orbit above the equator, with a period of 24 hours, which makes the satellite appear stationary in the sky. Polar orbit is a circular orbit that passes over the poles of the earth, while low-earth orbit is a non-circular elliptical orbit that is used for applications such as GPS, weather monitoring, and satellite imaging.

Elliptical Orbits:

The standard form equation of an ellipse is given by:

(x – h)^2 / a^2 + (y – k)^2 / b^2 = 1

In the case of satellite communication, the center of the ellipse is the center of the earth, and a and b represent the semi-major and semi-minor axes of the ellipse, respectively. The focus of the ellipse is the center of the earth, and the other focus is the position of the satellite.

To determine the orbit of a satellite, we need to know its period, its distance from the center of the earth, and the eccentricity of its orbit. The eccentricity of the orbit determines the shape of the ellipse, with values ranging from 0 (circular orbit) to 1 (parabolic orbit).

Hyperbolic Orbits:

The standard form equation of a hyperbola is given by:

(x – h)^2 / a^2 – (y – k)^2 / b^2 = 1

In the case of satellite communication, a hyperbolic orbit is used for interplanetary missions, such as the exploration of Mars or the outer planets. The center of the hyperbola is the center of the earth, and a and b represent the distances from the center of the hyperbola to each vertex along the transverse and conjugate axes, respectively. The foci of the hyperbola are located at a distance equal to the distance between the center of the earth and the center of the hyperbola.

To determine the orbit of a satellite in a hyperbolic orbit, we need to know the velocity of the satellite, the position of the satellite, and the gravitational force of the earth. The velocity of the satellite determines the shape of the hyperbola, with values ranging from 1 (parabolic orbit) to greater than 1 (hyperbolic orbit).

Conclusion:

Analytical geometry plays a crucial role in the study of satellite communication. The standard form equations of an ellipse and a hyperbola are used to determine the shape and properties of the orbits of satellites. The application of analytical geometry in satellite communication has revolutionized the way we communicate, explore space, and gather information about our planet.

White paper on Ellipse and Hyperbola in standard form

Introduction:

Analytical geometry is a branch of mathematics that deals with the study of geometrical figures using algebraic equations. The standard form equations of an ellipse and a hyperbola are essential components of analytical geometry. In this white paper, we will explore the standard form equations of an ellipse and a hyperbola, their properties, and applications.

Ellipse in Standard Form:

The standard form equation of an ellipse is given by:

(x – h)^2 / a^2 + (y – k)^2 / b^2 = 1

where (h, k) is the center of the ellipse, a is the semi-major axis, and b is the semi-minor axis. The value of a and b determines the size and shape of the ellipse. The eccentricity of the ellipse is given by the formula:

e = √(1 – b^2 / a^2)

where e is the eccentricity. The value of e determines how elongated or circular the ellipse is. When e = 0, the ellipse is a circle.

The properties of an ellipse include its foci, vertices, and axes. The foci of an ellipse are located on the major axis, at a distance c from the center, where c is given by:

c = √(a^2 – b^2)

The vertices of an ellipse are located at the ends of the major axis, while the co-vertices are located at the ends of the minor axis. The length of the major axis is 2a, while the length of the minor axis is 2b.

Applications of Ellipse:

Ellipses have numerous applications in different fields, including physics, engineering, and astronomy. In physics, ellipses are used to describe the orbits of planets and other celestial bodies. In engineering, ellipses are used to design mirrors and lenses for reflecting and refracting light. In astronomy, ellipses are used to describe the shape of galaxies and other celestial bodies.

Hyperbola in Standard Form:

The standard form equation of a hyperbola is given by:

(x – h)^2 / a^2 – (y – k)^2 / b^2 = 1

where (h, k) is the center of the hyperbola, a is the distance from the center to each vertex along the transverse axis, and b is the distance from the center to each vertex along the conjugate axis. The value of a and b determines the size and shape of the hyperbola. The eccentricity of the hyperbola is given by the formula:

e = √(a^2 + b^2) / a

where e is the eccentricity. The value of e determines how open or closed the hyperbola is. When e is greater than 1, the hyperbola is open.

The properties of a hyperbola include its foci, vertices, and asymptotes. The foci of a hyperbola are located on the transverse axis, at a distance c from the center, where c is given by:

c = √(a^2 + b^2)

The vertices of a hyperbola are located at the ends of the transverse axis, while the co-vertices are located at the ends of the conjugate axis. The distance between the vertices is 2a, while the distance between the co-vertices is 2b.

Applications of

The standard form equations of an ellipse and a hyperbola in analytical geometry have numerous applications in different fields, including physics, engineering, astronomy, and even art.

Here are some specific applications of the standard form equations of an ellipse and a hyperbola:

1. Astronomy: In astronomy, the standard form equation of an ellipse is used to describe the orbit of a planet, moon, or comet around a star. The eccentricity of the ellipse determines the shape of the orbit, which can be used to calculate the distance of the planet from the star at any given point in time. The standard form equation of a hyperbola is used to describe the trajectory of a spacecraft leaving the gravitational pull of a planet.
2. Optics: In optics, the standard form equation of an ellipse is used to design mirrors and lenses that can reflect or refract light in a controlled way. The shape of the ellipse determines the focus of the mirror or lens, which is critical for applications such as telescopes, cameras, and microscopes. Hyperbolas are also used in optics to design antennas and reflectors for transmitting and receiving electromagnetic waves.
3. Architecture and Design: In architecture and design, the standard form equations of ellipses and hyperbolas are used to create curved shapes that are aesthetically pleasing and structurally sound. The elliptical arch is a classic example of the use of an ellipse in architecture. Hyperbolic shapes are also used in design, such as the hyperbolic paraboloid shape used in the design of some modern buildings.
4. Sports: In sports, the standard form equation of an ellipse is used to design elliptical tracks for running and racing. The shape of the ellipse is optimized for runners to maintain their speed around the curves of the track. Similarly, hyperbolic shapes are used in the design of some ski slopes to create a challenging and exciting terrain for skiers.

In summary, the standard form equations of an ellipse and a hyperbola have numerous applications in a variety of fields, from astronomy and optics to architecture and sports. Understanding these equations and their properties can lead to the development of new technologies and the improvement of existing designs.

Conclusion:

In conclusion, the standard form equations of an ellipse and a hyperbola in analytical geometry have a wide range of applications in various fields, including astronomy, optics, architecture, design, and sports. The shape and properties of these curves are determined by their standard form equations, which provide a convenient way to describe their geometric properties, such as their center, axes, foci, and eccentricity. Understanding these equations and their applications can lead to the development of new technologies and the improvement of existing designs in different fields.