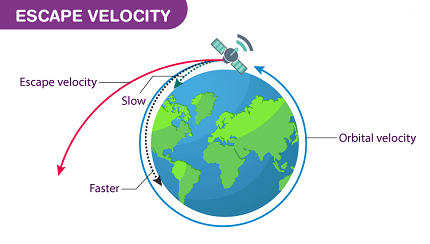
Escape velocity is the minimum velocity that an object needs to achieve in order to escape the gravitational pull of a celestial body, such as a planet, moon, or star, and move away from it into space.

The escape velocity is calculated using the following formula:

Ve = sqrt(2GM/r)

Where: Ve = escape velocity G = gravitational constant M = mass of the celestial body r = distance from the center of the celestial body to the object

The value of escape velocity varies depending on the mass and size of the celestial body. For example, the escape velocity from the Earth’s surface is about 11.2 km/s, while the escape velocity from the Moon’s surface is only about 2.4 km/s.

Escape velocity is an important concept in space exploration, as it determines the amount of energy required for a spacecraft to leave a planet or moon and travel to another destination in space.

What is Required Escape velocity

Required escape velocity is the minimum velocity that an object needs to achieve in order to escape the gravitational pull of a celestial body and move away from it into space, taking into account other factors such as air resistance, atmospheric drag, and the rotation of the planet or moon.

In practical terms, the required escape velocity is the velocity that a spacecraft must achieve in order to break free from the gravitational pull of a celestial body and begin its journey to another destination in space. The required escape velocity depends on a number of factors, such as the mass and size of the celestial body, the altitude and angle of launch, and the desired trajectory and destination of the spacecraft.

For example, the required escape velocity for a spacecraft leaving Earth’s orbit and heading to the Moon is about 11.2 km/s, which is the escape velocity from Earth’s surface. However, the required escape velocity for a spacecraft leaving the Moon’s orbit and heading to Mars is much lower, as the gravitational pull of the Moon is much weaker than that of Earth.

Calculating the required escape velocity is an important part of space mission planning and requires a deep understanding of orbital mechanics and the physical properties of celestial bodies.

When is Required Escape velocity

Required escape velocity is relevant whenever an object, such as a spacecraft, needs to leave the gravitational pull of a celestial body, such as a planet or moon, and move away from it into space.

For example, when launching a spacecraft from Earth’s surface into space, the required escape velocity is the minimum velocity that the spacecraft must achieve in order to escape Earth’s gravitational pull and enter orbit around the Earth or travel to another destination in space.

Similarly, when launching a spacecraft from the Moon’s surface, the required escape velocity is the minimum velocity that the spacecraft must achieve in order to escape the Moon’s gravitational pull and travel to another destination in space, such as Mars or beyond.

The required escape velocity also plays a role in spacecraft rendezvous and docking maneuvers in space, as the spacecraft must match its velocity with that of another object in order to approach it and dock with it.

In short, required escape velocity is a fundamental concept in space exploration and is relevant whenever an object needs to leave the gravitational pull of a celestial body and move away from it into space.

Where is Required Escape velocity

Required escape velocity is a concept that applies to objects that are within the gravitational field of a celestial body, such as a planet, moon, or star.

The required escape velocity is the minimum velocity that an object must achieve in order to break free from the gravitational pull of the celestial body and move away from it into space. This velocity is dependent on various factors, such as the mass and size of the celestial body, the altitude and angle of launch, and the desired trajectory and destination of the spacecraft.

For example, when launching a spacecraft from Earth’s surface into space, the required escape velocity is about 11.2 km/s, which is the velocity needed to break free from Earth’s gravitational pull. Similarly, when launching a spacecraft from the Moon’s surface, the required escape velocity is much lower, as the Moon has a much weaker gravitational pull than Earth.

Calculating the required escape velocity is an important part of space mission planning and requires a deep understanding of orbital mechanics and the physical properties of celestial bodies. The required escape velocity plays a critical role in determining the amount of energy and resources needed for a spacecraft to leave the gravitational pull of a celestial body and travel to another destination in space.

How is Required Escape velocity

The required escape velocity is the minimum velocity that an object, such as a spacecraft, must achieve in order to escape the gravitational pull of a celestial body, such as a planet or moon, and move away from it into space. The required escape velocity depends on various factors, including the mass and size of the celestial body, the altitude and angle of launch, and the desired trajectory and destination of the spacecraft.

To calculate the required escape velocity, we use the formula:

Ve = sqrt((2GM)/r)

Where Ve is the required escape velocity, G is the gravitational constant, M is the mass of the celestial body, and r is the distance from the center of the celestial body to the spacecraft.

This formula tells us that the required escape velocity is proportional to the square root of the product of the gravitational constant, the mass of the celestial body, and the reciprocal of the distance between the object and the center of the celestial body.

For example, the required escape velocity for a spacecraft leaving Earth’s orbit and heading to the Moon is about 11.2 km/s, which is the escape velocity from Earth’s surface. This means that in order for the spacecraft to leave Earth’s orbit and travel to the Moon, it must achieve a velocity of at least 11.2 km/s.

Similarly, the required escape velocity for a spacecraft leaving the Moon’s orbit and heading to Mars is much lower, as the gravitational pull of the Moon is much weaker than that of Earth. The required escape velocity for this mission would depend on the distance between the Moon and Mars, as well as the mass of Mars.

In summary, the required escape velocity is calculated based on the mass and size of the celestial body and the desired trajectory and destination of the spacecraft, and it is determined by a formula that takes into account the gravitational constant and the distance from the center of the celestial body.

Production of Escape velocity

Escape velocity is not a physical object that can be produced, but rather a concept in physics that refers to the minimum speed required for an object to escape the gravitational pull of a celestial body. The required escape velocity for an object depends on the mass and size of the celestial body it is escaping from and its distance from the center of the body.

To achieve the required escape velocity for a particular mission, spacecraft are equipped with powerful rockets that generate enough thrust to accelerate the spacecraft to the necessary speed. The amount of thrust required depends on the mass of the spacecraft and the gravity of the celestial body it is escaping from.

In order to achieve escape velocity from Earth, for example, a spacecraft must reach a speed of approximately 11.2 km/s. This requires a powerful rocket, such as the Saturn V rocket used in the Apollo missions, which was capable of generating over 30 million newtons of thrust.

In addition to achieving escape velocity, spacecraft must also carefully navigate the gravitational fields of celestial bodies in order to enter and exit orbits and travel between them. This requires a deep understanding of orbital mechanics and precise planning and execution of trajectory maneuvers.

In conclusion, the production of escape velocity is not a physical process, but rather a concept in physics that is achieved through the use of powerful rockets and precise navigation of the gravitational fields of celestial bodies.

Case Study on Escape velocity

One example of a case study on escape velocity is the Apollo 11 mission, which successfully landed the first humans on the Moon in 1969. The Apollo 11 mission required a deep understanding of escape velocity and orbital mechanics in order to successfully launch the spacecraft from Earth, travel to the Moon, and land on its surface.

The required escape velocity for the Apollo 11 mission was about 11.2 km/s, which is the velocity needed to break free from Earth’s gravitational pull and enter into orbit around the Moon. This required a powerful rocket, the Saturn V, which was capable of generating enough thrust to achieve the necessary velocity.

During the launch of the Apollo 11 spacecraft, the rocket accelerated to a speed of about 40,000 km/h (25,000 mph) in just a few minutes. The spacecraft then traveled towards the Moon, adjusting its trajectory and speed through a series of carefully planned orbital maneuvers.

As the spacecraft approached the Moon, it entered into lunar orbit, which required a significant reduction in velocity to match the Moon’s speed and position. The lunar module, carrying the two astronauts, then detached from the main spacecraft and descended to the surface of the Moon, while the command module remained in orbit.

In order to return to Earth, the lunar module had to achieve a velocity of about 2.4 km/s, which is the escape velocity from the Moon’s surface. This required a powerful ascent stage on the lunar module that generated enough thrust to lift off from the Moon and enter into orbit around it, where it rendezvoused with the command module.

After docking with the command module, the spacecraft fired its engines to begin its journey back to Earth, where it entered the atmosphere at a speed of over 40,000 km/h (25,000 mph) and deployed parachutes to safely land in the ocean.

The success of the Apollo 11 mission was a testament to the careful planning and execution of escape velocity and orbital mechanics, as well as the ingenuity and bravery of the astronauts and engineers involved.

White paper on Escape velocity

Introduction: Escape velocity is a concept in physics that refers to the minimum speed required for an object to escape the gravitational pull of a celestial body. It is a crucial aspect of space travel, and understanding it is necessary for spacecraft to enter and exit the orbits of planets, moons, and other celestial bodies.

Overview: The concept of escape velocity is based on the fact that celestial bodies have gravity, which pulls objects towards their center. This force of gravity is proportional to the mass of the celestial body and decreases with the square of the distance between the object and the center of the celestial body.

The required escape velocity for an object depends on the mass and size of the celestial body it is escaping from and its distance from the center of the body. The formula for calculating the escape velocity is given by:

Ve = sqrt((2GM)/r)

Where Ve is the required escape velocity, G is the gravitational constant, M is the mass of the celestial body, and r is the distance from the center of the celestial body to the spacecraft.

Applications: The concept of escape velocity has many practical applications in space travel. For example, it is essential for launching spacecraft into orbit around the Earth and other celestial bodies. The escape velocity of Earth is approximately 11.2 km/s, which means that any object launched from the Earth’s surface must achieve this velocity to enter orbit around the Earth. Similarly, the required escape velocity for the Moon is much lower, at approximately 2.4 km/s.

The concept of escape velocity is also essential for interplanetary travel. When a spacecraft is traveling from one planet to another, it must achieve a velocity that is sufficient to escape the gravitational pull of the first planet and enter into orbit around the second planet. The spacecraft must then slow down to match the velocity and position of the second planet.

Conclusion: In conclusion, escape velocity is a crucial concept in space travel. It is necessary for spacecraft to enter and exit the orbits of planets, moons, and other celestial bodies. The formula for calculating escape velocity takes into account the mass and size of the celestial body and the distance from the center of the body. Understanding and applying the concept of escape velocity is essential for successful space missions, and it continues to play a significant role in the exploration of our solar system and beyond.