Even and odd functions

Even and odd functions are two types of functions defined in mathematics.

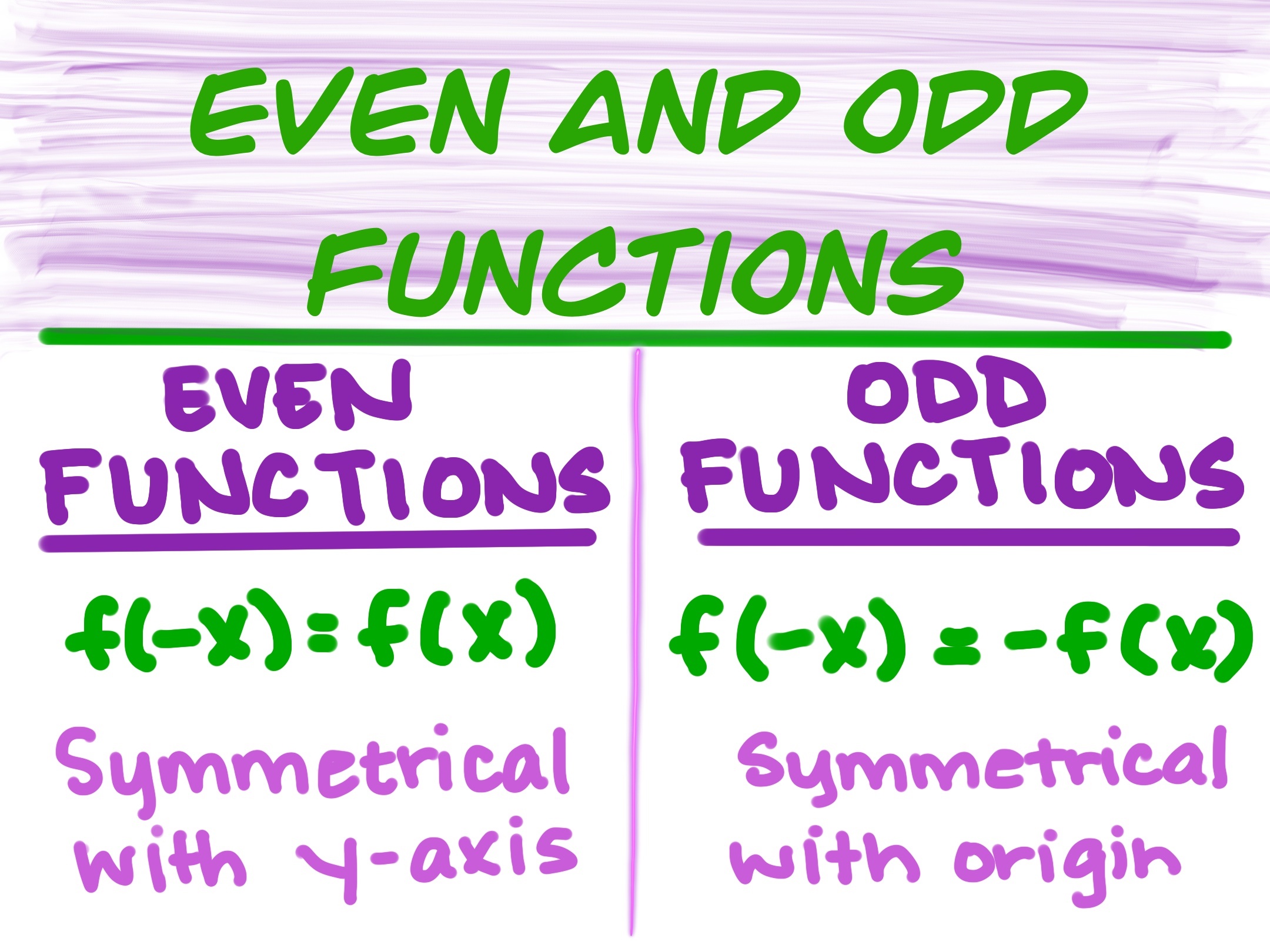
An even function is a function f(x) that satisfies the following property: f(-x) = f(x) for all x in the domain of the function. In other words, if you reflect the graph of an even function about the y-axis, the result is the same graph as the original function. Examples of even functions include f(x) = x² and f(x) = cos(x).

On the other hand, an odd function is a function g(x) that satisfies the following property: g(-x) = -g(x) for all x in the domain of the function. In other words, if you reflect the graph of an odd function about the origin, the result is the same graph as the original function, but with the y-axis flipped. Examples of odd functions include g(x) = x³ and g(x) = sin(x).

It’s worth noting that some functions are neither even nor odd. For example, the function f(x) = x is neither even nor odd, since f(-x) = -x and f(x) = x are not equal.

What is Required even and odd functions

One common use of even and odd functions in mathematics is in Fourier analysis, where functions are decomposed into their constituent frequencies. In this context, it is helpful to know whether a function is even or odd, since this affects the form of its Fourier series.

Another use of even and odd functions is in integration. If a function is even, then its integral over the interval [-a, a] is simply twice the integral over the interval [0, a]. This is because the positive and negative parts of the function cancel out when integrated over the symmetric interval. Similarly, if a function is odd, then its integral over the interval [-a, a] is zero, since the positive and negative parts of the function cancel out exactly.

In some contexts, it may be required to express a given function as a sum of an even function and an odd function. This can be done by using the even and odd parts of the function, which are defined as follows:

The even part of a function f(x) is given by (f(x) + f(-x))/2.
The odd part of a function f(x) is given by (f(x) – f(-x))/2.

The even and odd parts of a function are themselves even and odd, respectively, and they add up to the original function. By expressing a given function as the sum of its even and odd parts, it is sometimes easier to analyze or integrate the function.

Who is Required even and odd functions

Even and odd functions are important in many areas of mathematics and science, and as such, they are used by a wide range of people in different fields. Some of the people who may require knowledge of even and odd functions include:

Mathematicians: Even and odd functions are fundamental concepts in mathematics, and mathematicians use them extensively in areas such as Fourier analysis, differential equations, and group theory.
Physicists: Even and odd functions are used in physics to describe physical phenomena that exhibit symmetry or anti-symmetry, such as wavefunctions in quantum mechanics and electric fields in electromagnetism.
Engineers: Engineers use even and odd functions in signal processing, control theory, and other areas where Fourier analysis is applied.
Computer scientists: Even and odd functions are used in computer science and digital signal processing to represent and manipulate signals and data.
Economists: Even and odd functions are used in economics to analyze time series data, such as stock prices and economic indicators.
Statisticians: Even and odd functions are used in statistics to model and analyze data, particularly in time series analysis and spectral analysis.

In summary, anyone who works with functions, signals, or data may need to understand even and odd functions in order to better analyze and manipulate the information they are working with.

In arithmetic, even capabilities and odd capabilities are capabilities which fulfill specific balance relations, as for taking added substance inverses. They are significant in numerous areas of numerical examination, particularly the hypothesis of force series and Fourier series. They are named for the equality of the powers of the power capabilities which fulfill each condition: the capability f(x)=x^{n} is an even capability assuming n is an even number, and it is an odd capability on the off chance that n is an odd whole number.

Where is Required even and odd functions

Even and odd functions are used in a variety of fields, including mathematics, physics, engineering, computer science, economics, and statistics. Some specific applications where even and odd functions are required include:

Fourier analysis: Even and odd functions are used in Fourier analysis to decompose a given function into its constituent frequencies.
Signal processing: Even and odd functions are used in signal processing to represent and manipulate signals, such as audio and video signals.
Control theory: Even and odd functions are used in control theory to design controllers that can stabilize and control systems.
Quantum mechanics: Even and odd functions are used in quantum mechanics to describe the wavefunctions of particles, which exhibit symmetry or anti-symmetry.
Electromagnetism: Even and odd functions are used in electromagnetism to describe electric and magnetic fields that exhibit symmetry or anti-symmetry.
Time series analysis: Even and odd functions are used in time series analysis to model and analyze data that exhibit periodicity or symmetry.
Spectral analysis: Even and odd functions are used in spectral analysis to analyze the frequency content of a given signal or system.

In summary, even and odd functions are required in many areas where functions, signals, or data are analyzed or manipulated. They play a fundamental role in many mathematical and scientific fields, and are essential for understanding and solving many real-world problems.

How is Required even and odd functions

Even and odd functions are required in various ways depending on the field of study or application. Here are some examples of how even and odd functions are used:

In mathematics, even and odd functions are used to describe functions that have special symmetry properties. This is useful for analyzing the properties of functions, such as their integrals, derivatives, and Fourier series.
In physics and engineering, even and odd functions are used to describe physical systems that exhibit symmetry or anti-symmetry. For example, the electric field of a charged particle has odd symmetry, while the potential energy of a spring has even symmetry.
In signal processing, even and odd functions are used to analyze and manipulate signals. This is useful for applications such as audio and video processing, image analysis, and digital communications.
In control theory, even and odd functions are used to design controllers that can stabilize and control systems. This is useful for applications such as robotics, aerospace, and automotive control.
In quantum mechanics, even and odd functions are used to describe the wavefunctions of particles. This is useful for understanding the properties of atoms, molecules, and other quantum systems.
In economics and finance, even and odd functions are used to analyze time series data, such as stock prices, economic indicators, and financial instruments.

In summary, even and odd functions are required in various fields and applications to describe symmetry properties of functions, analyze and manipulate signals, control systems, and understand quantum systems and time series data.

Parity of zero

In math, zero is a considerably number. As such, its equality — the nature of a whole number being even or odd — is even. This can be effortlessly confirmed in light of the meaning of “even”: it is a number various of 2, explicitly 0 × 2. Thus, zero offers every one of the properties that portray even numbers: for instance, 0 is adjoined on the two sides by odd numbers, any decimal number has similar equality as its last digit — in this way, since 10 is even, 0 will be even, and on the off chance that y is even, y + x has a similar equality as x — and 0 + x generally have a similar equality.

Zero additionally squeezes into the examples framed by other even numbers. The equality rules of number-crunching, like even − even = even, expect 0 to be even. Zero is the added substance character component of the gathering of even whole numbers, and it is the beginning case from which other even normal numbers are recursively characterized. Uses of this recursion from diagram hypothesis to computational calculation depend on zero being even. In addition to the fact that 0 is distinct by 2, it is separable by each force of 2, which is applicable to the twofold numeral framework utilized by PCs. In this sense, 0 is the “most significantly” number of all.

Among the overall population, the equality of zero can be a wellspring of disarray. In response time tests, a great many people are more slow to distinguish 0 as even than 2, 4, 6, or 8. Some teachers[who?] — and a few kids in math classes — imagine that zero is odd, or both even and odd, or not one or the other. Scientists in arithmetic training suggest that these confusions can become learning valuable open doors. Concentrating on correspondences like 0 × 2 = 0 can address understudies’ questions about calling 0 a number and involving it in math. Class conversations can lead understudies to see the value in the essential standards of numerical thinking, like the significance of definitions. Assessing the equality of this outstanding number is an early illustration of an unavoidable subject in science: the deliberation of a recognizable idea to a new setting.

White paper on even and odd functions

Here is a brief white paper on even and odd functions:

Introduction: Even and odd functions are two types of functions that exhibit special symmetry properties. These functions are used in various fields such as mathematics, physics, engineering, and signal processing. Understanding the properties of even and odd functions is essential for analyzing and manipulating signals, controlling systems, and understanding quantum systems and time series data.

Even Functions: An even function is a function that exhibits symmetry about the y-axis. This means that if you reflect the graph of the function across the y-axis, you get the same graph. In mathematical terms, an even function f(x) satisfies the property f(-x) = f(x) for all values of x. Examples of even functions include x^2, cos(x), and cosh(x).

Odd Functions: An odd function is a function that exhibits symmetry about the origin. This means that if you reflect the graph of the function across the origin, you get the same graph. In mathematical terms, an odd function f(x) satisfies the property f(-x) = -f(x) for all values of x. Examples of odd functions include x^3, sin(x), and sinh(x).

Properties of Even and Odd Functions:

The product of two even functions is an even function.
The product of two odd functions is an even function.
The product of an even function and an odd function is an odd function.
The sum of an even function and an odd function is neither even nor odd.
The derivative of an even function is an odd function.
The derivative of an odd function is an even function.
The integral of an even function over a symmetric interval is twice the integral over half the interval.
The integral of an odd function over a symmetric interval is zero.

Applications of Even and Odd Functions:

Fourier Series: Even and odd functions are used in Fourier series to represent periodic functions as a sum of sine and cosine functions.
Signal Processing: Even and odd functions are used in signal processing to analyze and manipulate signals, such as audio and video signals.
Control Theory: Even and odd functions are used in control theory to design controllers that can stabilize and control systems.
Quantum Mechanics: Even and odd functions are used in quantum mechanics to describe the wavefunctions of particles, which exhibit symmetry or anti-symmetry.
Time Series Analysis: Even and odd functions are used in time series analysis to model and analyze data that exhibit periodicity or symmetry.

Conclusion: Even and odd functions are fundamental concepts in mathematics and science. They exhibit special symmetry properties that are useful for analyzing and manipulating signals, controlling systems, and understanding quantum systems and time series data. The properties and applications of even and odd functions are relevant in many fields and can help researchers and engineers better understand and solve real-world problems.