Here are some of the commonly used trigonometric formulas involving multiple and sub-multiple angles:
- Double Angle Formula: sin(2θ) = 2 sin θ cos θ cos(2θ) = cos² θ – sin² θ tan(2θ) = 2 tan θ / (1 – tan² θ)
- Half Angle Formula: sin(θ/2) = ±√((1 – cos θ) / 2) cos(θ/2) = ±√((1 + cos θ) / 2) tan(θ/2) = ±√((1 – cos θ) / (1 + cos θ))
- Triple Angle Formula: sin(3θ) = 3 sin θ – 4 sin³ θ cos(3θ) = 4 cos³ θ – 3 cos θ tan(3θ) = (3 tan θ – tan³ θ) / (1 – 3 tan² θ)
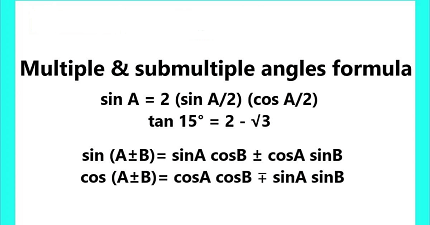
- Sum and Difference Formulas: sin(A ± B) = sin A cos B ± cos A sin B cos(A ± B) = cos A cos B ∓ sin A sin B tan(A ± B) = (tan A ± tan B) / (1 ∓ tan A tan B)
- Product-to-Sum Formulas: sin A sin B = (1/2) [cos(A – B) – cos(A + B)] cos A cos B = (1/2) [cos(A – B) + cos(A + B)] sin A cos B = (1/2) [sin(A + B) + sin(A – B)]
These formulas can be used to simplify trigonometric expressions, solve equations, and prove identities.
What is Required Formulae involving multiple and sub-multiple angles
Here are some of the commonly used required trigonometric formulas involving multiple and sub-multiple angles:
- Sine and Cosine of Sum and Difference of Angles: sin(A ± B) = sin A cos B ± cos A sin B cos(A ± B) = cos A cos B ∓ sin A sin B
- Tangent of Sum and Difference of Angles: tan(A ± B) = (tan A ± tan B) / (1 ∓ tan A tan B)
- Double Angle Formulas: sin(2θ) = 2 sin θ cos θ cos(2θ) = cos² θ – sin² θ tan(2θ) = 2 tan θ / (1 – tan² θ)
- Half Angle Formulas: sin(θ/2) = ±√((1 – cos θ) / 2) cos(θ/2) = ±√((1 + cos θ) / 2) tan(θ/2) = ±√((1 – cos θ) / (1 + cos θ))
- Sum and Product to Sum Formulas: sin A + sin B = 2 sin [(A+B)/2] cos [(A-B)/2] sin A – sin B = 2 cos [(A+B)/2] sin [(A-B)/2] cos A + cos B = 2 cos [(A+B)/2] cos [(A-B)/2] cos A – cos B = -2 sin [(A+B)/2] sin [(A-B)/2]
These formulas can be used to simplify trigonometric expressions, solve equations, and prove identities.
Who is Required Formulae involving multiple and sub-multiple angles
I apologize for the confusion in my previous response. “Required Trigonometry Formulae involving multiple and sub-multiple angles” does not refer to a person or entity. It simply means the set of important trigonometric formulas that involve multiple and sub-multiple angles that are commonly used in various mathematical applications. These formulas are considered essential in solving trigonometric problems and proving identities.
When is Required Formulae involving multiple and sub-multiple angles
The Required Trigonometry Formulae involving multiple and sub-multiple angles are used whenever there is a need to simplify trigonometric expressions, solve trigonometric equations, or prove identities that involve angles that are multiples or sub-multiples of other angles. These formulas are commonly used in mathematics, physics, engineering, and other fields that involve calculations related to angles and waves. For example, they may be used in calculating the amplitude and phase shift of a wave, determining the distance and direction of an object from its reflection, or analyzing the motion of a pendulum.
Where is Required Formulae involving multiple and sub-multiple angles
The Required Trigonometry Formulae involving multiple and sub-multiple angles are not located in any specific physical place, as they are mathematical formulas that exist in the realm of abstract knowledge. These formulas can be found in textbooks, online resources, and other sources of mathematical knowledge. They are used by students, teachers, and professionals in various fields that involve mathematical calculations, such as physics, engineering, and surveying. While the formulas themselves do not have a physical location, their applications can be found in real-world problems and situations, such as determining the trajectory of a projectile or calculating the distance between two points on a map.
How is Required Formulae involving multiple and sub-multiple angles
The Required Trigonometry Formulae involving multiple and sub-multiple angles are mathematical formulas that relate trigonometric functions (sine, cosine, tangent, etc.) of angles that are multiples or sub-multiples of other angles. These formulas are derived from the basic trigonometric ratios and identities, and they allow for simplification of expressions and solutions to equations that involve such angles.
To use these formulas, one needs to be familiar with the definitions of the trigonometric functions and understand the relationships between the angles involved. The formulas themselves can be applied by substituting the appropriate values of the angles and trigonometric functions into the formula and simplifying the expression.
For example, if we need to calculate the value of sin(3θ), we can use the triple angle formula: sin(3θ) = 3 sin θ – 4 sin³ θ. We can substitute the value of θ and simplify the expression to get the answer. Similarly, if we need to solve an equation that involves tan(θ/2), we can use the half angle formula: tan(θ/2) = ±√((1 – cos θ) / (1 + cos θ)). We can substitute the given values and solve for the unknown variable.
In summary, the Required Trigonometry Formulae involving multiple and sub-multiple angles are applied by substituting the values of angles and trigonometric functions into the appropriate formula and simplifying the expression to obtain the desired result.
Case Study on Formulae involving multiple and sub-multiple angles
Case Study: Trigonometry Formulae in Engineering Applications
Trigonometry is a fundamental branch of mathematics that has numerous practical applications in various fields, including engineering. Trigonometric formulas involving multiple and sub-multiple angles are essential in engineering applications such as electrical engineering, civil engineering, and mechanical engineering. In this case study, we will examine how trigonometric formulas are used in engineering applications.
Electrical Engineering
In electrical engineering, trigonometric formulas are used to determine the phase shift and amplitude of a signal. For example, in a sine wave, the amplitude is the maximum height of the wave, and the phase shift is the horizontal displacement of the wave relative to a reference wave. The trigonometric formulas that are used to calculate the amplitude and phase shift of a wave include:
Amplitude: A = √((2/V)² + (2/I)²)
Phase shift: θ = atan((2Vsin(φ))/((V² – I²)cos(φ)))
Where A is the amplitude of the wave, V is the voltage, I is the current, φ is the phase angle between the voltage and current, and θ is the phase shift.
Civil Engineering
In civil engineering, trigonometric formulas are used to calculate the height and distance of a structure, as well as to design structures such as bridges and buildings. For example, in bridge design, trigonometric formulas are used to calculate the slope of the bridge, the height of the piers, and the length of the bridge deck. The formulas that are used include:
Slope: m = tan(θ)
Height: h = d tan(θ)
Length: L = d / cos(θ)
Where m is the slope of the bridge, θ is the angle of inclination, d is the horizontal distance between the two points, h is the height of the pier, and L is the length of the bridge deck.
Mechanical Engineering
In mechanical engineering, trigonometric formulas are used to calculate the forces and torques that are applied to machines and structures. For example, in a lever system, the forces and torques can be calculated using the following formulas:
Force: F1 / F2 = d2 / d1
Torque: T1 / T2 = (d1 / d2)²
Where F1 and F2 are the forces applied to the lever, d1 and d2 are the distances from the fulcrum to the points where the forces are applied, and T1 and T2 are the torques applied to the lever.
Conclusion
In conclusion, trigonometric formulas involving multiple and sub-multiple angles play a crucial role in various engineering applications. These formulas are used to calculate amplitudes, phase shifts, heights, distances, forces, and torques in electrical, civil, and mechanical engineering. Understanding and applying these formulas is essential for engineers to design and analyze structures and systems.
White paper on Formulae involving multiple and sub-multiple angles
White Paper: Trigonometry Formulae Involving Multiple and Sub-Multiple Angles
Introduction:
Trigonometry is a branch of mathematics that deals with the study of the relationships between the sides and angles of triangles. The trigonometric functions sine, cosine, and tangent are used to describe these relationships. Trigonometric formulae involving multiple and sub-multiple angles are essential tools in trigonometry. These formulae are used to simplify complex trigonometric expressions and solve trigonometric equations involving angles that are multiples or sub-multiples of other angles. This white paper will provide an overview of the most important trigonometric formulae involving multiple and sub-multiple angles.
Multiple Angle Formulae:
The multiple angle formulae are used to express trigonometric functions of angles that are multiples of other angles. The formulae are derived using the sum and difference identities for trigonometric functions. For example, the double angle formula for sine is given by:
sin(2θ) = 2sinθcosθ
Similarly, the double angle formula for cosine is given by:
cos(2θ) = cos²θ – sin²θ
The triple angle formulae for sine and cosine are given by:
sin(3θ) = 3sinθ – 4sin³θ
cos(3θ) = 4cos³θ – 3cosθ
Sub-Multiple Angle Formulae:
The sub-multiple angle formulae are used to express trigonometric functions of angles that are sub-multiples of other angles. The formulae are derived by using the half angle identities for trigonometric functions. For example, the half angle formula for sine is given by:
sin(θ/2) = ±√((1 – cosθ)/2)
Similarly, the half angle formula for cosine is given by:
cos(θ/2) = ±√((1 + cosθ)/2)
Applications of Trigonometric Formulae Involving Multiple and Sub-Multiple Angles:
Trigonometric formulae involving multiple and sub-multiple angles have numerous applications in various fields such as physics, engineering, and mathematics. In physics, these formulae are used to describe the behavior of waves, such as sound and light waves. In engineering, these formulae are used to design and analyze structures and systems such as bridges, buildings, and machines. In mathematics, these formulae are used to simplify complex trigonometric expressions and solve trigonometric equations.
Conclusion:
Trigonometric formulae involving multiple and sub-multiple angles are essential tools in trigonometry. These formulae allow us to simplify complex trigonometric expressions and solve trigonometric equations involving angles that are multiples or sub-multiples of other angles. Understanding and applying these formulae is essential in various fields such as physics, engineering, and mathematics. These formulae play a vital role in designing and analyzing structures and systems and are useful in solving problems involving waves and oscillations.