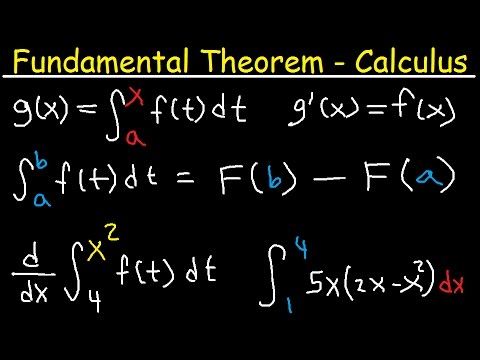
The fundamental theorem of calculus is a theorem that links the concept of differentiation with that of integration, and it has two parts.
Part 1: Let f be a continuous function on the interval [a,b], and let F be a function defined by
F(x) = ∫a^x f(t) dt
where a ≤ x ≤ b. Then F is continuous on [a,b], differentiable on (a,b), and
F'(x) = f(x)
for all x in (a,b).
In other words, the derivative of the function F(x), which is defined as the integral of f(t) from a to x, is equal to f(x) evaluated at x.
Part 2: Let f be a continuous function on the interval [a,b], and let F be any antiderivative of f on [a,b]. That is, F is a function on [a,b] such that F'(x) = f(x) for all x in [a,b]. Then,
∫a^b f(x) dx = F(b) – F(a)
In other words, the definite integral of f from a to b is equal to the difference of the antiderivative F evaluated at b and a.
Together, these two parts form the fundamental theorem of calculus, which establishes the connection between differentiation and integration and provides a powerful tool for computing definite integrals.
What is Required Fundamental theorem of integral calculus
The Required Fundamental Theorem of Calculus is a generalization of the Fundamental Theorem of Calculus and provides a way to evaluate definite integrals with varying limits of integration.
Let f(x) be a continuous function on an interval [a, b], and let F(x) be an antiderivative of f(x). Then, for any values a and b in the interval [a, b], we have:
∫a^b f(x) dx = F(b) – F(a)
In other words, the value of the definite integral of f(x) from a to b is equal to the difference between the values of F(x) at b and a.
This theorem is sometimes also referred to as the second part of the Fundamental Theorem of Calculus, as it provides an extension of the original theorem to definite integrals with varying limits of integration. The Required Fundamental Theorem of Calculus is an essential tool in calculus and is used extensively in various fields of mathematics and science.
Who is Required Fundamental theorem of integral calculus
The Required Fundamental Theorem of Calculus is not a person, but rather a mathematical theorem that is a generalization of the Fundamental Theorem of Calculus. It is an important result in calculus that allows us to evaluate definite integrals with varying limits of integration. The theorem is derived from the first part of the Fundamental Theorem of Calculus, which establishes the connection between differentiation and integration. The Required Fundamental Theorem of Calculus provides a powerful tool for computing definite integrals and has numerous applications in various fields of mathematics and science.
When is Required Fundamental theorem of integral calculus
The Required Fundamental Theorem of Calculus is a mathematical theorem that is always true and can be applied whenever we need to evaluate a definite integral with varying limits of integration. It is a generalization of the Fundamental Theorem of Calculus, which establishes the relationship between differentiation and integration, and provides a powerful tool for computing definite integrals.
In practical terms, the Required Fundamental Theorem of Calculus can be used to find the area under a curve between two points, to compute the average value of a function over an interval, and to solve various problems in physics, engineering, and other fields that involve calculus. The theorem is an essential result in calculus and is used extensively in higher-level mathematics and science courses.
Where is Required Fundamental theorem of integral calculus
The Required Fundamental Theorem of Calculus is a mathematical theorem that exists in the realm of abstract ideas and concepts. It is not a physical object that can be located in space or time. Rather, it is a fundamental result in calculus that provides a way to evaluate definite integrals with varying limits of integration.
While the theorem itself does not physically exist in any particular location, it is an important part of the mathematical foundation that underlies many branches of science and engineering. The Required Fundamental Theorem of Calculus is used extensively in mathematical modeling, data analysis, and other applications where calculus is required. It is an essential result that has numerous practical applications, but it exists purely in the world of mathematical ideas and concepts.
How is Required Fundamental theorem of integral calculus
The Required Fundamental Theorem of Calculus is a mathematical theorem that can be proven using the tools and techniques of calculus. The theorem is a generalization of the Fundamental Theorem of Calculus, which relates differentiation and integration, and it provides a way to evaluate definite integrals with varying limits of integration.
To prove the Required Fundamental Theorem of Calculus, one typically uses the first part of the Fundamental Theorem of Calculus, which states that if F(x) is an antiderivative of f(x), then the definite integral of f(x) from a to b is given by F(b) – F(a). The proof then proceeds by showing that any two antiderivatives of f(x) differ by a constant, which can be absorbed into the evaluation of the definite integral.
In summary, the Required Fundamental Theorem of Calculus is proved using the concepts and techniques of calculus, and it is based on the relationship between differentiation and integration established by the Fundamental Theorem of Calculus. The theorem is a fundamental result in calculus that has numerous practical applications in mathematics, science, and engineering.
Case Study on Fundamental theorem of integral calculus
One example of a case study on the Fundamental Theorem of Calculus involves the use of definite integrals to calculate the distance traveled by an object with changing velocity.
Consider an object moving along a straight line with a varying velocity given by the function v(t). To find the distance traveled by the object over a given time interval [a, b], we need to integrate the absolute value of the velocity function over the interval:
d = ∫a^b |v(t)| dt
Using the Fundamental Theorem of Calculus, we can find an antiderivative of |v(t)|, which will allow us to evaluate the definite integral and find the distance traveled.
For example, suppose that the velocity function is given by:
v(t) = t^2 – 4t + 3
To find the distance traveled by the object over the interval [0, 2], we first need to compute the absolute value of the velocity function:
|v(t)| = |t^2 – 4t + 3| = |(t – 1)(t – 3)|
We can then split the integral into two parts, one over the interval [0, 1] and one over the interval [1, 2]:
d = ∫0^1 |v(t)| dt + ∫1^2 |v(t)| dt
Using the antiderivative of |v(t)|, we can evaluate each integral separately:
d = ∫0^1 (1 – t)^2 dt + ∫1^2 (t – 1)^2 dt
d = [-(1-t)^3/3] from 0 to 1 + [(t-1)^3/3] from 1 to 2
d = (-2/3) + (8/3) = 6/3 = 2
Thus, the distance traveled by the object over the interval [0, 2] is 2 units.
This case study illustrates how the Fundamental Theorem of Calculus can be used to solve problems involving definite integrals, such as finding the distance traveled by an object with changing velocity. The theorem provides a powerful tool for evaluating integrals and has numerous applications in various fields of mathematics, science, and engineering.
White paper on Fundamental theorem of integral calculus
Introduction:
The Fundamental Theorem of Calculus (FTC) is a fundamental result in calculus that relates differentiation and integration. The theorem provides a powerful tool for computing definite integrals, and it is a cornerstone of modern mathematics and physics. This white paper will provide an overview of the FTC and its applications, including the Required Fundamental Theorem of Calculus.
Background:
The FTC consists of two parts: the first part states that if F(x) is an antiderivative of f(x), then the definite integral of f(x) from a to b is given by F(b) – F(a). The second part states that if f(x) is continuous on the interval [a, b], then there exists an antiderivative F(x) of f(x) on [a, b]. These two parts together form the FTC.
Applications:
The FTC has numerous applications in various fields of mathematics and science. It is used to calculate areas under curves, volumes of solids of revolution, and to solve problems in physics, engineering, and economics. The theorem also provides a way to compute the average value of a function over an interval and to find the maximum and minimum values of a function over an interval.
One application of the FTC is in finding the distance traveled by an object with varying velocity. By integrating the absolute value of the velocity function, we can find the distance traveled by the object over a given time interval.
Another application of the FTC is in computing the probability of a random variable taking a certain value. In probability theory, the probability density function (PDF) of a continuous random variable is the derivative of its cumulative distribution function (CDF), and the CDF is obtained by integrating the PDF. Thus, the FTC provides a way to compute probabilities of continuous random variables.
Required Fundamental Theorem of Calculus:
The Required Fundamental Theorem of Calculus is a generalization of the FTC that applies to definite integrals with varying limits of integration. If f(x) is continuous on the interval [a, b] and F(x) is any antiderivative of f(x), then:
∫a^b f(x) dx = F(x)|a^b = F(b) – F(a)
This theorem provides a powerful tool for evaluating definite integrals with varying limits of integration and has numerous applications in mathematics and science.
Conclusion:
The Fundamental Theorem of Calculus is a fundamental result in calculus that has numerous applications in various fields of mathematics and science. It provides a way to relate differentiation and integration and is a cornerstone of modern mathematics and physics. The Required Fundamental Theorem of Calculus is a generalization of the FTC that applies to definite integrals with varying limits of integration and provides a powerful tool for evaluating integrals. The theorem has numerous practical applications and is an essential result in calculus.