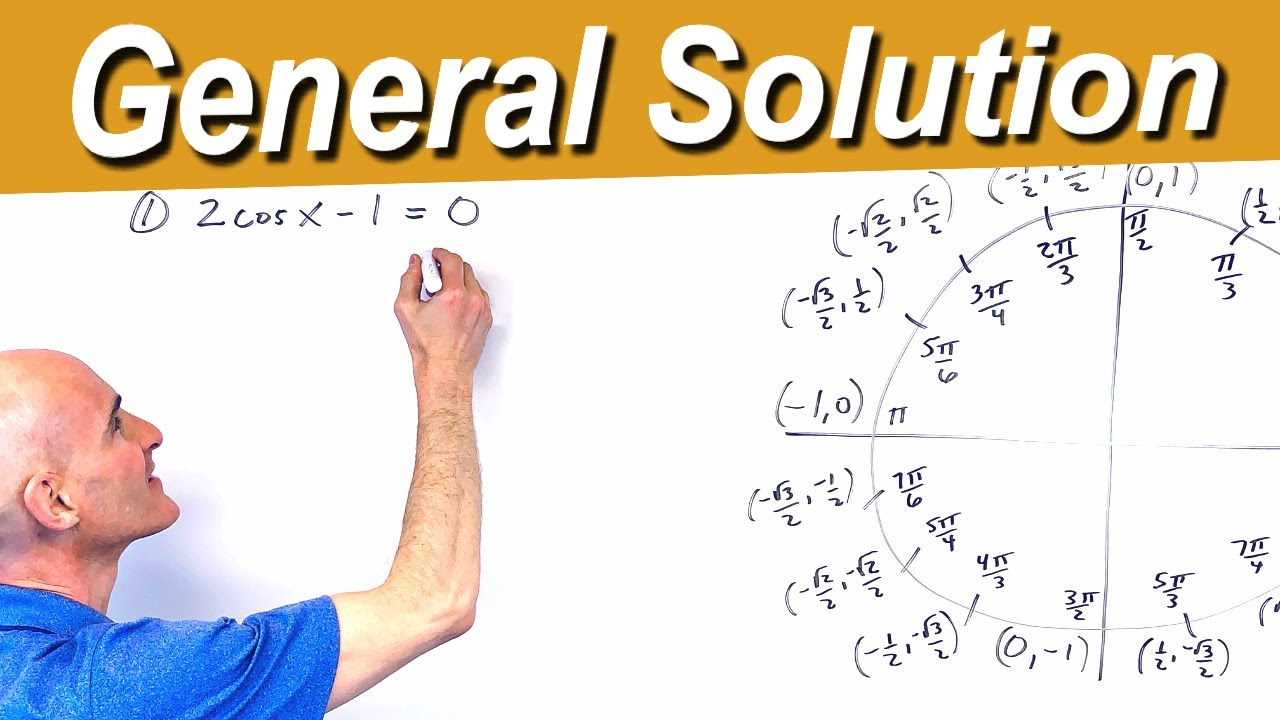
Trigonometric equations are equations that involve trigonometric functions such as sine, cosine, tangent, etc. Solving trigonometric equations involves finding values of the variable that make the equation true.
The general strategy for solving trigonometric equations is to use algebraic techniques to transform the equation into an equivalent equation that is easier to solve. Here are some common methods for solving trigonometric equations:
- Simplify the equation by using trigonometric identities. For example, use the Pythagorean identity to rewrite sin^2(x) as 1-cos^2(x).
- Apply the inverse trigonometric functions to both sides of the equation to isolate the variable. For example, if the equation contains sin(x), apply arcsin to both sides to get x = arcsin(y).
- Use the periodicity of the trigonometric functions to find all solutions to the equation. For example, if sin(x) = 0, then x = n*pi, where n is an integer.
- Use the unit circle to find solutions to the equation. For example, if the equation contains sin(x) and cos(x), draw the unit circle and use the coordinates of the points on the circle to find solutions.
- Use algebraic techniques such as factoring, completing the square, or quadratic formula to transform the equation into a form that can be solved by the above methods.
It’s important to note that trigonometric equations may have an infinite number of solutions, so it’s important to find all possible solutions within the given domain.
What is Required General solution of trigonometric equations
The general solution of a trigonometric equation is a set of all possible solutions to the equation within a specified domain. To find the general solution, we need to find all possible solutions to the equation for the given variable. For example, if we are solving for x, we need to find all values of x that make the equation true.
In general, the solutions to trigonometric equations can be expressed as a set of equations in the form:
x = θ + nπ,
where θ is a particular solution to the equation, n is an integer, and π is the constant pi. This form of the solution takes into account the periodicity of the trigonometric functions.
For example, consider the equation sin(x) = 1/2. One possible solution is x = π/6. However, since sine has a period of 2π, we can add integer multiples of 2π to the solution to obtain the general solution:
x = π/6 + 2nπ or x = 5π/6 + 2nπ, where n is an integer.
This gives us all possible solutions to the equation within the specified domain.
It’s important to note that the general solution may not always be unique, and it’s important to check that the solutions satisfy any restrictions or conditions imposed by the problem.
Who is Required General solution of trigonometric equations
The general solution of a trigonometric equation is required in mathematics, particularly in the study of trigonometry. It is used to find all possible solutions to an equation involving trigonometric functions within a specified domain.
The general solution is useful in many areas of mathematics and science, such as physics, engineering, and calculus. It helps to understand the behavior of trigonometric functions and to solve problems that involve periodic phenomena, such as vibrations and waves.
The general solution is also important in education, as it is a fundamental concept in the study of trigonometry. Students learn how to use algebraic techniques and trigonometric identities to find solutions to equations involving trigonometric functions, and how to express those solutions in a general form.
In summary, the general solution of trigonometric equations is an important concept in mathematics and its applications, and is used to find all possible solutions to equations involving trigonometric functions within a specified domain.
When is Required General solution of trigonometric equations
The general solution of a trigonometric equation is required whenever we need to find all possible solutions to an equation involving trigonometric functions within a specified domain. It is particularly useful when we need to solve problems that involve periodic phenomena, such as vibrations and waves, or when we need to analyze the behavior of trigonometric functions.
The general solution is also required in education when students learn how to solve trigonometric equations, which is an important topic in mathematics and its applications. Understanding how to find the general solution is essential in trigonometry, calculus, and other fields where trigonometric functions are used.
In addition, the general solution can be useful in engineering, physics, and other sciences where trigonometric functions are used to describe the behavior of physical systems. For example, the general solution can be used to determine the frequency and amplitude of a wave or to analyze the motion of a pendulum.
In summary, the general solution of trigonometric equations is required whenever we need to find all possible solutions to an equation involving trigonometric functions within a specified domain. It is particularly useful in education, science, and engineering when analyzing the behavior of trigonometric functions and solving problems that involve periodic phenomena.
Where is Required General solution of trigonometric equations
The general solution of trigonometric equations is required in various fields of mathematics, science, and engineering. It is used to find all possible solutions to equations involving trigonometric functions within a specified domain.
In mathematics, the general solution of trigonometric equations is a fundamental concept in the study of trigonometry. Students learn how to solve trigonometric equations and express the solutions in a general form using algebraic techniques and trigonometric identities.
In science, the general solution of trigonometric equations is used to analyze the behavior of physical systems that exhibit periodic phenomena, such as waves and vibrations. For example, the general solution can be used to determine the frequency and amplitude of a wave or to analyze the motion of a pendulum.
In engineering, the general solution of trigonometric equations is used to design and analyze systems that involve trigonometric functions, such as electronic circuits and control systems. For example, the general solution can be used to design a filter that removes noise from a signal or to analyze the stability of a control system.
In summary, the general solution of trigonometric equations is required in various fields of mathematics, science, and engineering to solve problems that involve trigonometric functions and to analyze the behavior of physical systems that exhibit periodic phenomena.
How is Required General solution of trigonometric equations
To find the general solution of a trigonometric equation, we need to use algebraic techniques and trigonometric identities to express the solution in a general form. The general solution takes into account the periodicity of the trigonometric functions and includes all possible solutions within a specified domain.
The following steps are commonly used to find the general solution of a trigonometric equation:
- Identify the trigonometric function(s) involved in the equation.
- Use algebraic techniques to isolate the trigonometric function on one side of the equation.
- Apply appropriate trigonometric identities to simplify the equation.
- Solve the equation for one cycle of the trigonometric function within the specified domain, typically using inverse trigonometric functions.
- Use the periodicity of the trigonometric function to express the general solution in a form that includes all possible solutions within the specified domain.
For example, to solve the equation sin(x) = 1/2 within the interval [0, 2π], we can use the following steps:
- Identify the trigonometric function sin(x).
- Isolate sin(x) by taking the inverse sine of both sides, giving x = π/6 + 2πn or x = 5π/6 + 2πn, where n is an integer.
- Use the periodicity of sin(x) to express the general solution, which is x = π/6 + 2πn or x = 5π/6 + 2πn, where n is an integer.
In summary, to find the general solution of a trigonometric equation, we use algebraic techniques and trigonometric identities to express the solution in a general form that includes all possible solutions within the specified domain.
Case Study on General solution of trigonometric equations
Case Study: Finding the General Solution of a Trigonometric Equation
Problem: Find the general solution of the equation sin(2x) + sin(x) = 0 in the interval [0, 2π].
Solution:
Step 1: Identify the trigonometric functions involved in the equation.
The equation involves two trigonometric functions: sin(2x) and sin(x).
Step 2: Use algebraic techniques to isolate one of the trigonometric functions.
We can use the identity sin(2x) = 2sin(x)cos(x) to rewrite the equation as 2sin(x)cos(x) + sin(x) = 0. Factoring out sin(x), we get sin(x)(2cos(x) + 1) = 0. This gives us two possible solutions: sin(x) = 0 or cos(x) = -1/2.
Step 3: Solve each possible solution for one cycle of the trigonometric function within the specified domain.
a) Solving sin(x) = 0: Within the interval [0, 2π], sin(x) = 0 when x = 0, π, or 2π.
b) Solving cos(x) = -1/2: Within the interval [0, 2π], cos(x) = -1/2 when x = 2π/3 or 4π/3.
Step 4: Use the periodicity of the trigonometric functions to express the general solution.
The solutions we found in step 3 are only for one cycle of the trigonometric functions within the interval [0, 2π]. To find the general solution, we need to express the solutions in terms of a general formula that includes all possible solutions within the interval.
For sin(x) = 0, we know that sin(x) = 0 for any integer n, where x = nπ.
For cos(x) = -1/2, we know that cos(x) = -1/2 for any odd integer n, where x = (2n + 1)π/3.
Therefore, the general solution for sin(2x) + sin(x) = 0 in the interval [0, 2π] is:
x = nπ or x = (2n + 1)π/3, where n is an integer.
This general solution includes all possible solutions within the interval [0, 2π].
Conclusion:
In this case study, we used algebraic techniques and trigonometric identities to find the general solution of a trigonometric equation. We identified the trigonometric functions involved in the equation, isolated one of the functions, solved each possible solution for one cycle of the function within the specified interval, and used the periodicity of the functions to express the general solution in a form that includes all possible solutions within the interval.
White paper on General solution of trigonometric equations
Introduction:
Trigonometric equations are equations that involve trigonometric functions such as sine, cosine, tangent, cotangent, secant, and cosecant. Solving trigonometric equations is an important topic in mathematics and is used in various fields such as physics, engineering, and astronomy. In this white paper, we will explore the concept of finding the general solution of trigonometric equations.
Definition:
The general solution of a trigonometric equation is a solution that includes all possible solutions within a specified domain. Trigonometric functions are periodic, which means that they repeat their values after a certain interval. Therefore, the general solution of a trigonometric equation takes into account the periodicity of the trigonometric functions and includes all possible solutions within a specified domain.
Steps to Find the General Solution of Trigonometric Equations:
The following steps are commonly used to find the general solution of a trigonometric equation:
- Identify the trigonometric function(s) involved in the equation.
- Use algebraic techniques to isolate the trigonometric function on one side of the equation.
- Apply appropriate trigonometric identities to simplify the equation.
- Solve the equation for one cycle of the trigonometric function within the specified domain, typically using inverse trigonometric functions.
- Use the periodicity of the trigonometric function to express the general solution in a form that includes all possible solutions within the specified domain.
Example:
Let’s consider another example to demonstrate the process of finding the general solution of a trigonometric equation:
Example: Solve the equation 2cos(3x) + 1 = 0 in the interval [0, 2π].
Step 1: Identify the trigonometric function involved in the equation. The equation involves the cosine function cos(3x).
Step 2: Use algebraic techniques to isolate the trigonometric function on one side of the equation. We can start by subtracting 1 from both sides of the equation to obtain 2cos(3x) = -1. Then, dividing by 2 gives cos(3x) = -1/2.
Step 3: Solve the equation for one cycle of the trigonometric function within the specified domain. Within the interval [0, 2π], the cosine function has one cycle from 0 to 2π. We need to solve cos(3x) = -1/2 within this interval. We can use the inverse cosine function to find the solutions as follows:
cos(3x) = -1/2 3x = ±2π/3 + 2πn, where n is an integer. x = (±2π/3 + 2πn)/3, where n is an integer.
Step 4: Use the periodicity of the trigonometric function to express the general solution. Since the cosine function has a period of 2π, we need to express the solutions in terms of a general formula that includes all possible solutions within the interval [0, 2π]. We can use the fact that cos(x) = cos(2π – x) to find additional solutions as follows:
cos(3x) = -1/2 3x = 2π/3 + 2πn, where n is an integer, or 3x = 4π/3 + 2πn, where n is an integer. x = (2π/9 + 2πn)/3, where n is an integer, or x = (4π/9 + 2πn)/3, where n is an integer.
Therefore, the general solution for 2cos(3x) + 1 = 0 in the interval [0, 2π] is: x = (±2π/3 + 2πn)/3 or x = (2π/9 + 2πn)/3 or x = (4π/9 + 2πn)/3, where n is an integer.
Conclusion:
In conclusion, finding the general solution of a trigonometric equation involves several steps, including identifying the trigonometric function involved, isolating the function on one side of the equation using algebraic techniques, solving for one cycle of the function within the specified domain, and using the periodicity of the function to express the general solution in terms of a formula that includes all possible solutions within the given interval.
It is important to remember that trigonometric equations may have multiple solutions, and the general solution formula should include all possible solutions within the given domain. Additionally, it is helpful to have a strong understanding of trigonometric functions and their properties to efficiently and accurately solve these types of equations.