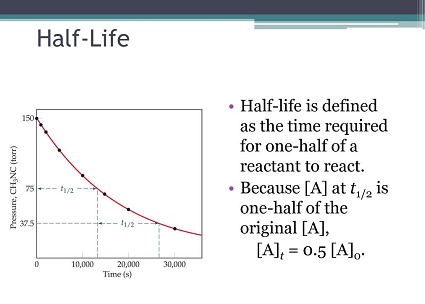
In physics, half-life refers to the time it takes for half of the atoms in a particular sample of a radioactive substance to decay. This decay process is random and unpredictable, so the half-life of a substance cannot be predicted with certainty. However, for any given substance, the half-life is a constant value, meaning that if you have a sample of the substance with a certain number of atoms, you can predict how long it will take for half of those atoms to decay.

Half-life is commonly used in fields such as nuclear physics, radiocarbon dating, and medical imaging. It is also used in pharmacology to describe the rate at which a drug is eliminated from the body, and in environmental science to describe the rate at which pollutants break down in the environment.

The concept of half-life is related to exponential decay, which is a type of mathematical function that describes how a quantity decreases over time. Specifically, the amount of a radioactive substance remaining after a certain amount of time can be described using an exponential function with a decay constant equal to the reciprocal of the substance’s half-life.

What is Required Half-life

The required half-life refers to the amount of time it takes for a particular substance to decrease in potency or concentration to a level that is considered safe or acceptable for a specific application. The required half-life can vary depending on the specific substance and the context in which it is being used.

For example, in the context of radiation therapy for cancer, the required half-life of a radioactive isotope used in the treatment will depend on the specific type and location of the cancer being treated, as well as the sensitivity of surrounding healthy tissue to radiation. The goal is to deliver a high enough dose of radiation to the cancer cells to kill them, while minimizing damage to healthy tissue.

Similarly, in the context of environmental cleanup of hazardous waste sites, the required half-life of a pollutant will depend on factors such as its toxicity, persistence in the environment, and potential for bioaccumulation in the food chain. The goal is to reduce the concentration of the pollutant to a level that is safe for human health and the environment over a certain period of time.

In summary, the required half-life is a critical parameter that must be considered when designing treatments or cleanup strategies for various substances, and it can vary depending on the specific context and application.

When is Required Half-life

The term “Required Half-life” refers to a specific parameter related to the decay of certain substances, and is not associated with a particular time or date. The required half-life is determined by the specific context and application of the substance in question, and represents the amount of time it takes for the substance to decay to a level that is considered safe or acceptable for that application.

For example, in nuclear physics, the required half-life of a radioactive isotope is important for determining its potential use in various applications, such as nuclear power generation or medical imaging. In pharmacology, the required half-life of a drug is an important factor in determining the optimal dosage and dosing frequency to achieve the desired therapeutic effect.

Therefore, the concept of required half-life is not associated with a specific time or date, but rather is a critical parameter to be considered when designing treatments or cleanup strategies for various substances, and can vary depending on the specific context and application.

Where is Required Half-life

The concept of “Required Half-life” is not associated with a physical location, but rather is a scientific term used to describe a key parameter for various substances and applications.

The required half-life refers to the amount of time it takes for a particular substance to decrease in potency or concentration to a level that is considered safe or acceptable for a specific application. This parameter is critical in various fields such as pharmacology, nuclear physics, environmental science, and others.

For example, in pharmacology, the required half-life of a drug is an important factor in determining the optimal dosage and dosing frequency to achieve the desired therapeutic effect. In environmental science, the required half-life of a pollutant is critical in determining the appropriate cleanup strategy for contaminated sites. In nuclear physics, the required half-life of a radioactive isotope is used to determine its potential use in various applications such as nuclear power generation or medical imaging.

Therefore, the concept of required half-life is not associated with a physical location, but rather is a critical parameter that must be considered when designing treatments or cleanup strategies for various substances, and can vary depending on the specific context and application.

How is Required Half-life

The required half-life is determined through scientific experimentation and analysis. In general, the half-life of a substance can be measured by observing the decay of a sample of the substance over time, and calculating the amount of time it takes for half of the sample to decay.

The required half-life, however, is not a fixed value for a substance but rather is determined by the specific context and application of the substance. For example, the required half-life of a radioactive isotope used in medical imaging may be shorter than the required half-life of a radioactive isotope used in nuclear power generation. Similarly, the required half-life of a drug used to treat a chronic condition may be longer than the required half-life of a drug used to treat an acute condition.

Determining the required half-life involves considering various factors such as the substance’s toxicity, its effectiveness in achieving the desired outcome, and the potential for adverse effects on the environment or human health. The required half-life is critical in determining the optimal dosage, dosing frequency, or cleanup strategy for the substance in question.

In summary, the required half-life is determined through scientific experimentation and analysis, and is influenced by the specific context and application of the substance in question. It is a critical parameter that must be considered when designing treatments or cleanup strategies for various substances.

Production of Half-life

The half-life of a substance is a natural property determined by its inherent characteristics and cannot be artificially produced or manipulated.

The half-life of a substance is the amount of time it takes for half of the atoms in a sample to undergo radioactive decay or other forms of decay. This property is determined by the physical and chemical properties of the substance, including the nature of the radioactive decay process, the energy released during decay, and the interactions of the substance with other particles and fields in its environment.

While the half-life of a substance cannot be artificially produced or manipulated, it can be measured and used for various applications in science and medicine. For example, in nuclear medicine, radioactive isotopes with specific half-lives are used to diagnose and treat various medical conditions. In environmental science, the half-life of pollutants is important in determining the appropriate cleanup strategy for contaminated sites.

In summary, the half-life of a substance is a natural property that cannot be artificially produced or manipulated. It is a critical parameter that can be measured and used for various applications in science and medicine.

Case Study on Half-life

A case study on half-life could focus on the use of radioactive isotopes in medicine, specifically in the diagnosis and treatment of cancer. One example is the use of the radioactive isotope iodine-131 in the treatment of thyroid cancer.

Iodine-131 has a half-life of approximately 8 days, which means that half of the radioactive atoms will decay every 8 days. This makes it a suitable choice for treating thyroid cancer, as the isotope can be delivered directly to the thyroid gland through ingestion or injection, and its short half-life allows for quick clearance from the body after treatment.

During the treatment, the patient ingests or is injected with iodine-131, which is selectively taken up by thyroid cells due to the gland’s need for iodine. The radiation emitted by the isotope then destroys the cancerous thyroid cells, while leaving the surrounding healthy tissue relatively unaffected.

The short half-life of iodine-131 means that the patient’s exposure to radiation is limited and the isotope is cleared from the body within a few weeks. This reduces the risk of long-term side effects, such as the development of other cancers.

The use of iodine-131 in the treatment of thyroid cancer is just one example of how the half-life of a substance can be a critical parameter in its use for medical applications. It is important to carefully consider the half-life of any substance used in medical treatments, in order to minimize the potential risks and maximize the therapeutic benefits for patients.

White paper on Half-life

Half-life is a term used to describe the amount of time it takes for a substance to decrease in potency or concentration to half of its initial value. This term is commonly used in various scientific fields, including pharmacology, nuclear physics, and environmental science. In this white paper, we will explore the concept of half-life in more detail, including its definition, calculation, and practical applications.

Definition of Half-life Half-life refers to the amount of time it takes for half of the atoms or molecules in a sample of a substance to undergo radioactive decay or other forms of decay. The concept of half-life is based on the exponential decay model, which assumes that the rate of decay of a substance is proportional to the amount of the substance remaining at any given time. In other words, the larger the amount of a substance remaining, the faster it will decay.

Calculation of Half-life The half-life of a substance can be calculated using the following formula:

t1/2 = (ln2) / λ

where t1/2 is the half-life, ln2 is the natural logarithm of 2 (approximately 0.693), and λ is the decay constant of the substance. The decay constant is a measure of the rate at which the substance undergoes decay, and is specific to each substance.

Applications of Half-life The concept of half-life has numerous applications in science and medicine. In pharmacology, the half-life of a drug is an important parameter in determining the optimal dosage and dosing frequency to achieve the desired therapeutic effect. The half-life of a drug also determines the length of time it stays in the body, which is important for determining the potential for side effects and drug interactions.

In nuclear physics, the half-life of a radioactive isotope is used to determine its potential use in various applications such as nuclear power generation or medical imaging. The half-life of a radioactive isotope also determines the length of time it must be stored or disposed of safely, as it can remain hazardous for many years.

In environmental science, the half-life of pollutants is important in determining the appropriate cleanup strategy for contaminated sites. The half-life of pollutants also determines the potential for long-term environmental effects and human health risks.

Conclusion The concept of half-life is a critical parameter in various scientific fields and applications, and its calculation and measurement are essential for understanding the behavior of substances over time. The half-life of a substance is determined by its inherent physical and chemical properties, and cannot be artificially produced or manipulated. Understanding the half-life of substances is important for ensuring their safe and effective use in medicine, industry, and the environment.