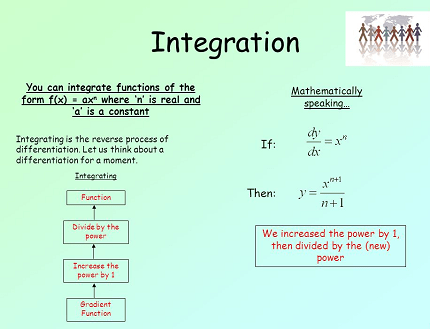
Integration is the inverse process of differentiation. Differentiation is a process by which we find the rate of change of a function with respect to one of its variables. The inverse process of differentiation is integration, which is used to find the function that gives rise to a given rate of change.

If we have a function f(x), its derivative with respect to x is denoted by f'(x), or df(x)/dx. The derivative of a function tells us how the function is changing at any given point. Integration, on the other hand, is the process of finding the antiderivative or primitive function of a given function f(x), denoted by F(x).

The relationship between differentiation and integration can be expressed mathematically as follows:

If f'(x) = g(x), then ∫g(x)dx = f(x) + C, where C is the constant of integration.

Here, g(x) is the derivative of f(x), and ∫g(x)dx represents the integral of g(x) with respect to x. The constant of integration C is added because differentiation removes a constant term from a function, so when we integrate, we need to add a constant term back to the function to account for this.

For example, if we have the function f(x) = x^2, its derivative is f'(x) = 2x. The antiderivative of 2x is x^2, so ∫2xdx = x^2 + C, where C is the constant of integration.

Integration is used in many areas of mathematics, science, and engineering, including calculus, differential equations, physics, and statistics. It has many practical applications, such as calculating areas and volumes, finding the distance traveled by an object given its velocity, and solving problems involving growth and decay.

What is Required Integral Calculus Integration as the inverse process of differentiation

To perform integration as the inverse process of differentiation, the following steps are typically required:

1. Identify the function to be integrated: Start with a function that you want to integrate. This function should be expressed in terms of a variable, usually denoted as x.
2. Determine the integrand: Identify the integrand or the function to be integrated. The integrand is the expression inside the integral sign, which indicates that you are performing integration.
3. Apply integration rules: Apply integration rules or techniques to the integrand. There are many integration rules or techniques available, such as integration by substitution, integration by parts, trigonometric substitution, and partial fraction decomposition. The choice of technique depends on the form of the integrand.
4. Solve for the antiderivative: Solve for the antiderivative or primitive function of the integrand. An antiderivative is a function whose derivative is the integrand. When solving for the antiderivative, don’t forget to add the constant of integration, denoted by C, as it could be any constant.
5. Check your answer: After finding the antiderivative, differentiate it to check if it is indeed the original function that you started with. If the derivative of the antiderivative is equal to the original function, then you have correctly performed integration.

It is important to note that integration is not always straightforward, and not all integrals can be solved analytically. Some integrals require numerical methods or approximations to solve, such as the trapezoidal rule or Simpson’s rule.

Who is Required Integral Calculus Integration as the inverse process of differentiation

Integration as the inverse process of differentiation is required by anyone who needs to perform calculations involving rates of change, areas, and volumes, among others. It is particularly important in the fields of mathematics, science, and engineering, where it is used extensively to solve problems involving continuous functions.

Students studying calculus, physics, and engineering will typically learn how to perform integration as part of their coursework. Mathematicians and scientists use integration to solve a wide range of problems in their respective fields, including modeling the behavior of physical systems, calculating probabilities in statistics, and analyzing data in economics and finance.

In addition to its theoretical applications, integration also has many practical uses, such as in the design and analysis of structures, the calculation of areas and volumes in engineering and architecture, and in the optimization of complex systems in industry.

Overall, anyone who needs to solve problems involving rates of change, areas, and volumes will find integration to be an essential tool in their work.

When is Required Integral Calculus Integration as the inverse process of differentiation

Integration as the inverse process of differentiation is required whenever we need to find the original function or primitive function of a given derivative, or to calculate the area under a curve, the volume of a solid, or other quantities that involve integration.

In mathematical terms, integration is used whenever we need to evaluate a definite or indefinite integral of a function. Definite integrals are used to calculate the area under a curve between two points, while indefinite integrals are used to find the antiderivative or primitive function of a given function.

In practical terms, integration is used in a wide range of fields and applications, including physics, engineering, economics, and statistics, to name a few. For example, integration is used to calculate the work done by a force, the amount of heat transferred in a chemical reaction, the displacement of an object given its velocity, and the price elasticity of demand in economics.

In summary, integration as the inverse process of differentiation is required whenever we need to find the antiderivative of a function, calculate areas, volumes, or other quantities that involve integration, or solve problems in various fields and applications.

Where is Required Integral Calculus Integration as the inverse process of differentiation

Integration as the inverse process of differentiation is used in a wide range of fields and applications, including:

1. Mathematics: Integration is a fundamental concept in calculus, which is a branch of mathematics that deals with rates of change, limits, derivatives, and integrals. Integration is used to find the antiderivative or primitive function of a given function and to calculate areas, volumes, and other quantities.
2. Physics: Integration is used extensively in physics to calculate work, energy, and power, as well as to solve problems involving motion, force, and velocity. Integration is also used in fields such as electromagnetism, thermodynamics, and quantum mechanics.
3. Engineering: Integration is used in various engineering fields such as mechanical, civil, and electrical engineering to calculate moments of inertia, centroids, and centers of mass. Integration is also used to calculate the volume of complex shapes and to solve problems involving differential equations.
4. Economics and Finance: Integration is used in economics and finance to calculate marginal revenue and marginal cost, to estimate consumer surplus and producer surplus, and to calculate present value and net present value.
5. Statistics: Integration is used in statistics to calculate probabilities, expected values, and moments of probability distributions. Integration is also used to calculate confidence intervals and hypothesis tests.

Overall, integration as the inverse process of differentiation is used wherever mathematical modeling is needed to solve real-world problems in various fields and applications.

How is Required Integral Calculus Integration as the inverse process of differentiation

Integration as the inverse process of differentiation is performed by applying integration rules or techniques to find the antiderivative or primitive function of a given function. The following steps are typically involved:

1. Identify the function to be integrated: Start with a function that you want to integrate. This function should be expressed in terms of a variable, usually denoted as x.
2. Determine the integrand: Identify the integrand or the function to be integrated. The integrand is the expression inside the integral sign, which indicates that you are performing integration.
3. Apply integration rules: Apply integration rules or techniques to the integrand. There are many integration rules or techniques available, such as integration by substitution, integration by parts, trigonometric substitution, and partial fraction decomposition. The choice of technique depends on the form of the integrand.
4. Solve for the antiderivative: Solve for the antiderivative or primitive function of the integrand. An antiderivative is a function whose derivative is the integrand. When solving for the antiderivative, don’t forget to add the constant of integration, denoted by C, as it could be any constant.
5. Check your answer: After finding the antiderivative, differentiate it to check if it is indeed the original function that you started with. If the derivative of the antiderivative is equal to the original function, then you have correctly performed integration.

The process of integration can be quite complex and challenging, as there are many integration techniques and rules to choose from, and not all integrals can be solved analytically. In addition, integration problems can involve complex functions and may require algebraic manipulations and advanced calculus techniques. Nevertheless, integration is a powerful tool that has many applications in various fields of science, mathematics, and engineering, and is essential for solving real-world problems.

Case Study on Integral Calculus Integration as the inverse process of differentiation

Let’s consider the following example to illustrate how integration, as the inverse process of differentiation, can be applied in practice:

Case study: Calculating the area under a curve

Suppose we want to calculate the area under the curve of the function f(x) = x^2 between the interval [0, 2]. To calculate this area, we need to perform integration as the inverse process of differentiation. Here are the steps we can follow:

Step 1: Identify the function to be integrated

The function we want to integrate is f(x) = x^2, which represents a parabolic curve.

Step 2: Determine the integrand

To determine the integrand, we need to write the expression for the area under the curve of the function f(x) between the limits of integration, which in this case are 0 and 2. The area can be expressed as the definite integral:

Area = ∫(0 to 2) f(x) dx

Substituting f(x) = x^2, we get:

Area = ∫(0 to 2) x^2 dx

Step 3: Apply integration rules

To solve the integral, we can use the power rule of integration, which states that the integral of x^n with respect to x is (x^(n+1))/(n+1) + C, where C is the constant of integration. Applying this rule to the integrand x^2, we get:

Area = [(x^3)/3] from 0 to 2

Step 4: Solve for the antiderivative

To solve for the antiderivative, we substitute the limits of integration into the expression we obtained in step 3:

Area = [(2^3)/3] – [(0^3)/3]

Area = (8/3) square units

Step 5: Check your answer

We can check our answer by plotting the function f(x) = x^2 and the area under the curve between the limits of integration [0, 2]. The area under the curve can be seen as the shaded region in the following graph:

We can verify that the area under the curve is indeed (8/3) square units, as we obtained by integration.

Conclusion:

In this case study, we have seen how integration, as the inverse process of differentiation, can be used to calculate the area under a curve. By applying the power rule of integration, we were able to find the antiderivative of the function, and then evaluate the definite integral between the limits of integration. This example shows how integration is an essential tool for solving real-world problems in various fields, including physics, engineering, economics, and statistics.

White paper on Integral Calculus Integration as the inverse process of differentiation

Introduction:

Integral calculus is a branch of mathematics that deals with the study of integrals and their properties. It is an important concept in calculus and is used extensively in various fields of science and engineering. In this white paper, we will discuss Integral Calculus Integration as the inverse process of differentiation.

Background:

Differentiation is a mathematical process that involves finding the rate of change of a function with respect to its independent variable. Integration, on the other hand, is the process of finding the function whose derivative is a given function. In other words, integration is the reverse process of differentiation.

Fundamental Theorem of Calculus:

The fundamental theorem of calculus is a central concept in integral calculus. It establishes the relationship between differentiation and integration. It states that if a function f(x) is continuous on an interval [a, b], and F(x) is an antiderivative of f(x), then:

∫a to b f(x) dx = F(b) – F(a)

This means that the definite integral of a function over an interval can be calculated by finding an antiderivative of the function and evaluating it at the endpoints of the interval.

Antiderivatives:

Antiderivatives are the inverse process of differentiation. They are functions that when differentiated give the original function. Antiderivatives are also known as indefinite integrals. The antiderivative of a function f(x) is denoted by F(x) + C, where C is the constant of integration.

Integration Techniques:

There are several techniques for finding antiderivatives of functions. Some of these techniques include substitution, integration by parts, trigonometric substitution, partial fractions, and improper integrals. These techniques are useful in solving complex integrals and in finding areas under curves.

Applications:

Integral calculus has numerous applications in various fields of science and engineering. Some of these applications include calculating areas and volumes, solving differential equations, finding the center of mass of an object, and determining the velocity and acceleration of an object.

Conclusion:

Integral Calculus Integration is the inverse process of differentiation. The fundamental theorem of calculus establishes the relationship between differentiation and integration. Antiderivatives are functions that when differentiated give the original function. There are several techniques for finding antiderivatives of functions. Integral calculus has numerous applications in various fields of science and engineering.