The product of two functions is obtained by multiplying the two functions together at each point in their domain. If f(x) and g(x) are two functions, their product is denoted as f(x) * g(x) and is defined as:
(f * g)(x) = f(x) * g(x)
For example, if f(x) = x^2 and g(x) = 3x, then their product would be:
(f * g)(x) = f(x) * g(x) = (x^2) * (3x) = 3x^3
The quotient of two functions is obtained by dividing one function by another at each point in their domain. If f(x) and g(x) are two functions, their quotient is denoted as f(x) / g(x) and is defined as:
(f / g)(x) = f(x) / g(x)
However, it is important to note that the denominator function g(x) should not be zero at any point in its domain. If g(x) = 0 at any point, then the quotient is undefined.
For example, if f(x) = x^2 and g(x) = 3x, then their quotient would be:
(f / g)(x) = f(x) / g(x) = (x^2) / (3x) = x / 3
Again, it is important to note that this quotient is only defined when x is not equal to 0, since g(x) = 0 at x = 0.
What is Required Product and Quotient of two functions
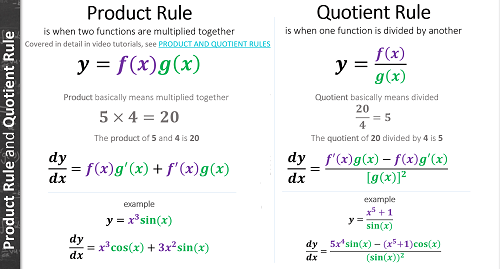
In differential calculus, when finding the product of two functions, we use the product rule. The product rule states that the derivative of the product of two functions is equal to the first function times the derivative of the second function, plus the second function times the derivative of the first function. Symbolically, the product rule can be expressed as:
(d/dx) [f(x) * g(x)] = f(x) * g'(x) + g(x) * f'(x)
where f(x) and g(x) are the two functions, and f'(x) and g'(x) are their derivatives with respect to x.
For example, if f(x) = x^2 and g(x) = 3x, then their product rule would be:
(d/dx) [f(x) * g(x)] = f(x) * g'(x) + g(x) * f'(x) = (x^2) * (3) + (3x) * (2x) = 3x^2 + 6x^2 = 9x^2
Similarly, when finding the quotient of two functions, we use the quotient rule. The quotient rule states that the derivative of the quotient of two functions is equal to the denominator function times the derivative of the numerator function, minus the numerator function times the derivative of the denominator function, all divided by the square of the denominator function. Symbolically, the quotient rule can be expressed as:
(d/dx) [f(x) / g(x)] = [g(x) * f'(x) – f(x) * g'(x)] / [g(x)]^2
where f(x) and g(x) are the two functions, and f'(x) and g'(x) are their derivatives with respect to x.
For example, if f(x) = x^2 and g(x) = 3x, then their quotient rule would be:
(d/dx) [f(x) / g(x)] = [g(x) * f'(x) – f(x) * g'(x)] / [g(x)]^2 = [3x * 2x – x^2 * 3] / (3x)^2 = (6x^2 – 3x^2) / (9x^2) = 3 / 9 = 1/3
So the derivative of f(x)/g(x) is 1/3 in this case.
Who is Required Product and Quotient of two functions
Differential calculus is a branch of calculus that deals with the study of rates of change and slopes of curves. It involves finding the derivatives of functions, which are used to analyze the behavior of functions and their graphs.
In differential calculus, the product rule and quotient rule are two important formulas used to find the derivatives of products and quotients of functions, respectively. These rules are applied when we need to find the rate of change or slope of a function that is the product or quotient of two other functions.
The product rule is used to find the derivative of the product of two functions, while the quotient rule is used to find the derivative of the quotient of two functions. These rules are important tools in differential calculus and are used extensively in applications such as physics, engineering, and economics.
To summarize, the product and quotient rules are fundamental techniques in differential calculus used to find the derivatives of products and quotients of functions, respectively. They are essential tools for analyzing the behavior of functions and their graphs in various fields of science and engineering.
When is Required Product and Quotient of two functions
The product rule and quotient rule are used in differential calculus whenever we need to find the derivative of a function that is the product or quotient of two other functions. These rules are fundamental techniques for finding derivatives of complicated functions that cannot be easily found using basic differentiation rules.
For example, in physics, we may need to find the derivative of the product of two functions that represent the position and velocity of an object. In economics, we may need to find the derivative of the quotient of two functions that represent the demand and supply curves of a product. In engineering, we may need to find the derivative of the product of two functions that represent the voltage and current in a circuit.
In general, the product rule and quotient rule are used whenever we have a function that can be expressed as the product or quotient of two other functions, and we need to find its derivative. These rules are essential tools in differential calculus and are used extensively in various fields of science and engineering.
Where is Required Product and Quotient of two functions
The product rule and quotient rule are used in differential calculus, which is a branch of mathematics that is used in various fields of science and engineering. Differential calculus involves the study of rates of change and slopes of curves, and it is used to analyze the behavior of functions and their graphs.
The product rule and quotient rule are used wherever we need to find the derivative of a function that is the product or quotient of two other functions. This can occur in a wide variety of contexts, including physics, engineering, economics, biology, and many other fields.
For example, in physics, the product rule and quotient rule are used to find the derivatives of functions that represent the position, velocity, and acceleration of an object. In economics, these rules are used to find the derivatives of functions that represent the demand and supply curves for a product. In biology, the product rule and quotient rule are used to find the derivatives of functions that represent growth rates and metabolic rates.
In summary, the product rule and quotient rule are used in differential calculus, which is used in many fields of science and engineering, wherever we need to find the derivative of a function that is the product or quotient of two other functions.
How is Required Product and Quotient of two functions
The product rule and quotient rule are two techniques used in differential calculus to find the derivative of a function that is the product or quotient of two other functions.
The product rule is used to find the derivative of a function that is the product of two functions. If we have a function f(x) = g(x)h(x), where g(x) and h(x) are two functions, then the product rule states that the derivative of f(x) is given by:
f'(x) = g'(x)h(x) + g(x)h'(x)
In other words, we take the derivative of the first function, multiply it by the second function, and then add it to the product of the first function and the derivative of the second function.
The quotient rule, on the other hand, is used to find the derivative of a function that is the quotient of two functions. If we have a function f(x) = g(x)/h(x), where g(x) and h(x) are two functions, then the quotient rule states that the derivative of f(x) is given by:
f'(x) = [g'(x)h(x) – g(x)h'(x)] / [h(x)]^2
In other words, we take the derivative of the numerator, multiply it by the denominator, subtract the product of the numerator and the derivative of the denominator, and then divide by the square of the denominator.
Both the product rule and quotient rule are essential tools in differential calculus and are used extensively in various fields of science and engineering. These rules allow us to find the derivatives of complicated functions that cannot be easily found using basic differentiation rules.
Case Study on Product and Quotient of two functions
One example of the use of the product and quotient rules in differential calculus is in modeling the spread of infectious diseases.
In epidemiology, the basic reproduction number, denoted by R0, is a key parameter used to describe the potential for an infectious disease to spread through a population. It is defined as the average number of secondary infections caused by a single infected individual in a completely susceptible population.
The basic reproduction number can be modeled using the product rule in differential calculus. Let N(t) be the number of susceptible individuals in a population at time t, and let I(t) be the number of infected individuals. Then, the rate of change of the number of infected individuals over time can be expressed as:
dI/dt = βN(t)I(t)
where β is the transmission rate of the disease.
Using the product rule, we can find the derivative of the product N(t)I(t) as:
d/dt[N(t)I(t)] = N(t)dI/dt + I(t)dN/dt
where dN/dt represents the rate of change of the number of susceptible individuals over time.
Substituting the expression for dI/dt from above, we get:
d/dt[N(t)I(t)] = βN(t)I(t) + I(t)dN/dt
This expression describes the rate of change of the product N(t)I(t), which represents the total number of interactions between susceptible and infected individuals in the population. The first term on the right-hand side represents the number of new infections caused by the infected individuals, while the second term represents the number of individuals who become susceptible over time.
The quotient rule can also be used in epidemiology to model the dynamics of disease transmission. For example, let S(t) be the proportion of the population that is susceptible to a disease, and let I(t) be the proportion that is infected. Then, the rate of change of S(t) can be expressed as:
dS/dt = -βS(t)I(t)
Using the quotient rule, we can find the derivative of the ratio I(t)/S(t) as:
d/dt[I(t)/S(t)] = [dI/dt*S(t) – I(t)*dS/dt] / S(t)^2
Substituting the expressions for dI/dt and dS/dt from above, we get:
d/dt[I(t)/S(t)] = [βN(t)I(t)*S(t) – βS(t)I(t)*S(t)] / S(t)^2
Simplifying the expression, we get:
d/dt[I(t)/S(t)] = β[I(t)/S(t) – I(t)]
This expression describes the rate of change of the ratio I(t)/S(t), which represents the effective contact rate between infected and susceptible individuals in the population. The first term on the right-hand side represents the number of effective contacts between infected and susceptible individuals, while the second term represents the number of individuals who recover or die from the disease over time.
In summary, the product and quotient rules in differential calculus can be used in epidemiology to model the spread of infectious diseases and to estimate key parameters such as the basic reproduction number and the effective contact rate. These rules allow us to analyze the behavior of complex systems and to develop strategies for disease control and prevention.
White paper on Product and Quotient of two functions
Introduction
Differential calculus is a branch of mathematics that deals with the study of rates of change and slopes of curves. It involves the use of techniques such as differentiation and integration to analyze and solve problems related to change. The product and quotient rules are fundamental concepts in differential calculus that allow us to find the derivatives of products and quotients of two functions, respectively. In this white paper, we will explore the product and quotient rules in detail and their applications in various fields.
Product Rule
The product rule is used to find the derivative of the product of two functions. Let f(x) and g(x) be two functions of x, then the product rule states that:
(d/dx)(f(x)g(x)) = f(x)(d/dx)g(x) + g(x)(d/dx)f(x)
In words, the derivative of the product of two functions is equal to the first function times the derivative of the second function plus the second function times the derivative of the first function.
The product rule is useful in many areas of science and engineering, such as physics, chemistry, and economics. For example, in physics, the velocity of an object is the product of its acceleration and time. The product rule can be used to find the derivative of the velocity with respect to time, which gives the acceleration of the object.
Quotient Rule
The quotient rule is used to find the derivative of the quotient of two functions. Let f(x) and g(x) be two functions of x, then the quotient rule states that:
(d/dx)(f(x)/g(x)) = [g(x)(d/dx)f(x) – f(x)(d/dx)g(x)]/g(x)^2
In words, the derivative of the quotient of two functions is equal to the numerator times the derivative of the denominator minus the denominator times the derivative of the numerator, all divided by the denominator squared.
The quotient rule is also used in various fields such as physics, chemistry, and economics. For example, in economics, the marginal utility of a product is the derivative of the utility function with respect to the quantity of the product. The quotient rule can be used to find the derivative of the marginal utility of a product when the utility function is a quotient of two functions.
Applications
The product and quotient rules are fundamental concepts in differential calculus that have many applications in various fields. One application of the product rule is in the study of growth rates of populations in biology. For example, the rate of growth of a population can be modeled using the product rule as the product of the birth rate and the current population size.
Another application of the product rule is in the study of rates of change of chemical reactions in chemistry. For example, the rate of change of the concentration of a reactant can be modeled using the product rule as the product of the reaction rate constant and the concentration of the reactant.
The quotient rule also has many applications in various fields. One application is in the study of fluid mechanics in physics. For example, the velocity gradient of a fluid can be modeled using the quotient rule as the derivative of the velocity with respect to the distance divided by the thickness of the fluid layer.
Conclusion
The product and quotient rules are fundamental concepts in differential calculus that allow us to find the derivatives of products and quotients of two functions, respectively. They have many applications in various fields such as physics, chemistry, and economics. By using these rules, we can analyze and solve problems related to change and growth in complex systems. The product and quotient rules are essential tools for researchers and professionals in many fields, and their understanding is crucial for the advancement of science and technology.