Rationality refers to the ability to think logically, make sound judgments, and make decisions based on reason rather than emotions or impulses. It involves using critical thinking skills to analyze information, evaluate evidence, and draw conclusions based on facts and evidence.
In general, a rational person is someone who can separate their emotions from their decisions, considers different perspectives before making a decision, and makes choices that are consistent with their goals and values. Rationality is an important skill in many areas of life, including personal relationships, business, politics, and science. It allows people to make informed decisions, solve problems, and achieve their goals in a logical and efficient manner.
What is Required Rational
Required differential calculus in mathematics refers to the study of the derivative, which is a fundamental concept in calculus. The derivative measures the rate at which a function changes with respect to its input variable. In particular, it measures the instantaneous rate of change of the function at a given point.
In differential calculus, you will learn various techniques to find the derivative of a function, such as using the power rule, product rule, quotient rule, chain rule, and implicit differentiation. You will also learn how to use the derivative to find critical points, maxima and minima, and inflection points of a function.
Moreover, differential calculus plays a vital role in many fields, such as physics, engineering, economics, and statistics. It is used to model real-world phenomena and to optimize systems and processes. Understanding differential calculus is a crucial step for further study in calculus and many other advanced mathematical topics.
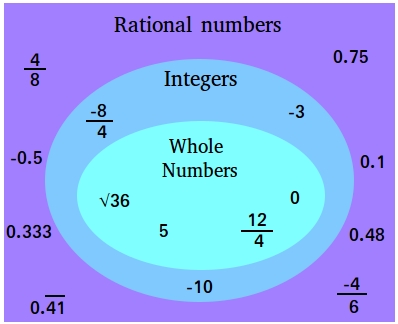
Rational number

In mathematics, a rational number is a number that can be expressed as the quotient or fraction 

A rational number is a real number. The real numbers that are rational are those whose decimal expansion either terminates after a finite number of digits (example: 3/4 = 0.75), or eventually begins to repeat the same finite sequence of digits over and over (example: 9/44 = 0.20454545…). This statement is true not only in base 10, but also in every other integer base, such as the binary and hexadecimal ones (see Repeating decimal § Extension to other bases).
A real number that is not rational is called irrational. Irrational numbers include the square root of 2 (2

Rational numbers can be formally defined as equivalence classes of pairs of integers (p, q) with q ≠ 0, using the equivalence relation defined as follows:
The fraction 
Rational numbers together with addition and multiplication form a field which contains the integers, and is contained in any field containing the integers. In other words, the field of rational numbers is a prime field, and a field has characteristic zero if and only if it contains the rational numbers as a subfield. Finite extensions of 

In mathematical analysis, the rational numbers form a dense subset of the real numbers. The real numbers can be constructed from the rational numbers by completion, using Cauchy sequences, Dedekind cuts, or infinite decimals (see Construction of the real numbers).
Rational (disambiguation)
Objective might allude to:
Objective number, a number that can be communicated as a proportion of two whole numbers
Objective mark of a logarithmic assortment, a point characterized over the levelheaded numbers
Objective capability, a capability that might be characterized as the remainder of two polynomials
Objective portion, an articulation worked from the numbers and a few factors by expansion, deduction, increase and division
Objective Programming, a product organization currently claimed by IBM
Tenberry Programming, previously Judicious Frameworks, an outdated American programming organization
Judicious AG, a German producer of food processors
Objective, stage name of Canadian hip-bounce craftsman Matt Brotzel
The Rationals, a previous American wild band
Judicious, a character order in the Keirsey Disposition Sorter
Rationalism



In way of thinking, logic is the epistemological view that “sees reason as the central source and trial of knowledge” or “any view interesting to reason as a wellspring of information or justification”. All the more officially, realism is characterized as a strategy or a hypothesis “in which the standard of truth isn’t tactile yet scholarly and deductive”.
In an old discussion, realism was against experimentation, where the pragmatists accepted that reality has a naturally coherent design. Along these lines, the pragmatists contended that specific bits of insight exist and that the mind can straightforwardly accept these bits of insight. In other words, pragmatists declared that specific reasonable standards exist in rationale, math, morals, and mysticism that are so essentially evident that denying them makes one fall into logical inconsistency. The realists had such a high trust in reason that exact confirmation and actual proof were viewed as superfluous to learn specific insights – all in all, “there are critical manners by which our ideas and information are acquired freely of sense experience”.
Various levels of accentuation on this strategy or hypothesis lead to a scope of pragmatist points of view, from the moderate position “that reason has priority over alternate approaches to procuring information” to the more outrageous place that reason is “the one of a kind way to knowledge”. Given a pre-current comprehension of reason, logic is indistinguishable from theory, the Socratic existence of request, or the zetetic (wary) clear understanding of power (open to the basic or fundamental reason for things as they appear to our feeling of conviction). In ongoing many years, Leo Strauss looked to restore “Traditional Political Logic” as a discipline that grasps the errand of thinking, not as basic, however as maieutic.
Rationality
Judiciousness is the nature of being directed by or in light of reasons. In such manner, an individual demonstrations normally on the off chance that they have a valid justification for what they do or a conviction is reasonable assuming that it depends serious areas of strength for on. This quality can apply to a capacity, as in sane creature, to a mental cycle, such as thinking, to mental states, like convictions and expectations, or to people who have these different types of levelheadedness. A thing that needs sanity is either arational, in the event that it is outside the space of levelheaded assessment, or silly, on the off chance that it has a place with this area however doesn’t satisfy its guidelines.
There are numerous conversations about the fundamental highlights shared by all types of levelheadedness. As indicated by reason-responsiveness accounts, to be sane is to be receptive to reasons. For instance, foreboding shadows are a justification behind taking an umbrella, which is the reason it is objective for a specialist to do as such accordingly. A significant adversary to this approach are intelligence based accounts, which characterize sanity as inward soundness among the specialist’s psychological states. Many guidelines of cognizance have been proposed in such manner, for instance, that one shouldn’t hold problematic convictions or that one ought to plan to follow through with something if one accepts that one ought to get it done. Objective based accounts describe levelheadedness corresponding to objectives, like gaining truth on account of hypothetical discernment. Internalists accept that soundness relies just upon the individual’s psyche. Externalists battle that outer variables may likewise be pertinent. Banters about the normativity of judiciousness concern whether or not one ought to continuously be levelheaded. A further conversation is whether sanity expects that all convictions are investigated without any preparation as opposed to confiding in previous convictions.
Different sorts of levelheadedness are examined in the scholastic writing. The most powerful differentiation is among hypothetical and pragmatic soundness. Hypothetical reasonableness concerns the sanity of convictions. Reasonable convictions depend on proof that upholds them. Useful levelheadedness relates essentially to activities. This incorporates specific mental states and occasions going before activities, similar to goals and choices. Now and again, the two can struggle, as when commonsense discernment expects that one embraces an unreasonable conviction. Another differentiation is between ideal soundness, which requests that normal specialists submit to every one of the regulations and ramifications of rationale, and limited levelheadedness, which considers that this isn’t generally imaginable since the computational force of the human psyche is excessively restricted. Most scholastic conversations center around the discernment of people. This differentiations with social or aggregate judiciousness, which relates to assemblages and their gathering convictions and choices.
Reasonableness is significant for tackling a wide range of issues to arrive at one’s objective proficiently. It is important for and talked about in many disciplines. In morals, one inquiry is whether one can be reasonable without being moral simultaneously. Brain research is keen on how mental cycles execute reasonableness. This additionally incorporates the investigation of disappointments to do as such, as on account of mental inclinations. Mental and conduct sciences ordinarily expect that individuals are reasonable to anticipate how they think and act. Rationale concentrates on the laws of right contentions. These regulations are exceptionally significant for the judiciousness of convictions. An extremely persuasive origination of functional objectivity is given in choice hypothesis: it expresses that a choice is reasonable on the off chance that the picked choice has the most noteworthy anticipated utility. Other applicable fields incorporate game hypothesis, Bayesianism, financial aspects, and man-made brainpower.
Case Study on Rational
Differential Calculus is a branch of mathematics that deals with the study of rates of change and slopes of curves. The term “rational” usually refers to a number or an expression that can be written as a ratio of two integers. In the context of Differential Calculus, rational functions play an important role in the study of calculus and its applications.
A rational function is a function of the form f(x) = p(x)/q(x), where p(x) and q(x) are polynomials in x, and q(x) is not identically equal to zero. The domain of a rational function is the set of all real numbers except those for which the denominator q(x) is equal to zero.
One important concept in Differential Calculus related to rational functions is the idea of limits. The limit of a function is the value that the function approaches as its input approaches a certain value. For example, consider the rational function f(x) = (x^2 – 1)/(x – 1). As x approaches 1, the denominator q(x) approaches 0, and the function f(x) becomes undefined. However, by factoring the numerator, we can simplify the function to f(x) = (x + 1), which is defined at x = 1. Therefore, the limit of f(x) as x approaches 1 is 2.
Another important concept in Differential Calculus related to rational functions is the derivative. The derivative of a function gives the rate at which the function is changing at a particular point. For a rational function f(x) = p(x)/q(x), the derivative can be computed using the quotient rule of differentiation. The quotient rule states that the derivative of f(x) is given by:
f'(x) = [q(x)*p'(x) – p(x)*q'(x)]/[q(x)]^2
where p'(x) and q'(x) are the derivatives of the polynomials p(x) and q(x), respectively.
For example, consider the rational function f(x) = (x^2 – 1)/(x – 1). Using the quotient rule, we can find the derivative of f(x) as:
f'(x) = [(x – 1)*2x – (x^2 – 1)*1]/[(x – 1)^2]
= (x^2 – 2x + 1)/[(x – 1)^2]
Simplifying the expression, we get:
f'(x) = (x – 1)/(x – 1)^2
= 1/(x – 1)
This derivative tells us the rate at which the function f(x) is changing at any given point. For example, when x = 2, the derivative is 1, which means that the function is increasing at a rate of 1 unit per unit change in x.
In conclusion, rational functions play an important role in the study of Differential Calculus. They provide important examples for the concepts of limits and derivatives, and they are frequently encountered in applications of calculus in physics, engineering, economics, and other fields.
White paper on Rational
Here is a white paper on Differential Calculus Rational:
Introduction Differential Calculus is a branch of mathematics that deals with the study of rates of change and slopes of curves. Rational functions play an important role in the study of calculus and its applications. In this paper, we will discuss the properties of rational functions and their applications in Differential Calculus.
Properties of Rational Functions A rational function is a function of the form f(x) = p(x)/q(x), where p(x) and q(x) are polynomials in x, and q(x) is not identically equal to zero. The domain of a rational function is the set of all real numbers except those for which the denominator q(x) is equal to zero.
One important property of rational functions is that they are continuous on their domain. This means that if a rational function is defined at a point c, then the limit of the function as x approaches c exists and is equal to the value of the function at c.
Another important property of rational functions is that they have vertical asymptotes at the zeros of the denominator q(x). A vertical asymptote is a vertical line that the graph of the function approaches but never touches. The location of the vertical asymptotes can be found by setting the denominator equal to zero and solving for x.
Applications of Rational Functions in Differential Calculus Limits: Rational functions play an important role in the computation of limits. In particular, if the limit of a rational function f(x) exists as x approaches a, then the value of the limit can be found by evaluating the function at a or by canceling any common factors in the numerator and denominator.
Derivatives: The derivative of a rational function can be found using the quotient rule of differentiation. The quotient rule states that the derivative of f(x) = p(x)/q(x) is given by:
f'(x) = [q(x)*p'(x) – p(x)*q'(x)]/[q(x)]^2
where p'(x) and q'(x) are the derivatives of the polynomials p(x) and q(x), respectively.
For example, consider the rational function f(x) = (x^2 – 1)/(x – 1). Using the quotient rule, we can find the derivative of f(x) as:
f'(x) = [(x – 1)*2x – (x^2 – 1)*1]/[(x – 1)^2]
= (x^2 – 2x + 1)/[(x – 1)^2]
Simplifying the expression, we get:
f'(x) = (x – 1)/(x – 1)^2
= 1/(x – 1)
This derivative tells us the rate at which the function f(x) is changing at any given point.
Optimization: Rational functions are frequently encountered in optimization problems. For example, suppose we want to find the maximum value of a rational function f(x) = p(x)/q(x) on some interval [a, b]. To find the maximum value, we first find the critical points of the function by setting the derivative equal to zero and solving for x. We then evaluate the function at the critical points and the endpoints of the interval, and the largest of these values is the maximum value of the function on the interval.
Conclusion In this paper, we have discussed the properties of rational functions and their applications in Differential Calculus. Rational functions are continuous on their domain and have vertical asymptotes at the zeros of the denominator. They are frequently encountered in the computation of limits, the computation of derivatives, and optimization problems.

