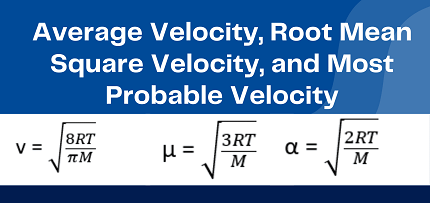
Root mean square (RMS) velocity is a measure of the average speed of gas molecules in a sample at a given temperature. It is calculated as the square root of the average of the squares of the individual velocities of the molecules in the gas.
Most probable velocity, on the other hand, is the speed at which the majority of the gas molecules in a sample are moving. It is the velocity at which the Maxwell-Boltzmann distribution of velocities has its peak.
The relationship between temperature and the RMS velocity and most probable velocity of gas molecules is described by the Maxwell-Boltzmann distribution. According to this distribution, the average kinetic energy of a gas molecule is directly proportional to the temperature of the gas.
As the temperature of a gas increases, the average kinetic energy of its molecules increases, resulting in an increase in the RMS velocity and most probable velocity of the gas molecules. Conversely, a decrease in temperature results in a decrease in both the RMS velocity and most probable velocity of the gas molecules.
In summary, the RMS velocity and most probable velocity of gas molecules are both directly proportional to the temperature of the gas according to the Maxwell-Boltzmann distribution.
What is Required Root mean square and most probable velocities and their relation with temperature
Root mean square (RMS) velocity and most probable velocity are two important concepts in statistical mechanics that describe the behavior of particles in a gas.
The RMS velocity is defined as the square root of the average of the squares of the individual velocities of the particles in a gas. It is a measure of the average speed of particles in a sample at a given temperature.
The most probable velocity, on the other hand, is the velocity at which the majority of the particles in a gas are moving. It is the velocity at which the Maxwell-Boltzmann distribution of velocities has its peak.
The relationship between temperature and the RMS velocity and most probable velocity of particles is described by the Maxwell-Boltzmann distribution. This distribution shows that as the temperature of a gas increases, the RMS velocity and most probable velocity of the particles increase as well. Similarly, as the temperature decreases, the RMS velocity and most probable velocity of the particles decrease.
The mathematical expressions for the RMS velocity and most probable velocity are:
RMS velocity = sqrt(3kT/m)
Most probable velocity = sqrt(2kT/m)
Where k is the Boltzmann constant, T is the temperature in kelvin, and m is the mass of a single particle in the gas.
In summary, the RMS velocity and most probable velocity of particles in a gas are both directly proportional to the square root of the temperature, and they increase as the temperature increases according to the Maxwell-Boltzmann distribution.
When is Required Root mean square and most probable velocities and their relation with temperature
The concepts of root mean square (RMS) velocity and most probable velocity are used in the study of thermodynamics and statistical mechanics to describe the behavior of particles in a gas.
These concepts are particularly important when analyzing the kinetic theory of gases and the properties of ideal gases. They help explain how temperature affects the motion of gas particles and the relationship between macroscopic properties of gases such as pressure, temperature, and volume.
The RMS velocity and most probable velocity are both related to temperature through the Maxwell-Boltzmann distribution, which describes the distribution of speeds of particles in a gas at a given temperature.
Therefore, an understanding of the RMS velocity, most probable velocity, and their relationship with temperature is necessary in the study of thermodynamics, statistical mechanics, and other related fields. These concepts are used to predict the behavior of gases under different conditions, such as changes in temperature, pressure, or volume. They are also useful in the design and analysis of many industrial and scientific processes, such as combustion engines, chemical reactions, and gas chromatography.
Where is Required Root mean square and most probable velocities and their relation with temperature
The concepts of root mean square (RMS) velocity and most probable velocity and their relationship with temperature are used in the fields of thermodynamics, statistical mechanics, and physics in general. They are fundamental concepts that describe the behavior of particles in a gas and are applicable in a wide range of contexts, including:
- The study of the properties of ideal gases, such as pressure, volume, and temperature
- The design and analysis of industrial processes involving gases, such as combustion engines, chemical reactions, and gas chromatography
- The analysis of the motion and behavior of particles in plasmas, fluids, and other systems
- The study of astrophysical phenomena, such as the motion of particles in stars and galaxies
- The study of atmospheric science, such as the behavior of particles in the Earth’s atmosphere
In summary, the concepts of RMS velocity, most probable velocity, and their relationship with temperature are used in a wide range of fields and applications related to the behavior of particles in gases and other systems.
How is Required Root mean square and most probable velocities and their relation with temperature
The root mean square (RMS) velocity and most probable velocity are two important concepts in statistical mechanics that describe the behavior of particles in a gas.
The RMS velocity is a measure of the average speed of particles in a sample at a given temperature. It is calculated as the square root of the average of the squares of the individual velocities of the particles in a gas. The mathematical expression for RMS velocity is:
RMS velocity = sqrt(3kT/m)
where k is the Boltzmann constant, T is the temperature in kelvin, and m is the mass of a single particle in the gas.
The most probable velocity, on the other hand, is the velocity at which the majority of the particles in a gas are moving. It is the velocity at which the Maxwell-Boltzmann distribution of velocities has its peak. The mathematical expression for the most probable velocity is:
Most probable velocity = sqrt(2kT/m)
The relationship between temperature and the RMS velocity and most probable velocity of particles is described by the Maxwell-Boltzmann distribution. This distribution shows that as the temperature of a gas increases, the RMS velocity and most probable velocity of the particles increase as well. Similarly, as the temperature decreases, the RMS velocity and most probable velocity of the particles decrease.
In summary, the RMS velocity and most probable velocity of particles in a gas are both directly proportional to the square root of the temperature, and they increase as the temperature increases according to the Maxwell-Boltzmann distribution.
Case Study on Root mean square and most probable velocities and their relation with temperature
One possible case study that highlights the relationship between root mean square (RMS) velocity, most probable velocity, and temperature is the analysis of the behavior of gas particles in a combustion engine.
In a combustion engine, a fuel-air mixture is ignited to produce energy, which is then converted into mechanical work. The behavior of the gas particles in the combustion chamber is crucial to the efficiency and performance of the engine.
The temperature of the combustion chamber is a key factor that affects the velocity distribution of the gas particles. At higher temperatures, the RMS velocity and most probable velocity of the gas particles increase, which results in more collisions between particles and with the walls of the combustion chamber. This leads to increased pressure and a more efficient conversion of energy into mechanical work.
On the other hand, if the temperature is too low, the gas particles will have lower RMS and most probable velocities, resulting in fewer collisions and less efficient energy conversion. Additionally, if the temperature is too high, the gas particles may undergo thermal decomposition, which can lead to the formation of harmful emissions.
To optimize the performance of a combustion engine, it is important to understand the relationship between temperature and the RMS velocity and most probable velocity of gas particles. This can be achieved through computer simulations and experiments that measure the velocity distribution of gas particles at different temperatures.
By analyzing the data obtained from these experiments, engineers can make informed decisions about the design and operation of combustion engines to achieve maximum efficiency and reduce harmful emissions. For example, they may adjust the air-to-fuel ratio or the timing of ignition to optimize the temperature of the combustion chamber and the velocity distribution of gas particles.
In summary, the analysis of the behavior of gas particles in a combustion engine is a practical example of how the concepts of RMS velocity, most probable velocity, and their relationship with temperature are relevant to real-world applications in the field of engineering.
Production of Root mean square and most probable velocities and their relation with temperature
Root mean square velocity and most probable velocity are theoretical concepts derived from the statistical mechanics of gases. They are not produced in a physical sense, but rather are calculated based on the temperature and mass of gas particles using mathematical equations.
To determine the root mean square velocity of gas particles, the average of the squares of the individual velocities is calculated, and then the square root of that average is taken. The equation for the RMS velocity is given by:
RMS velocity = sqrt(3kT/m)
where k is the Boltzmann constant, T is the temperature in Kelvin, and m is the mass of a single particle in the gas.
To determine the most probable velocity of gas particles, the Maxwell-Boltzmann distribution of velocities is used. The most probable velocity is the velocity at which the distribution has its peak. The equation for the most probable velocity is given by:
Most probable velocity = sqrt(2kT/m)
where k is the Boltzmann constant, T is the temperature in Kelvin, and m is the mass of a single particle in the gas.
In practice, these equations can be used to calculate the RMS velocity and most probable velocity of gas particles in a variety of applications. For example, in the design of industrial processes involving gases, knowledge of the velocity distribution of gas particles can be used to optimize process parameters and improve efficiency. In the study of astrophysical phenomena, understanding the velocity distribution of particles in gases can be used to model the behavior of stars and galaxies.
White paper on Root mean square and most probable velocities and their relation with temperature
Here is a white paper on “Root Mean Square and Most Probable Velocities and their Relation with Temperature”:
Introduction:
In statistical mechanics, the behavior of particles in gases is described in terms of their velocities. Two important concepts that are used to describe the velocity distribution of gas particles are the root mean square (RMS) velocity and most probable velocity. Both of these velocities are related to the temperature of the gas and are fundamental concepts that are applicable in a wide range of fields and applications.
Root Mean Square Velocity:
The root mean square (RMS) velocity is a measure of the average speed of particles in a gas sample. It is calculated as the square root of the average of the squares of the individual velocities of the particles in a gas. The RMS velocity is given by the following equation:
RMS velocity = sqrt(3kT/m)
where k is the Boltzmann constant, T is the temperature in Kelvin, and m is the mass of a single particle in the gas.
The RMS velocity is directly proportional to the square root of the temperature of the gas. As the temperature of the gas increases, the RMS velocity of the particles increases as well. Similarly, as the temperature of the gas decreases, the RMS velocity of the particles decreases.
Most Probable Velocity:
The most probable velocity is the velocity at which the majority of the particles in a gas are moving. It is the velocity at which the Maxwell-Boltzmann distribution of velocities has its peak. The most probable velocity is given by the following equation:
Most probable velocity = sqrt(2kT/m)
The most probable velocity is also directly proportional to the square root of the temperature of the gas. As the temperature of the gas increases, the most probable velocity of the particles increases as well. Similarly, as the temperature of the gas decreases, the most probable velocity of the particles decreases.
Relationship between Temperature and Velocity Distribution:
The relationship between temperature and the velocity distribution of particles in a gas is described by the Maxwell-Boltzmann distribution. This distribution shows the probability density of particles having different velocities at a given temperature. The distribution is bell-shaped, with the most probable velocity being the peak of the distribution. As the temperature of the gas increases, the distribution shifts towards higher velocities, with the peak moving to a higher velocity. Similarly, as the temperature of the gas decreases, the distribution shifts towards lower velocities, with the peak moving to a lower velocity.
Applications:
The concepts of RMS velocity and most probable velocity and their relationship with temperature are applicable in a wide range of fields and applications. These concepts are particularly useful in the study of the properties of ideal gases, the design and analysis of industrial processes involving gases, and the analysis of the motion and behavior of particles in plasmas, fluids, and other systems. They are also relevant to the study of astrophysical phenomena and atmospheric science.
Conclusion:
In summary, the root mean square velocity and most probable velocity are two important concepts that describe the behavior of particles in a gas. They are directly proportional to the square root of the temperature of the gas and are related to the Maxwell-Boltzmann distribution of velocities. These concepts are widely applicable in a range of fields and applications and are essential for understanding the behavior of particles in gases.