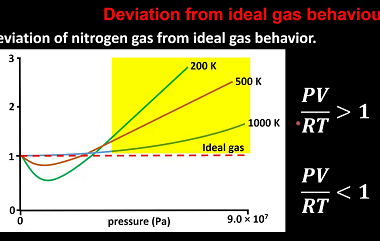
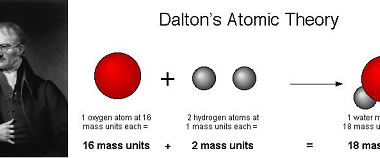
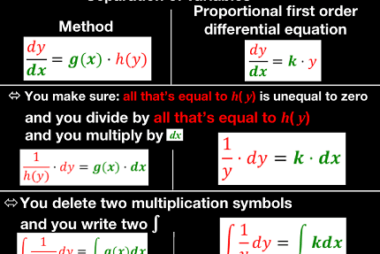
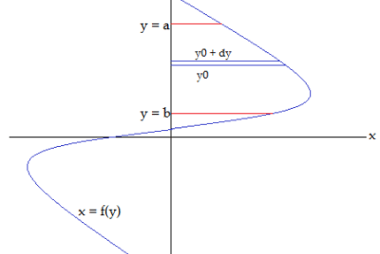
In many fields, including physics, chemistry, and engineering, the concept of an ideal situation or system is often used as a theoretical or idealized benchmark for comparison with real-world situations. However, in practice, it is rare to encounter a situation that perfectly conforms to the ideal model. When a system deviates from the ideal model,…
Gas laws describe the behavior of gases under different conditions, such as temperature, pressure, and volume. There are several gas laws, including Boyle’s law, Charles’s law, Gay-Lussac’s law, and the combined gas law, that relate these variables to one another. Boyle’s law states that at constant temperature, the volume of a gas is inversely proportional…
Molality and Normality are both measures of concentration used in chemistry. Molality (symbol: m) is defined as the number of moles of solute per kilogram of solvent. It is expressed in units of moles per kilogram (mol/kg). Molality is a useful measure of concentration when temperature changes occur because it is not dependent on temperature,…
Oxidation-reduction (redox) reactions involve the transfer of electrons between reactants. The mole concept and stoichiometry can be used to calculate the quantities of reactants and products involved in redox reactions. Here are some common calculations based on mole concept and stoichiometry involving redox reactions: 5Fe2+ + MnO4- + 8H+ → 5Fe3+ + Mn2+ + 4H2O…
Dalton’s atomic theory, proposed by John Dalton in the early 19th century, was a fundamental theory of chemistry that laid the groundwork for the modern understanding of atoms and their properties. The theory included the following key postulates: Dalton’s theory was an important contribution to the development of modern chemistry, as it provided a framework…
The scalar triple product and vector triple product are two different operations that involve three vectors in three-dimensional space. The scalar triple product of three vectors a, b, and c is defined as: a . (b x c) where “x” represents the cross product of vectors b and c, and “.” represents the dot product…
Dot Product: The dot product of two vectors is a scalar quantity that measures the degree of similarity between them. The dot product of two vectors A and B is defined as: A · B = |A| |B| cos(θ) where |A| and |B| are the magnitudes of the vectors A and B, respectively, and θ…
To add two vectors, you need to add their corresponding components. If you have two vectors, A and B, both with three components (x, y, z), then you can add them as follows: A + B = (Ax + Bx, Ay + By, Az + Bz) In other words, to add two vectors, you simply…
Separation of variables is a method used to solve certain types of differential equations. The method involves assuming that the solution to the differential equation can be expressed as a product of two functions, each of which depends on only one of the variables in the equation. For example, consider the partial differential equation: ∂u/∂t…
Definite integrals can be used to determine the area bounded by a simple curve and the x-axis, or by a simple curve and the y-axis. The area can be found by dividing it into small rectangles, finding the area of each rectangle, and then adding up the areas of all the rectangles. This process is…
