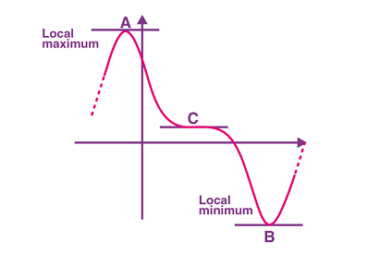
Maximum and Minimum values of a function
The maximum and minimum values of a function refer to the largest and smallest values that the function takes on, respectively. To find the maximum and minimum values of a function, we can use the following steps: It’s important to note that a function may not have a maximum or minimum value if it is…