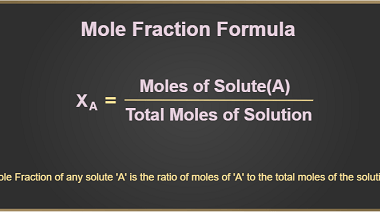
Molality and Normality are both measures of concentration used in chemistry. Molality (symbol: m) is defined as the number of moles of solute per kilogram of solvent. It is expressed in units of moles per kilogram (mol/kg). Molality is a useful measure of concentration when temperature changes occur because it is not dependent on temperature,…
Concentration can be expressed in terms of mole fraction, which is defined as the ratio of the number of moles of a particular substance to the total number of moles in the mixture. The mole fraction of a substance A in a mixture of n substances can be calculated using the following formula: Mole fraction…
A displacement reaction, also known as a replacement reaction, is a chemical reaction in which an atom or ion from one compound is replaced by an atom or ion of another compound. These reactions typically occur between a metal and a solution of a metal salt, or between a nonmetal and a solution of a…
Oxidation-reduction (redox) reactions involve the transfer of electrons between reactants. The mole concept and stoichiometry can be used to calculate the quantities of reactants and products involved in redox reactions. Here are some common calculations based on mole concept and stoichiometry involving redox reactions: 5Fe2+ + MnO4- + 8H+ → 5Fe3+ + Mn2+ + 4H2O…
The scalar triple product and vector triple product are two different operations that involve three vectors in three-dimensional space. The scalar triple product of three vectors a, b, and c is defined as: a . (b x c) where “x” represents the cross product of vectors b and c, and “.” represents the dot product…
Dot Product: The dot product of two vectors is a scalar quantity that measures the degree of similarity between them. The dot product of two vectors A and B is defined as: A · B = |A| |B| cos(θ) where |A| and |B| are the magnitudes of the vectors A and B, respectively, and θ…
Scalar multiplication is a mathematical operation that involves multiplying a scalar (a real number) by a vector, resulting in a new vector. The scalar multiplication of a vector is represented as: c * v = (c * v1, c * v2, c * v3, …, c * vn) where c is the scalar, v is…
A linear first-order differential equation has the form: y’ + p(x)y = q(x) where y’ denotes the derivative of y with respect to x, p(x) and q(x) are functions of x. To solve this equation, we use an integrating factor, which is a function u(x) that we multiply both sides of the equation by. We…
Separation of variables is a method used to solve certain types of differential equations. The method involves assuming that the solution to the differential equation can be expressed as a product of two functions, each of which depends on only one of the variables in the equation. For example, consider the partial differential equation: ∂u/∂t…
A homogeneous differential equation of the first order and first degree is an equation of the form: dy/dx = f(x,y) where f(x,y) is a homogeneous function of degree 1 in x and y. In other words, if we replace y with ky and x with kx for any constant k, the function f(x,y) remains the…
