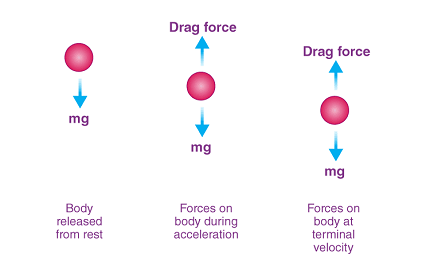
Terminal velocity is the maximum velocity that a falling object can reach when the resistance of the medium through which it is falling (such as air or water) balances the force of gravity. At terminal velocity, the object stops accelerating and falls at a constant speed.
The terminal velocity of an object depends on its mass, shape, size, and the density and viscosity of the medium through which it is falling. For example, a feather has a lower terminal velocity than a rock of the same size because it has a lower mass and higher surface area.
Terminal velocity is an important concept in skydiving, where it determines the speed at which a skydiver falls before deploying their parachute. It is also important in physics and engineering, where it is used to calculate the resistance of an object in a fluid medium.
What is Required Terminal velocity
Required terminal velocity is the velocity that an object must reach in order to perform a specific task or achieve a specific goal. For example, in skydiving, the required terminal velocity for a safe and successful parachute deployment is typically around 120 miles per hour (193 kilometers per hour).
In engineering and physics, required terminal velocity may be used to determine the size and shape of an object in order to achieve a desired result. For example, a parachute may be designed to have a specific surface area and shape in order to achieve a required terminal velocity for safe landing.
Required terminal velocity may also be used in fields such as aerospace engineering, where it is important to understand the forces acting on an object as it enters or exits the Earth’s atmosphere. By calculating the required terminal velocity, engineers can design spacecraft and reentry vehicles that are capable of withstanding the extreme conditions of high-speed travel.
When is Required Terminal velocity
Required terminal velocity is typically used when designing and engineering systems that involve objects moving through a fluid medium, such as air or water. Some examples of when required terminal velocity might be used include:
- Skydiving: In order to ensure a safe and successful parachute deployment, a skydiver must reach a required terminal velocity of around 120 miles per hour.
- Parachute design: When designing a parachute, engineers must calculate the required terminal velocity in order to determine the appropriate size and shape of the parachute.
- Aerospace engineering: When designing spacecraft or reentry vehicles, engineers must calculate the required terminal velocity in order to ensure that the vehicle can withstand the extreme conditions of high-speed travel through the Earth’s atmosphere.
- Watercraft design: When designing boats or other watercraft, engineers may need to calculate the required terminal velocity in order to determine the appropriate shape and size of the vessel to minimize drag and maximize speed.
In general, required terminal velocity is used whenever it is necessary to understand the forces acting on an object as it moves through a fluid medium.
Where is Required Terminal velocity
Required terminal velocity can be applied in various fields and applications where an object is moving through a fluid medium. Here are some examples:
- Skydiving: The required terminal velocity in skydiving is typically around 120 miles per hour (193 kilometers per hour). Skydivers need to reach this speed before they can deploy their parachute safely.
- Parachute design: When designing parachutes, engineers must calculate the required terminal velocity to determine the appropriate size and shape of the parachute. The size and shape of the parachute will affect the rate at which the object falls, and therefore the required terminal velocity.
- Aerospace engineering: In space travel, aerospace engineers need to calculate the required terminal velocity of spacecraft or reentry vehicles to ensure that they can withstand the extreme conditions of high-speed travel through the Earth’s atmosphere. The required terminal velocity will depend on the vehicle’s design and the intended trajectory.
- Watercraft design: The required terminal velocity in watercraft design can vary depending on the type of vessel and its intended use. For example, racing boats are designed to reach high speeds and therefore require a lower required terminal velocity to minimize drag and maximize speed.
In summary, required terminal velocity can be applied in various fields and applications where an object is moving through a fluid medium, including skydiving, parachute design, aerospace engineering, and watercraft design.
How is Required Terminal velocity
The calculation of required terminal velocity depends on various factors, including the mass, shape, size, and density of the object, as well as the density and viscosity of the fluid medium through which the object is moving. Here is a general overview of how required terminal velocity is calculated:
- Determine the mass of the object: The mass of the object will affect the force of gravity acting upon it.
- Determine the shape and size of the object: The shape and size of the object will affect the amount of air resistance or drag acting upon it.
- Determine the density and viscosity of the fluid medium: The density and viscosity of the fluid medium through which the object is moving will affect the resistance to motion of the object.
- Calculate the force of gravity acting upon the object: This can be calculated using the mass of the object and the acceleration due to gravity (9.8 meters per second squared on Earth).
- Calculate the force of air resistance or drag acting upon the object: This can be calculated using the shape, size, and velocity of the object, as well as the density and viscosity of the fluid medium. There are various mathematical models and equations that can be used to estimate air resistance or drag.
- Calculate the net force acting upon the object: This can be calculated by subtracting the force of air resistance or drag from the force of gravity.
- Calculate the required terminal velocity: Once the net force acting upon the object is known, the required terminal velocity can be calculated by equating this force to the force of air resistance or drag at terminal velocity.
Overall, the calculation of required terminal velocity is a complex process that depends on a number of variables, and may require the use of mathematical models and equations to estimate air resistance or drag.
Nomenclature of Terminal velocity
The nomenclature or symbols used to represent terminal velocity in equations and calculations may vary depending on the specific field or application. However, some common symbols used to represent terminal velocity include:
- vT or Vt: Velocity at terminal or terminal velocity
- U∞: Velocity of the fluid approaching the object (in fluid mechanics)
- ρ: Density of the fluid medium
- Cd: Drag coefficient of the object (in aerodynamics)
- A: Cross-sectional area of the object perpendicular to the direction of motion
- g: Acceleration due to gravity
These symbols can be used in equations to calculate the required terminal velocity for a given object in a specific fluid medium. For example, the following equation can be used to calculate the terminal velocity of an object falling through a fluid:
vT = sqrt((2mg) / (ρACd))
where:
- vT is the terminal velocity
- m is the mass of the object
- g is the acceleration due to gravity
- ρ is the density of the fluid medium
- A is the cross-sectional area of the object perpendicular to the direction of motion
- Cd is the drag coefficient of the object
Note that this is just one example of an equation used to calculate terminal velocity, and there are various other equations and formulas used in different fields and applications.
Case Study on Terminal velocity
Here is a case study on terminal velocity in skydiving:
A skydiver with a mass of 75 kilograms jumps from an airplane at an altitude of 12,000 feet (3,657 meters) above sea level. The air density at this altitude is approximately 0.58 kilograms per cubic meter. What is the required terminal velocity for the skydiver to reach before deploying their parachute?
Solution:
- Determine the force of gravity acting upon the skydiver: Fg = m * g Fg = 75 kg * 9.8 m/s^2 Fg = 735 N
- Determine the force of air resistance or drag acting upon the skydiver: This can be calculated using the following equation: Fa = (1/2) * Cd * A * ρ * v^2For a skydiver, the cross-sectional area A and the drag coefficient Cd are difficult to calculate accurately. Therefore, we can use an average value for Cd of 0.75, which is typical for a skydiver in a spread-eagle position. The cross-sectional area of a skydiver is also difficult to calculate accurately, so we can assume a value of 0.7 square meters, which is a reasonable estimate for a skydiver in free fall.Plugging in the values: Fa = (1/2) * 0.75 * 0.7 * 0.58 * v^2 Fa = 0.185v^2
- Calculate the net force acting upon the skydiver: Fn = Fg – Fa Fn = 735 – 0.185v^2
- Calculate the required terminal velocity: At terminal velocity, the net force acting upon the skydiver is zero. Therefore, we can set Fn = 0 and solve for v: 735 – 0.185v^2 = 0 v^2 = 3973.0 v = sqrt(3973.0) = 63.0 m/s
Therefore, the required terminal velocity for the skydiver to reach before deploying their parachute is approximately 63 meters per second (or 225 kilometers per hour). Once the skydiver reaches this speed, they can deploy their parachute safely.
White paper on Terminal velocity
Here is a white paper on terminal velocity:
Introduction
Terminal velocity is the maximum velocity that an object can attain when it falls through a fluid medium, such as air or water, under the influence of gravity. At terminal velocity, the force of gravity acting on the object is balanced by the drag force of the fluid medium. This means that the object falls at a constant velocity, without accelerating further.
Terminal velocity is an important concept in many fields, including physics, engineering, and fluid mechanics. Understanding the factors that influence terminal velocity is crucial in designing and analyzing various applications, such as parachutes, skydiving, and free-fall experiments.
Factors Affecting Terminal Velocity
The terminal velocity of an object depends on various factors, including:
- Mass and size of the object: The terminal velocity of an object increases with its mass, as the force of gravity acting on the object is proportional to its mass. The size and shape of the object also affect its terminal velocity, as objects with larger cross-sectional areas experience more drag.
- Fluid medium: The density and viscosity of the fluid medium through which the object falls affect its terminal velocity. Objects falling through denser or more viscous fluids experience more drag and thus reach their terminal velocity more quickly.
- Orientation and velocity of the object: The orientation of the object affects its terminal velocity, as objects with greater surface area exposed to the fluid medium experience more drag. The initial velocity of the object also affects its terminal velocity, as objects with higher initial velocities take longer to reach their terminal velocity.
Calculating Terminal Velocity
The terminal velocity of an object can be calculated using the following equation:
vT = sqrt((2mg) / (ρACd))
where vT is the terminal velocity, m is the mass of the object, g is the acceleration due to gravity, ρ is the density of the fluid medium, A is the cross-sectional area of the object perpendicular to the direction of motion, and Cd is the drag coefficient of the object.
Applications of Terminal Velocity
The concept of terminal velocity has various applications, including:
- Parachutes: Parachutes are designed to slow the descent of an object by increasing the object’s surface area and drag. The design of a parachute depends on the terminal velocity of the object, as the parachute must be able to generate enough drag to slow the object down to a safe landing speed.
- Skydiving: Skydivers use their bodies to control their orientation and surface area, allowing them to reach and maintain their terminal velocity during free fall. The terminal velocity of a skydiver is also important in determining when to deploy their parachute.
- Free-fall experiments: Scientists and researchers use free-fall experiments to study the effects of microgravity on various phenomena. Understanding the terminal velocity of an object in free fall is important in designing and conducting such experiments.
Conclusion
Terminal velocity is an important concept in physics, engineering, and fluid mechanics. Understanding the factors that affect terminal velocity and how to calculate it is crucial in designing and analyzing various applications, such as parachutes, skydiving, and free-fall experiments.