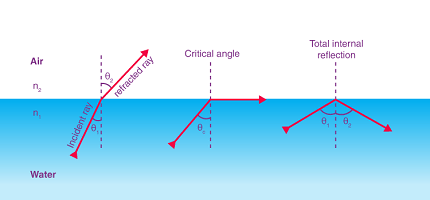
Total internal reflection is a phenomenon that occurs when a light ray passes from a medium with a higher refractive index to a medium with a lower refractive index at an angle greater than the critical angle. When this happens, the light ray is reflected back into the higher refractive index medium rather than being refracted into the lower refractive index medium.
The critical angle is the smallest angle of incidence at which total internal reflection occurs, and it is dependent on the refractive indices of the two media. The formula for calculating the critical angle is:
sin(critical angle) = n2/n1
where n1 is the refractive index of the higher refractive index medium, and n2 is the refractive index of the lower refractive index medium.
Total internal reflection is used in many practical applications, including optical fibers, prisms, and mirages. In optical fibers, total internal reflection is used to keep the light signal within the fiber by reflecting the light back into the fiber when it reaches the boundary between the fiber and the surrounding medium. In prisms, total internal reflection is used to reflect light at specific angles, allowing for the dispersion of white light into its component colors. Mirages are also caused by total internal reflection, where light is refracted by a hot surface, such as a road or desert, and then reflected back by a layer of air of a different temperature, creating the illusion of a water surface.
What is Total internal reflection
Total internal reflection is a phenomenon that occurs when a light ray travels from a medium with a higher refractive index to a medium with a lower refractive index at an angle of incidence greater than the critical angle. Instead of refracting, the light ray is reflected back into the medium with the higher refractive index.
The critical angle is the minimum angle of incidence at which total internal reflection occurs and is determined by the refractive indices of the two media. The formula for calculating the critical angle is sinθc = n2/n1, where θc is the critical angle, n1 is the refractive index of the medium with the higher index of refraction, and n2 is the refractive index of the medium with the lower index of refraction.
Total internal reflection has practical applications in optics, including in fiber optics, where it is used to transmit light signals over long distances. Total internal reflection also plays a role in the formation of mirages and the reflection of light in prisms.
When is Total internal reflection
Total internal reflection occurs when a light ray travels from a medium with a higher refractive index to a medium with a lower refractive index at an angle of incidence greater than the critical angle. The critical angle is the minimum angle of incidence at which total internal reflection occurs, and it depends on the refractive indices of the two media involved.
For example, if a light ray travels from water, which has a higher refractive index, to air, which has a lower refractive index, it can undergo total internal reflection if the angle of incidence is greater than the critical angle, which is approximately 48.6 degrees. This means that if the light ray enters the air at an angle greater than 48.6 degrees, it will be totally reflected back into the water, rather than refracted into the air.
Total internal reflection has a variety of practical applications, such as in optical fibers for communication, prisms for dispersing light, and mirages caused by the bending of light in Earth’s atmosphere.
Where is Total internal reflection
Total internal reflection can occur at the boundary between two different transparent media, such as air and water, or between two different types of glass with different refractive indices. The conditions necessary for total internal reflection to occur include:
- The light ray must travel from a medium with a higher refractive index to a medium with a lower refractive index.
- The angle of incidence of the light ray must be greater than the critical angle, which is determined by the refractive indices of the two media involved.
Examples of where total internal reflection occurs include:
- Optical fibers: Total internal reflection is used to keep light signals within an optical fiber, which is made of glass or plastic, by reflecting the light back into the fiber when it reaches the boundary with the surrounding medium.
- Mirages: Total internal reflection plays a role in the formation of mirages, which are optical illusions caused by the bending of light in Earth’s atmosphere. For example, a hot road surface can create a layer of hot air near the surface, which has a lower refractive index than the cooler air above it. This can cause light to be refracted and then reflected back down towards the ground, creating the appearance of water on the road.
- Prisms: Total internal reflection is used in prisms to reflect and disperse light at specific angles, allowing for the separation of white light into its component colors.
How is Total internal reflection
Total internal reflection occurs when a light ray travels from a medium with a higher refractive index to a medium with a lower refractive index at an angle of incidence greater than the critical angle. This causes the light ray to be reflected back into the higher refractive index medium, rather than refracted into the lower refractive index medium.
The critical angle is determined by the refractive indices of the two media involved and is defined by the equation sinθc = n2/n1, where θc is the critical angle, n1 is the refractive index of the higher refractive index medium, and n2 is the refractive index of the lower refractive index medium.
When the angle of incidence is greater than the critical angle, the light ray is reflected back into the higher refractive index medium at an angle equal to the angle of incidence. This reflection is called total internal reflection because all of the incident light is reflected back into the medium with the higher refractive index.
Total internal reflection is important in many practical applications, including in fiber optic communication, where it allows for the transmission of light signals over long distances. It is also used in prisms for the dispersion of light and in the formation of mirages caused by the bending of light in Earth’s atmosphere.
Structures of Total internal reflection
Total internal reflection occurs when a light ray is incident on the boundary between two different transparent media at an angle greater than the critical angle, which is determined by the refractive indices of the two media. The critical angle is the angle of incidence at which the light ray is refracted along the boundary between the two media, rather than passing through it.
The structure of total internal reflection involves the interaction of two media with different refractive indices, with the boundary between the two media acting as a reflective surface. When the light ray is incident on the boundary at an angle greater than the critical angle, the entire light ray is reflected back into the higher refractive index medium, rather than refracting into the lower refractive index medium.
The phenomenon of total internal reflection has many practical applications in optics, such as in fiber optic communication, where it allows for the transmission of light signals over long distances. Total internal reflection is also used in prisms for the dispersion of light, and in the formation of mirages caused by the bending of light in Earth’s atmosphere.
In order to maximize the effect of total internal reflection, the surfaces of the materials involved are often designed with a special coating that minimizes the amount of light that is absorbed or scattered, which can reduce the efficiency of total internal reflection. This is particularly important in applications such as optical fibers, where the transmission of light signals over long distances requires a high degree of efficiency and minimal signal loss.
Case Study on Total internal reflection
One example of the practical application of total internal reflection is in the technology of optical fibers used in telecommunication networks. Optical fibers are made of glass or plastic and are used to transmit information in the form of light pulses over long distances with minimal signal loss.
The core of an optical fiber is surrounded by a cladding material with a lower refractive index. This creates a boundary between the core and the cladding, which is used to guide and reflect light within the core of the fiber. When light enters the core of the fiber, it is guided along the length of the fiber through total internal reflection.
The light pulse, consisting of a series of light rays, is transmitted through the core of the fiber, reflecting off the boundary between the core and cladding at each point along the length of the fiber. This reflection occurs because the angle of incidence of the light rays is greater than the critical angle, causing total internal reflection to occur at each boundary.
The use of total internal reflection in optical fibers allows for the transmission of light signals over long distances with minimal signal loss. This is because the light signal is reflected along the length of the fiber, rather than being absorbed or scattered by the material of the fiber. This makes optical fibers an efficient and effective means of transmitting information over long distances, and they are used extensively in telecommunication networks for this purpose.
Overall, the use of total internal reflection in optical fibers is a key example of how the phenomenon can be harnessed for practical applications in modern technology.
White paper on Total internal reflection
Here is a white paper on Total internal reflection:
Introduction
Total internal reflection (TIR) is a phenomenon that occurs when a light ray traveling from a medium with a higher refractive index to a medium with a lower refractive index is reflected back into the higher refractive index medium rather than refracted into the lower refractive index medium. This occurs when the angle of incidence of the light ray is greater than the critical angle, which is determined by the refractive indices of the two media involved. Total internal reflection is an important concept in optics and has many practical applications in areas such as fiber optic communication and prism technology.
History
The phenomenon of total internal reflection was first described by the Dutch mathematician and physicist Willebrord Snell in 1621. Snell discovered the law of refraction, which describes how light is refracted when it passes from one medium to another. This law was later used by James Clerk Maxwell in his theory of electromagnetic waves, which describes how light propagates as an electromagnetic wave through space.
The concept of total internal reflection was first observed in the early 1800s by the physicist John William Strutt, also known as Lord Rayleigh. Rayleigh observed that light traveling through a glass prism could be reflected back into the prism at certain angles of incidence, creating a bright spot of light known as a caustic.
Applications
Total internal reflection has many practical applications in optics and technology. One important application is in fiber optic communication. Optical fibers are made of glass or plastic and are used to transmit information in the form of light pulses over long distances with minimal signal loss. The core of an optical fiber is surrounded by a cladding material with a lower refractive index, which creates a boundary between the core and cladding that is used to guide and reflect light within the core of the fiber through total internal reflection.
Total internal reflection is also used in prism technology, where it is used to disperse and reflect light. Prisms are made of glass or plastic and are used in many optical instruments, such as cameras and telescopes, to direct and focus light. When light enters a prism, it is refracted as it passes through the prism and is then reflected off the surfaces of the prism through total internal reflection, creating the characteristic rainbow-like dispersion of colors.
Another application of total internal reflection is in the formation of mirages. A mirage is an optical illusion caused by the bending of light in Earth’s atmosphere. When light passes from one medium to another with different refractive indices, such as air and the ground, it can be refracted and reflected through total internal reflection, creating the appearance of objects that are not actually present.
Conclusion
Total internal reflection is a fundamental concept in optics that has many practical applications in technology. From fiber optic communication to prism technology, the use of total internal reflection allows for the efficient and effective transmission, redirection, and dispersion of light. Understanding the principles of total internal reflection is crucial for the development of new technologies and for furthering our understanding of the behavior of light.