Vibration of strings is a physical phenomenon that occurs when a string, such as that of a musical instrument, is plucked or struck. When a string is plucked, it vibrates back and forth, causing sound waves to be produced. The frequency and amplitude of the vibration determine the pitch and volume of the sound produced.

The vibration of a string is governed by a number of factors, including its tension, length, and mass per unit length. These properties determine the fundamental frequency of the string, as well as the harmonics that are produced as the string vibrates.

Musical instruments such as guitars, violins, and pianos all rely on the vibration of strings to produce sound. The way in which the strings are plucked or struck, as well as the length and thickness of the strings, can be adjusted to produce different tones and timbres.

The physics of string vibration has been studied extensively by scientists and mathematicians, and the resulting equations have applications in fields such as acoustics, engineering, and physics.

What is Required Vibration of strings

The required vibration of strings depends on the application or context in which the string is being used.

In the context of musical instruments, the required vibration of strings is determined by the desired pitch and tone of the instrument. For example, a guitar string that is tuned to play the note E (the lowest string on a standard guitar) must vibrate at a frequency of approximately 82.4 Hz. The required vibration of a string can be adjusted by changing the tension, length, or mass per unit length of the string.

In other applications, such as in engineering or physics, the required vibration of strings may be used to measure properties such as tension, stiffness, or damping. In these cases, the vibration of the string can be measured and analyzed to determine the properties of the material or system being studied.

Overall, the required vibration of strings is dependent on the specific application or context in which the string is being used, and can be adjusted through various means to achieve the desired outcome.

When is Required Vibration of strings

The required vibration of strings occurs whenever a string is used to produce sound, measure properties of materials, or in other applications where vibration is necessary.

In musical instruments, the required vibration of strings is necessary to produce the desired pitch and tone of the instrument. For example, a guitar string must vibrate at a specific frequency to produce a certain note. The frequency of the vibration is determined by the length, tension, and mass per unit length of the string.

In engineering and physics, the required vibration of strings can be used to measure properties such as tension, stiffness, and damping. By measuring the vibration of a string under different conditions, engineers and scientists can gain insights into the properties of materials and systems.

Overall, the required vibration of strings is an important aspect of many applications, including music, engineering, and physics, and is necessary whenever vibration is used to achieve a specific outcome.

Where is Required Vibration of strings

The required vibration of strings can occur in various places depending on the specific application or context in which the vibration is needed.

In musical instruments, such as guitars, violins, or pianos, the required vibration of strings occurs within the instrument itself. The strings are usually stretched between two points, such as the bridge and the nut on a guitar, and are either plucked or struck by a mechanism in the instrument, such as the hammer in a piano. The resulting vibration of the string produces the sound that is heard.

In engineering and physics, the required vibration of strings may occur in a laboratory setting where researchers are studying the properties of materials or systems. In this context, the vibration of strings can be measured using various instruments and techniques, such as laser interferometry or strain gauges.

Overall, the required vibration of strings can occur in a variety of settings and contexts, depending on the specific application and the desired outcome.

How is Required Vibration of strings

The required vibration of strings is achieved by plucking, striking, or otherwise exciting the string, causing it to vibrate at a specific frequency. The frequency of the vibration is determined by the physical properties of the string, including its length, tension, and mass per unit length.

In musical instruments, the required vibration of strings is achieved by plucking, strumming, or bowing the string, which sets the string into motion and causes it to vibrate. The length and thickness of the string, as well as the tension applied to it, determine the frequency of the vibration and, therefore, the pitch of the sound produced.

In engineering and physics, the required vibration of strings can be induced through various means, such as through the use of an external force or by applying an electrical signal to the string. The vibration of the string can then be measured and analyzed to determine the properties of the material or system being studied.

Overall, the required vibration of strings can be achieved through various means, depending on the specific application and the desired outcome. The physical properties of the string, such as length, tension, and mass per unit length, play a critical role in determining the frequency of the vibration and the resulting sound or measurement.

Nomenclature of Vibration of strings

The nomenclature of vibration of strings refers to the terms and symbols used to describe the various parameters and characteristics of string vibration. Some common terms and symbols used in the nomenclature of vibration of strings include:

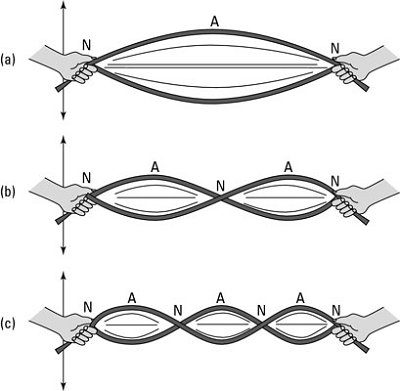
• Frequency (f): The number of cycles per second of the vibration, measured in Hertz (Hz).
• Period (T): The time it takes for one cycle of the vibration, measured in seconds.
• Amplitude (A): The maximum displacement of the string from its resting position during the vibration, measured in meters.
• Wavelength (λ): The distance between two consecutive points on the string that are in phase, measured in meters.
• Wave speed (v): The speed at which the wave propagates through the string, measured in meters per second.
• Tension (T): The amount of force applied to the string, measured in Newtons (N).
• Linear mass density (μ): The mass per unit length of the string, measured in kilograms per meter (kg/m).

These parameters and symbols can be used to describe and analyze the vibration of strings in various applications, including music, engineering, and physics.

Case Study on Vibration of strings

One example of a case study on the vibration of strings is the analysis of guitar strings. The vibration of guitar strings is critical to the sound and playability of the instrument. By understanding the properties and behavior of guitar strings, engineers and musicians can design and select strings that produce the desired sound and feel.

In a study published in the Journal of Sound and Vibration, researchers analyzed the vibration of guitar strings using a combination of experimental and numerical techniques. They measured the frequency response of guitar strings using laser vibrometry and analyzed the results using finite element simulations.

The researchers found that the frequency response of the strings was affected by a number of factors, including the material properties of the string, the tension applied to the string, and the geometry of the string. They also observed that the damping characteristics of the strings were important in determining the overall sound of the instrument.

The study demonstrated the importance of understanding the vibration of guitar strings in designing and selecting strings for specific musical applications. By analyzing the vibration characteristics of strings, engineers and musicians can make informed decisions about string selection and design, leading to better performance and sound quality in musical instruments.

Overall, this case study highlights the importance of understanding the vibration of strings in various applications, including music, engineering, and physics. The analysis of string vibration can provide insights into the behavior of materials and systems, leading to improved design and performance.

White paper on Vibration of strings

Introduction:

The vibration of strings is a fascinating phenomenon that has been studied for centuries. It is essential to various fields, including music, engineering, and physics. The purpose of this white paper is to provide an overview of the vibration of strings, including its properties, behavior, and applications.

Properties of Vibration of Strings:

The vibration of strings is characterized by several properties, including frequency, period, amplitude, wavelength, wave speed, tension, and linear mass density. These properties are interrelated and can be used to describe and analyze the vibration of strings.

Frequency refers to the number of cycles per second of the vibration and is measured in Hertz (Hz). The period is the time it takes for one cycle of the vibration and is measured in seconds. Amplitude is the maximum displacement of the string from its resting position during the vibration and is measured in meters. Wavelength is the distance between two consecutive points on the string that are in phase and is measured in meters. Wave speed is the speed at which the wave propagates through the string and is measured in meters per second. Tension is the amount of force applied to the string and is measured in Newtons (N). Linear mass density is the mass per unit length of the string and is measured in kilograms per meter (kg/m).

Behavior of Vibration of Strings:

The behavior of the vibration of strings is affected by various factors, including the properties of the string, the tension applied to the string, and the geometry of the string. The vibration of strings can be induced by plucking, striking, or otherwise exciting the string, causing it to vibrate at a specific frequency.

In musical instruments, such as guitars, violins, and pianos, the vibration of strings is critical to the sound and playability of the instrument. By understanding the properties and behavior of strings, engineers and musicians can design and select strings that produce the desired sound and feel.

Applications of Vibration of Strings:

The vibration of strings has various applications, including music, engineering, and physics. In music, the vibration of strings is essential to the sound and playability of instruments such as guitars, violins, and pianos. In engineering and physics, the vibration of strings can be induced through various means, such as through the use of an external force or by applying an electrical signal to the string. The vibration of the string can then be measured and analyzed to determine the properties of the material or system being studied.

Conclusion:

The vibration of strings is a complex phenomenon that has been studied for centuries. It is essential to various fields, including music, engineering, and physics. By understanding the properties and behavior of strings, engineers and musicians can design and select strings that produce the desired sound and feel. The analysis of string vibration can provide insights into the behavior of materials and systems, leading to improved design and performance.