The ideal gas law is a fundamental concept in thermodynamics that describes the behavior of gases under certain conditions. It is a combination of several gas laws, including Boyle’s law, Charles’s law, and Avogadro’s law, and can be expressed mathematically as:
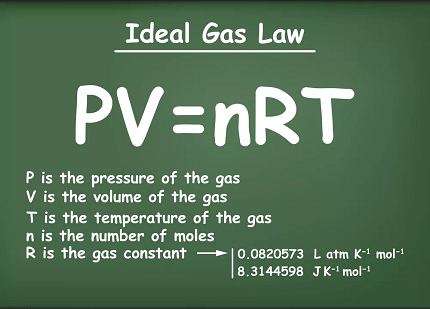
PV = nRT
Where: P = pressure of the gas V = volume of the gas n = number of moles of gas R = the ideal gas constant T = temperature of the gas
The ideal gas law assumes that the gas molecules are small, have no volume, and do not interact with each other. It is most accurate for gases at low pressures and high temperatures. At high pressures and low temperatures, the behavior of real gases deviates from that predicted by the ideal gas law, and more complex equations must be used to describe their behavior.
What is Required Ideal gas laws
The ideal gas law equation requires four variables to be defined: pressure (P), volume (V), number of moles (n), and temperature (T).
Pressure (P) is the force exerted by the gas on the walls of its container per unit area. It is typically measured in units of pascals (Pa), but other units like atm, bar, and psi are also commonly used.
Volume (V) is the amount of space occupied by the gas. It is typically measured in units of cubic meters (m³), but other units like liters (L) and cubic feet (ft³) are also commonly used.
Number of moles (n) is a measure of the amount of gas present, and it is defined as the number of atoms or molecules divided by Avogadro’s number (6.022 x 10²³).
Temperature (T) is the average kinetic energy of the gas molecules. It is typically measured in units of Kelvin (K), but other units like Celsius (°C) and Fahrenheit (°F) can also be used.
In summary, to use the ideal gas law equation, you need to know the pressure, volume, number of moles, and temperature of a gas.
When is Required Ideal gas laws
The ideal gas law is used to describe the behavior of gases under conditions of low pressure and high temperature, where the gas molecules are assumed to have negligible volume and do not interact with each other.
The ideal gas law can be used to solve a wide range of problems in physics and chemistry, including the calculation of the pressure, volume, temperature, or number of moles of a gas. It is particularly useful in thermodynamics, where it is used to describe the behavior of ideal gases in processes such as compression, expansion, heating, and cooling.
However, it is important to note that the ideal gas law is only an approximation, and its accuracy decreases as the pressure and temperature of the gas increase. Under conditions of high pressure and low temperature, the behavior of real gases deviates from that predicted by the ideal gas law, and more complex equations must be used to describe their behavior.
Where is Required Ideal gas laws
The ideal gas law is used in many fields of science and engineering, including physics, chemistry, and mechanical engineering.
In physics, the ideal gas law is used to describe the behavior of gases in many different contexts, including thermodynamics, fluid dynamics, and statistical mechanics. For example, it is used to model the behavior of gases in stars, to describe the behavior of gases in weather systems, and to understand the behavior of gases in combustion engines.
In chemistry, the ideal gas law is used to calculate the behavior of gases in chemical reactions, to predict the behavior of gases under different conditions, and to design and optimize chemical processes. For example, it is used to calculate the amount of gas produced or consumed in a chemical reaction, to determine the conditions required for a reaction to occur, and to design gas-handling equipment.
In mechanical engineering, the ideal gas law is used to design and optimize engines, turbines, and other machines that use gases as working fluids. For example, it is used to calculate the efficiency of a combustion engine, to design the cooling systems for gas turbines, and to optimize the flow of gases through pipelines and ducts.
In summary, the ideal gas law is used in a wide range of scientific and engineering fields to describe and predict the behavior of gases under different conditions.
How is Required Ideal gas laws
The ideal gas law describes the behavior of ideal gases, which are gases that follow certain assumptions. These assumptions include that the gas particles have negligible volume, there are no intermolecular forces between the particles, and that the particles are in constant, random motion.
The ideal gas law is expressed as PV = nRT, where P is the pressure of the gas, V is its volume, n is the number of moles of gas, R is the ideal gas constant, and T is the temperature of the gas. This equation relates the pressure, volume, temperature, and number of moles of an ideal gas.
To use the ideal gas law, one needs to know the values of three of these variables and solve for the fourth. For example, if the volume, pressure, and number of moles of a gas are known, the temperature of the gas can be calculated using the ideal gas law.
The ideal gas law can also be rearranged to derive other useful equations. For example, by rearranging the ideal gas law equation, one can derive Boyle’s law (P₁V₁ = P₂V₂), Charles’s law (V₁/T₁ = V₂/T₂), and Avogadro’s law (V₁/n₁ = V₂/n₂).
It is important to note that while the ideal gas law is a useful approximation for the behavior of real gases under certain conditions, it does not accurately describe the behavior of gases at high pressures or low temperatures. In such cases, more complex equations that take into account the non-ideal behavior of gases must be used.
Structures of Ideal gas laws
The ideal gas law is a mathematical equation that describes the relationship between pressure (P), volume (V), number of moles (n), and temperature (T) for an ideal gas. It is expressed as:
PV = nRT
where P is the pressure of the gas, V is its volume, n is the number of moles of gas, R is the ideal gas constant, and T is the temperature of the gas.
The ideal gas law equation can be used to derive other useful equations, such as:
- Boyle’s law: P₁V₁ = P₂V₂, which describes the inverse relationship between pressure and volume of a gas at constant temperature and number of moles.
- Charles’s law: V₁/T₁ = V₂/T₂, which describes the direct relationship between volume and temperature of a gas at constant pressure and number of moles.
- Avogadro’s law: V₁/n₁ = V₂/n₂, which describes the direct relationship between volume and number of moles of a gas at constant temperature and pressure.
In addition to the ideal gas law equation and its derived equations, there are several other equations that are used to describe the behavior of real gases, taking into account their non-ideal behavior. These include the Van der Waals equation, which accounts for the volume and intermolecular forces of real gases, and the virial equation, which accounts for the non-ideal behavior of gases in terms of a series of mathematical terms.
Overall, the ideal gas law and its derived equations are fundamental to the study of the behavior of gases in various fields, including chemistry, physics, and engineering.
Case Study on Ideal gas laws
One example of a case study involving the ideal gas law is the design of an internal combustion engine. In an internal combustion engine, a fuel-air mixture is ignited inside a cylinder, causing the pressure and temperature of the gases to increase rapidly. This increase in pressure and temperature forces a piston to move, which is then used to generate mechanical energy.
The ideal gas law is used to model the behavior of the fuel-air mixture inside the cylinder. For example, the ideal gas law can be used to calculate the pressure and temperature of the gases at different stages of the engine cycle. The ideal gas law can also be used to determine the amount of fuel and air needed for a given engine design, based on the desired power output and efficiency.
However, it is important to note that the behavior of real gases in an internal combustion engine is not perfectly described by the ideal gas law, due to the non-ideal behavior of gases under high pressures and temperatures. More complex models, such as the adiabatic flame temperature model, must be used to accurately describe the combustion process in an internal combustion engine.
Another example of a case study involving the ideal gas law is the design of a gas turbine. In a gas turbine, high-pressure gases are expanded through a turbine, generating mechanical energy that can be used to power a generator or other machinery.
The ideal gas law is used to model the behavior of the gases in the turbine. For example, the ideal gas law can be used to calculate the pressure and temperature of the gases at different stages of the turbine. The ideal gas law can also be used to determine the efficiency of the turbine, based on the pressure and temperature ratios across the turbine.
Again, it is important to note that the behavior of real gases in a gas turbine is not perfectly described by the ideal gas law, and more complex models, such as the Brayton cycle, must be used to accurately describe the performance of the turbine.
In summary, the ideal gas law is a fundamental tool in the design and analysis of many different types of machinery and processes that involve the behavior of gases. While the ideal gas law is a useful approximation in many cases, more complex models may be required to accurately describe the behavior of real gases under different conditions.
White paper on Ideal gas laws
Title: Ideal Gas Laws: Theory, Applications, and Limitations
Abstract:
The ideal gas laws are a set of equations that describe the behavior of gases under ideal conditions. They are based on the assumptions that gas particles have negligible volume and no intermolecular forces, and that they move randomly in all directions at a constant velocity. The ideal gas laws are widely used in various fields of science and engineering, including chemistry, physics, and thermodynamics.
This white paper provides a comprehensive overview of the ideal gas laws, including their theory, applications, and limitations. It begins with a brief introduction to the historical background and development of the ideal gas laws, and then presents the three main equations that make up the ideal gas laws: the Boyle’s law, Charles’s law, and Avogadro’s law. The ideal gas law equation, which combines all three laws, is then derived and explained in detail.
The applications of the ideal gas laws in different fields are then discussed, including their use in the design and analysis of various types of machinery and processes, such as internal combustion engines, gas turbines, and refrigeration systems. The ideal gas laws are also used to calculate the behavior of gases in chemical reactions, such as the calculation of reaction rates and equilibrium constants.
The limitations of the ideal gas laws are also explored, including their inability to accurately describe the behavior of real gases under non-ideal conditions, such as high pressures and low temperatures. The deviations from ideal gas behavior are discussed, and more complex models, such as the Van der Waals equation, are introduced as a way to account for these deviations.
Finally, the white paper concludes with a summary of the key points and an overview of the current research trends in the field of gas laws. Overall, the ideal gas laws are a fundamental tool in the study and analysis of gases in various fields, and their applications and limitations must be carefully considered in order to make accurate predictions and calculations.
Keywords: ideal gas laws, Boyle’s law, Charles’s law, Avogadro’s law, gas behavior, thermodynamics, chemical reactions, non-ideal gases, Van der Waals equation.