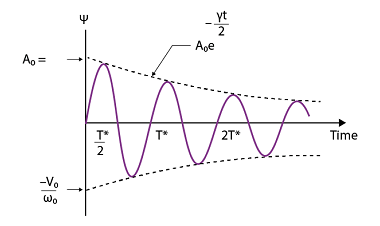
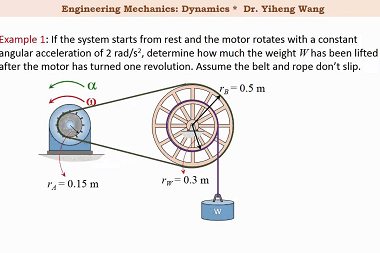
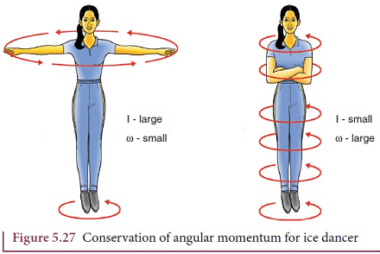
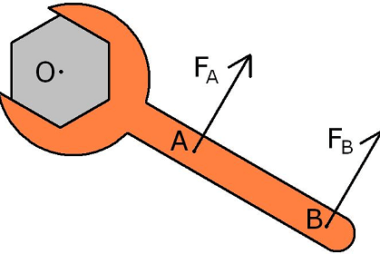
Damped oscillation refers to the behavior of a system that undergoes oscillation (i.e., periodic motion) but with the amplitude of the oscillation decreasing over time due to the presence of damping. In one dimension, a simple example of a damped oscillation is a mass-spring system with damping, where a mass is attached to a spring…
“Mechanics forced” could refer to a few different things depending on the context, but one possible interpretation is the concept of forced motion in mechanics. Forced motion in mechanics refers to a system that is subject to external forces that cause it to move in a specific way, rather than moving according to its own…
When a point mass collides with a rigid body, the collision can be classified as either elastic or inelastic. In an elastic collision, the total kinetic energy of the system is conserved. That is, the kinetic energy of the point mass and the rigid body before the collision is equal to the kinetic energy of…
The equilibrium of rigid bodies refers to the state of a rigid body when it is at rest or moving with a constant velocity, and the net force and torque acting on it are both zero. In other words, the body is not accelerating and is in a state of balance. To determine the equilibrium…
Cylinders and spheres are both three-dimensional shapes commonly encountered in mathematics and the physical world. Here are some important characteristics of each shape: Cylinder: Spheres: Both cylinders and spheres have properties that make them useful in a variety of applications. For example, cylinders are commonly used as containers for liquids or gases because they have…
When a ring rolls without slipping, it means that the ring is rotating around its axis while also translating without any slipping on a surface. In this situation, the point on the ring’s edge that is in contact with the surface has zero velocity relative to the surface, while the ring’s center of mass is…
The dynamics of rigid bodies with a fixed axis of rotation can be described using rotational motion equations and principles. Firstly, it’s important to note that for a rigid body rotating about a fixed axis, all particles of the body rotate in circles or arcs about the axis, with the same angular velocity. The rotational…
Conservation of angular momentum is a fundamental principle in physics that states that the total angular momentum of a system is conserved in the absence of external torques. Angular momentum is a property of rotating objects and is defined as the product of the moment of inertia and the angular velocity. In simpler terms, the…
Torque is a measure of the twisting force that an object experiences when a force is applied to it. It is a vector quantity, meaning it has both magnitude and direction. In physics, torque is often denoted by the symbol “τ” and is measured in units of newton-meters (N·m) or pound-feet (lb·ft). Torque is calculated…
Angular momentum is a fundamental concept in physics that describes the rotational motion of an object or system. It is a vector quantity that has both magnitude and direction, and is defined as the product of the moment of inertia of the object and its angular velocity. The moment of inertia is a measure of…
