Integration by substitution is a technique used to simplify an integral by replacing the variable of integration with a new variable. This new variable is chosen so that the resulting integral becomes easier to evaluate. The basic steps for integration by substitution are as follows:
- Identify a sub-expression within the integrand that can be simplified by substitution. This sub-expression is typically a function of the variable of integration.
- Choose a new variable of integration by letting the sub-expression equal the new variable. This step is called the substitution step.
- Rewrite the integrand in terms of the new variable.
- Differentiate the new variable with respect to the original variable to obtain an expression for the differential, and substitute this into the rewritten integrand.
- Evaluate the resulting integral using the rules of integration.
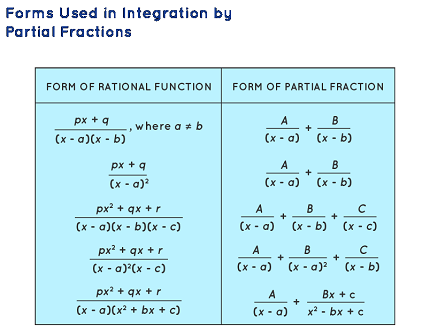
Partial fraction decomposition is a technique used to simplify a rational function by breaking it down into simpler, more easily integrable parts. The basic steps for partial fraction decomposition are as follows:
- Factor the denominator of the rational function into its irreducible factors.
- Write the rational function as a sum of partial fractions, where each partial fraction has a denominator that is one of the irreducible factors of the original denominator.
- Determine the constants that appear in each partial fraction by comparing the numerators of both sides of the equation obtained in step 2.
- Use the resulting partial fraction decomposition to evaluate the integral of the original rational function.
These two techniques can be used together in some cases to simplify the integrand before integration by substitution. For example, if the integrand is a rational function, it may be simplified using partial fraction decomposition before a substitution is made to simplify the resulting integral.
What is Required Integration by the methods of substitution and partial fractions
The process of integration by substitution involves finding a suitable substitution that can transform the integrand into a simpler form that can be easily integrated. This method is useful for integrals of the form ∫f(g(x))g'(x)dx, where f and g are functions.
To perform integration by substitution, you need to identify the inner function g(x) and the corresponding derivative g'(x), and then substitute a new variable u for the inner function, so that the integral can be rewritten in terms of the new variable u. This allows you to evaluate the integral using standard integration techniques.
The process of partial fractions involves decomposing a rational function into simpler fractions that can be more easily integrated. This method is useful for integrals of the form ∫(P(x)/Q(x))dx, where P(x) and Q(x) are polynomials.
To perform partial fractions, you need to factor the denominator Q(x) into irreducible factors and then write the rational function P(x)/Q(x) as a sum of simpler fractions with denominators equal to each of the irreducible factors. You then solve for the constants in the partial fraction decomposition by equating the numerators of both sides of the equation.
By combining integration by substitution and partial fractions, you can simplify more complicated integrals and make them easier to solve. For example, if you have an integral of the form ∫(P(x)/Q(g(x)))g'(x)dx, you can use integration by substitution to transform the integral into a simpler form and then use partial fractions to decompose the resulting rational function.
Who is Required Integration by the methods of substitution and partial fractions
Integration by substitution and partial fractions are techniques that are commonly used in calculus, a branch of mathematics that deals with rates of change and accumulation. These techniques are typically studied in calculus courses at the high school or college level.
Students who are studying calculus, particularly those who are learning how to integrate functions, will need to know how to use integration by substitution and partial fractions to evaluate integrals. These techniques are important for solving a wide range of problems in calculus, physics, engineering, and other scientific fields.
Professionals in fields such as physics, engineering, and economics may also use integration by substitution and partial fractions to solve more complex problems related to their respective fields. These techniques are particularly useful in fields that involve modeling and analyzing rates of change, such as fluid dynamics, thermodynamics, and financial analysis.
When is Required Integration by the methods of substitution and partial fractions
Integration by substitution and partial fractions are required when you are faced with an integral that is difficult to solve using basic integration rules. These techniques are particularly useful for integrating functions that involve nested expressions, complex polynomials, or rational functions.
Integration by substitution is typically used when the integrand involves a composite function, where the inner function can be substituted with a new variable to simplify the expression. For example, the integral of sin(2x)cos(2x)dx can be simplified using the substitution u = sin(2x), which transforms the integral into the simpler form of ∫(1/2)u du.
Partial fractions, on the other hand, are used to simplify integrals of rational functions, where the denominator can be factored into irreducible factors. For example, the integral of (3x^2 + 5x + 7)/(x^3 – 1)dx can be solved using partial fractions, which allows you to break the rational function into simpler fractions with denominators equal to each of the irreducible factors.
In general, integration by substitution and partial fractions are required when you are faced with a complex integral that cannot be solved using basic integration techniques. These techniques are important tools for solving a wide range of problems in calculus, physics, engineering, and other scientific fields.
Where is Required Integration by the methods of substitution and partial fractions
Integration by substitution and partial fractions are used in a variety of fields where calculus is applied, including:
- Physics: Integration is used in physics to calculate quantities such as velocity, acceleration, force, and energy. Integration by substitution and partial fractions are used to solve more complex problems in physics, such as modeling the motion of objects under the influence of gravity or electromagnetic fields.
- Engineering: Engineers use integration to analyze systems and solve design problems in a variety of fields, including mechanical, electrical, and civil engineering. Integration by substitution and partial fractions are used to solve differential equations, which are commonly used to model physical systems.
- Economics: Integration is used in economics to analyze functions that represent supply and demand, cost functions, and production functions. Integration by substitution and partial fractions are used to calculate areas under curves that represent economic quantities, such as consumer surplus or producer surplus.
- Computer Science: Integration is used in computer science to solve problems related to numerical analysis, optimization, and data analysis. Integration by substitution and partial fractions are used in algorithms that approximate integrals and in machine learning algorithms that optimize models based on data.
In general, integration by substitution and partial fractions are used in any field that applies calculus to solve complex problems. These techniques are important tools for solving a wide range of problems in science, engineering, and other fields.
How is Required Integration by the methods of substitution and partial fractions
Integration by substitution and partial fractions are techniques that are used to simplify and evaluate integrals. The steps involved in using these techniques can be summarized as follows:
Integration by Substitution:
- Identify the inner function g(x) and its derivative g'(x) in the integrand.
- Substitute a new variable u for g(x), such that u = g(x).
- Replace all instances of g(x) and g'(x) in the integrand with u and du/dx, respectively.
- Evaluate the resulting integral in terms of the new variable u using basic integration rules.
- Substitute the original function g(x) back into the solution to obtain the final result.
Partial Fractions:
- Factor the denominator of the rational function into irreducible factors.
- Express the rational function as a sum of simpler fractions with denominators equal to each of the irreducible factors.
- Solve for the constants in the partial fraction decomposition by equating the numerators of both sides of the equation.
- Evaluate the resulting integrals using basic integration rules.
- Combine the results of each integral to obtain the final solution.
In general, the process of integration by substitution involves finding a suitable substitution that transforms the integrand into a simpler form that can be easily integrated. The process of partial fractions involves decomposing a rational function into simpler fractions that can be more easily integrated. By combining these techniques, you can simplify more complex integrals and make them easier to solve.
Case Study on Integration by the methods of substitution and partial fractions
Let’s consider the following integral as a case study:
∫(x^3 + 2x + 1)/(x^2 + 1)^2 dx
This integral involves a rational function with a complex polynomial in the numerator and a quadratic polynomial in the denominator raised to the power of 2. We can use the technique of partial fractions to simplify this integral and make it easier to integrate.
Step 1: Factor the denominator of the rational function into irreducible factors.
(x^2 + 1)^2 = (x^2 + 1)(x^2 + 1)
Step 2: Express the rational function as a sum of simpler fractions with denominators equal to each of the irreducible factors.
(x^3 + 2x + 1)/[(x^2 + 1)^2] = A/(x^2 + 1) + B/(x^2 + 1)^2
Step 3: Solve for the constants in the partial fraction decomposition by equating the numerators of both sides of the equation.
(x^3 + 2x + 1) = A(x^2 + 1) + B(x^2 + 1)^2
Expanding the right-hand side and equating coefficients of like terms, we get:
x^3 + 2x + 1 = A(x^2 + 1) + B(x^4 + 2x^2 + 1)
Simplifying and equating coefficients, we get:
A + B = 0 2B = 2 A = 1
Solving for A and B, we get:
A = 1, B = -1/2
Step 4: Evaluate the resulting integrals using basic integration rules.
∫(x^3 + 2x + 1)/(x^2 + 1)^2 dx = ∫[1/(x^2 + 1) – 1/2(x^2 + 1)^2] dx
To evaluate the integral, we can use the substitution u = x^2 + 1. This gives us:
∫[1/u – 1/2u^2] du/2x
= (1/2)ln|x^2 + 1| + (1/4)(x^2 + 1)^-1 + C
where C is the constant of integration.
In this case study, we used the technique of partial fractions to simplify a complex rational function and then evaluated the resulting integrals using the substitution method. By breaking down the integral into simpler parts, we were able to solve a more complex problem using basic integration rules.
White paper on Integration by the methods of substitution and partial fractions
Integration by the methods of substitution and partial fractions are two important techniques in calculus that are used to evaluate complex integrals. In this white paper, we will discuss the concepts and applications of these techniques in detail.
Integration by Substitution:
Integration by substitution, also known as the u-substitution method, is a technique used to evaluate integrals by replacing a function in the integrand with a new variable. The substitution simplifies the integrand, making it easier to evaluate. The steps involved in this method are:
- Identify the inner function g(x) and its derivative g'(x) in the integrand.
- Substitute a new variable u for g(x), such that u = g(x).
- Replace all instances of g(x) and g'(x) in the integrand with u and du/dx, respectively.
- Evaluate the resulting integral in terms of the new variable u using basic integration rules.
- Substitute the original function g(x) back into the solution to obtain the final result.
For example, consider the integral:
∫(3x^2 + 2x)^3 dx
Using the u-substitution method, we can set u = 3x^2 + 2x, and then find du/dx = 6x + 2. Substituting these expressions, we get:
∫u^3 (1/6)(du/dx) dx
= (1/6)∫u^3 du
= (1/24)(3x^2 + 2x)^4 + C
where C is the constant of integration.
Integration by Partial Fractions:
Integration by partial fractions is a technique used to integrate a rational function by breaking it down into simpler fractions. The steps involved in this method are:
- Factor the denominator of the rational function into irreducible factors.
- Express the rational function as a sum of simpler fractions with denominators equal to each of the irreducible factors.
- Solve for the constants in the partial fraction decomposition by equating the numerators of both sides of the equation.
- Evaluate the resulting integrals using basic integration rules.
- Combine the results of each integral to obtain the final solution.
For example, consider the integral:
∫(x^2 + 3)/(x^3 + x) dx
Using the partial fractions method, we can factor the denominator as x(x^2 + 1) and then write the rational function as:
(x^2 + 3)/(x^3 + x) = A/x + B/(x^2 + 1)
Solving for the constants A and B, we get:
A = 1/3, B = 2/3
Substituting these values, we get:
∫(1/3) dx/x + ∫(2/3) dx/(x^2 + 1)
= (1/3)ln|x| + (2/3)arctan(x) + C
where C is the constant of integration.
Applications of Integration by Substitution and Partial Fractions:
Integration by substitution and partial fractions have numerous applications in science, engineering, and economics. These techniques are used to solve complex problems in fields such as physics, chemistry, and biology. They are also used in signal processing, control systems, and financial modeling.
For example, in physics, integration by substitution and partial fractions are used to calculate the work done by a force, the amount of energy stored in a system, and the trajectory of an object. In economics, these techniques are used to model the behavior of consumers, firms, and markets.
Conclusion:
In conclusion, integration by substitution and partial fractions are important techniques in calculus that are used to evaluate complex integrals. The u-substitution method involves replacing a function in the integrand with a new variable, simplifying the integrand and making it easier to evaluate. The partial fractions method involves breaking down a rational function into simpler fractions, solving for constants, and integrating each term separately. These techniques have numerous applications in science, engineering, economics, and other fields, allowing us to model and solve complex problems. Understanding and mastering these methods can greatly enhance one’s ability to solve challenging integrals and tackle real-world problems.