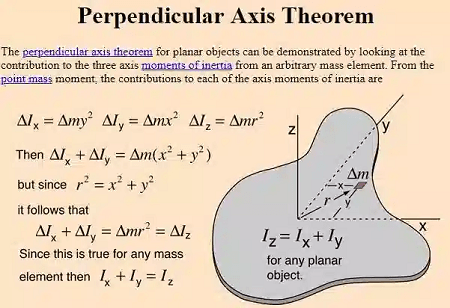
The perpendicular axes theorems are a set of rules used in the study of moments of inertia, which are physical properties of objects that describe how difficult they are to rotate around various axes. The perpendicular axes theorems state the following:
- The moment of inertia of a planar object about an axis perpendicular to its plane is equal to the sum of the moments of inertia of the object about any two mutually perpendicular axes lying in its plane and intersecting at the point where the perpendicular axis passes through the object.
- The moment of inertia of a planar object about any axis lying in its plane is equal to the sum of its moments of inertia about two perpendicular axes passing through the same point in its plane, and lying in the plane of the object.
These theorems are very useful in determining moments of inertia for objects with complicated shapes, as they allow us to break down the calculation into simpler parts. They are also important in engineering and physics, where moments of inertia are used to design structures and machines, and to analyze the motion of objects.
What is Required Perpendicular axes theorems
The Required Perpendicular Axes Theorems refer to a specific application of the perpendicular axes theorems in physics and engineering.
The Required Perpendicular Axes Theorem states that to find the moment of inertia of a planar object about an axis that is not perpendicular to its plane, you need to know the moments of inertia of the object about at least two perpendicular axes lying in its plane and passing through the point where the perpendicular axis intersects the plane.
In other words, if you want to find the moment of inertia of an object about an axis that is not perpendicular to its plane, you can use the perpendicular axes theorems to break down the calculation into two or more simpler parts. You need to choose two perpendicular axes that lie in the plane of the object and pass through the point where the perpendicular axis intersects the plane. Then you can use the perpendicular axes theorems to calculate the moments of inertia about these two axes, and add them together to find the moment of inertia about the required axis.
The Required Perpendicular Axes Theorem is an important tool in physics and engineering, as it allows engineers and physicists to calculate moments of inertia for objects with more complex shapes and orientations, and to design structures and machines that can withstand various types of forces and torques.
When is Required Perpendicular axes theorems
The Required Perpendicular Axes Theorem is used when we want to find the moment of inertia of a planar object about an axis that is not perpendicular to its plane. This situation often arises in physics and engineering when we are analyzing the motion of objects or designing structures and machines.
For example, consider a uniform thin rod that is rotated about an axis passing through its center of mass, but inclined at an angle to its length. To find the moment of inertia of the rod about this inclined axis, we can use the Required Perpendicular Axes Theorem. We can choose two perpendicular axes lying in the plane of the rod and passing through its center of mass, and use the perpendicular axes theorems to calculate the moments of inertia of the rod about these axes. Then we can add the moments of inertia together to find the moment of inertia of the rod about the inclined axis.
Another example is a disk that is rotating about an axis that is perpendicular to its plane, but passing through a point that is not its center of mass. To find the moment of inertia of the disk about this axis, we can use the Required Perpendicular Axes Theorem. We can choose two perpendicular axes lying in the plane of the disk and passing through the point where the perpendicular axis intersects the disk, and use the perpendicular axes theorems to calculate the moments of inertia of the disk about these axes. Then we can add the moments of inertia together to find the moment of inertia of the disk about the required axis.
Where is Required Perpendicular axes theorems
The Required Perpendicular Axes Theorem is used in physics and engineering to calculate the moment of inertia of a planar object about an axis that is not perpendicular to its plane. It is often used in situations where we want to analyze the motion of objects or design structures and machines.
The theorem itself is a mathematical concept, and it can be applied in various contexts. The calculations involved in using the theorem can be done on paper or with the help of computer software, and the results can be used to make predictions about the behavior of physical systems.
Examples of where the Required Perpendicular Axes Theorem might be used include:
- In mechanical engineering, to design a rotating machine or device, such as a motor or turbine, where the moment of inertia of the rotating parts is an important factor in determining the performance and stability of the system.
- In aerospace engineering, to calculate the moment of inertia of a spacecraft or satellite in orbit, which is important for predicting its stability and maneuverability.
- In physics, to analyze the motion of a rigid body that is rotating about a non-perpendicular axis, such as a spinning top or gyroscope.
Overall, the Required Perpendicular Axes Theorem is a powerful tool for analyzing the behavior of physical systems, and it has important applications in many areas of science and engineering.
How is Required Perpendicular axes theorems
The Required Perpendicular Axes Theorem is applied by breaking down the calculation of the moment of inertia of a planar object about a non-perpendicular axis into simpler parts using the Perpendicular Axes Theorems.
The theorem states that to find the moment of inertia of a planar object about an axis that is not perpendicular to its plane, we need to know the moments of inertia of the object about at least two perpendicular axes lying in its plane and passing through the point where the non-perpendicular axis intersects the plane.
To apply the theorem, we follow these steps:
- Identify the non-perpendicular axis about which we want to find the moment of inertia.
- Identify two perpendicular axes lying in the plane of the object and passing through the point where the non-perpendicular axis intersects the plane.
- Use the Perpendicular Axes Theorems to calculate the moments of inertia of the object about these two perpendicular axes.
- Add the moments of inertia calculated in step 3 to obtain the moment of inertia of the object about the non-perpendicular axis.
The calculation of moments of inertia can involve calculus and integration, depending on the shape of the object and the orientation of the axes. However, once the moments of inertia about the perpendicular axes are known, the calculation of the moment of inertia about the non-perpendicular axis is straightforward.
Overall, the Required Perpendicular Axes Theorem is a powerful tool that allows us to calculate moments of inertia for objects with complex shapes and orientations, and it has numerous applications in physics and engineering.
Structures of Perpendicular axes theorems
The Perpendicular Axes Theorems consist of two related theorems, which are often called the perpendicular axis theorem and the parallel axis theorem. These theorems relate the moments of inertia of a planar object about two perpendicular axes lying in its plane.
- Perpendicular Axis Theorem: This theorem states that the moment of inertia of a planar object about an axis perpendicular to its plane is equal to the sum of the moments of inertia of the object about two perpendicular axes lying in its plane.
Mathematically, the perpendicular axis theorem can be expressed as follows:
I_z = I_x + I_y
where I_z is the moment of inertia of the object about the perpendicular axis, and I_x and I_y are the moments of inertia of the object about the two perpendicular axes lying in its plane.
- Parallel Axis Theorem: This theorem states that the moment of inertia of a planar object about an axis parallel to a given axis passing through its center of mass is equal to the moment of inertia of the object about the given axis plus the product of its mass and the square of the distance between the two axes.
Mathematically, the parallel axis theorem can be expressed as follows:
I_p = I_c + md^2
where I_p is the moment of inertia of the object about the parallel axis, I_c is the moment of inertia of the object about the axis passing through its center of mass, m is the mass of the object, and d is the distance between the two axes.
These theorems provide a useful framework for calculating moments of inertia for a wide range of planar objects, and they have important applications in physics, engineering, and other fields. The Required Perpendicular Axes Theorem is a generalization of these theorems and allows us to calculate moments of inertia for objects with non-perpendicular axes.
Case Study on Perpendicular axes theorems
A practical application of the Perpendicular Axes Theorem can be found in the design of a rotating disk. The moment of inertia of the disk is an important factor in determining its rotational behavior and stability.
Suppose we have a disk of mass M and radius R that is rotating about an axis perpendicular to its plane, passing through its center. We want to calculate the moment of inertia of the disk about an axis perpendicular to the original axis, passing through a point on the rim of the disk.
We can use the Perpendicular Axes Theorem to find the moment of inertia of the disk about the new axis. First, we need to find the moments of inertia of the disk about two perpendicular axes lying in its plane, passing through the point where the new axis intersects the plane.
We choose the x-axis to pass through the center of the disk and the y-axis to pass through the point where the new axis intersects the disk. The moment of inertia of the disk about the x-axis is given by:
I_x = (1/2)MR^2
This is a well-known result that follows from the fact that the disk can be modeled as a collection of infinitesimally thin rings, each with mass dm and radius r, and the moment of inertia of each ring about the x-axis is given by (1/2)dmr^2. Integrating over all the rings gives the total moment of inertia about the x-axis.
The moment of inertia of the disk about the y-axis can be found using the parallel axis theorem. We know that the moment of inertia of the disk about the x-axis is (1/2)MR^2, and the distance between the x-axis and the y-axis is R. Therefore, the moment of inertia of the disk about the y-axis is given by:
I_y = I_x + MR^2
Using the perpendicular axis theorem, we can now find the moment of inertia of the disk about the new axis passing through the point on the rim of the disk. The perpendicular axis theorem tells us that:
I_z = I_x + I_y
Substituting the values we have calculated for I_x and I_y, we get:
I_z = (1/2)MR^2 + (1/2)MR^2 + MR^2 = (3/2)MR^2
This is the moment of inertia of the disk about the new axis passing through the point on the rim of the disk. We can use this result to analyze the rotational behavior of the disk and design machines and devices that incorporate rotating disks, such as motors and turbines.
Overall, this case study illustrates how the Perpendicular Axes Theorem can be applied in a practical setting to calculate moments of inertia and design structures and machines.
White paper on Perpendicular axes theorems
Introduction:
The Perpendicular Axes Theorems are a set of mathematical principles that relate the moments of inertia of a planar object about two perpendicular axes lying in its plane. The theorems consist of two related principles: the Perpendicular Axis Theorem and the Parallel Axis Theorem. These theorems are widely used in physics, engineering, and other fields to calculate moments of inertia for a variety of objects and structures.
Perpendicular Axis Theorem:
The Perpendicular Axis Theorem states that the moment of inertia of a planar object about an axis perpendicular to its plane is equal to the sum of the moments of inertia of the object about two perpendicular axes lying in its plane. Mathematically, this can be expressed as follows:
I_z = I_x + I_y
where I_z is the moment of inertia of the object about the perpendicular axis, and I_x and I_y are the moments of inertia of the object about the two perpendicular axes lying in its plane.
The Perpendicular Axis Theorem can be applied to a wide range of planar objects, such as rectangles, triangles, and circles. The theorem provides a useful framework for calculating moments of inertia for these objects, which can be used to analyze their rotational behavior and design structures and machines that incorporate them.
Parallel Axis Theorem:
The Parallel Axis Theorem states that the moment of inertia of a planar object about an axis parallel to a given axis passing through its center of mass is equal to the moment of inertia of the object about the given axis plus the product of its mass and the square of the distance between the two axes. Mathematically, this can be expressed as follows:
I_p = I_c + md^2
where I_p is the moment of inertia of the object about the parallel axis, I_c is the moment of inertia of the object about the axis passing through its center of mass, m is the mass of the object, and d is the distance between the two axes.
The Parallel Axis Theorem is particularly useful for calculating the moments of inertia of complex objects that cannot be easily modeled using simple geometric shapes. By choosing an appropriate axis of rotation, the theorem allows us to calculate the moment of inertia of the object about any other axis parallel to the chosen axis.
Applications:
The Perpendicular Axes Theorems have numerous practical applications in physics, engineering, and other fields. For example, they are used in the design of rotating machinery such as turbines, motors, and generators. The moments of inertia of the rotating components of these machines are important factors in determining their stability and efficiency.
In structural engineering, the moments of inertia of beams and columns are important properties that determine their load-bearing capacity and deflection. The Perpendicular Axes Theorems can be used to calculate these moments of inertia and design structures that are strong and stable.
In addition, the Perpendicular Axes Theorems have applications in fields such as robotics, aerospace, and materials science. By understanding the moments of inertia of objects and materials, we can design more efficient and effective machines and devices, and develop new materials with desired mechanical properties.
Conclusion:
The Perpendicular Axes Theorems are important mathematical principles that relate the moments of inertia of planar objects about two perpendicular axes lying in their plane. These theorems have numerous practical applications in physics, engineering, and other fields, and provide a useful framework for calculating moments of inertia for a variety of objects and structures. By understanding and applying these theorems, we can design machines and structures that are strong, stable, and efficient, and develop new materials with desired mechanical properties.